PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
Number of solution(s) of the equation logcosθ(x+2)=2+3log(x+2)sin(5θ2) is
0
1
2
3
The correct Answer is:C
Step by step video, text & image solution for PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and angleAPB= theta . On the basis of above information answer the questions . Number of solution(s) of the equation log_(cos theta )(x+2)=2+3 log_((x+2)) sin""((5 theta )/(2)) is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise For Session 115videos
TRIGONOMETRIC EQUATIONS AND INEQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC EQUATIONS AND INEQUATIONSEXERCISE - Exercise For Session 28videos
TRIGONOMETRIC EQUATIONS AND INEQUATIONS
- BOOK - ARIHANT MATHSCHAPTER - THREE DIMENSIONAL COORDINATE SYSTEMEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)44videos
THREE DIMENSIONAL COORDINATE SYSTEM
- BOOK - ARIHANT MATHSCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)19videos
TRIGONOMETRIC FUNCTIONS AND IDENTITIES
Similar Questions
Consider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
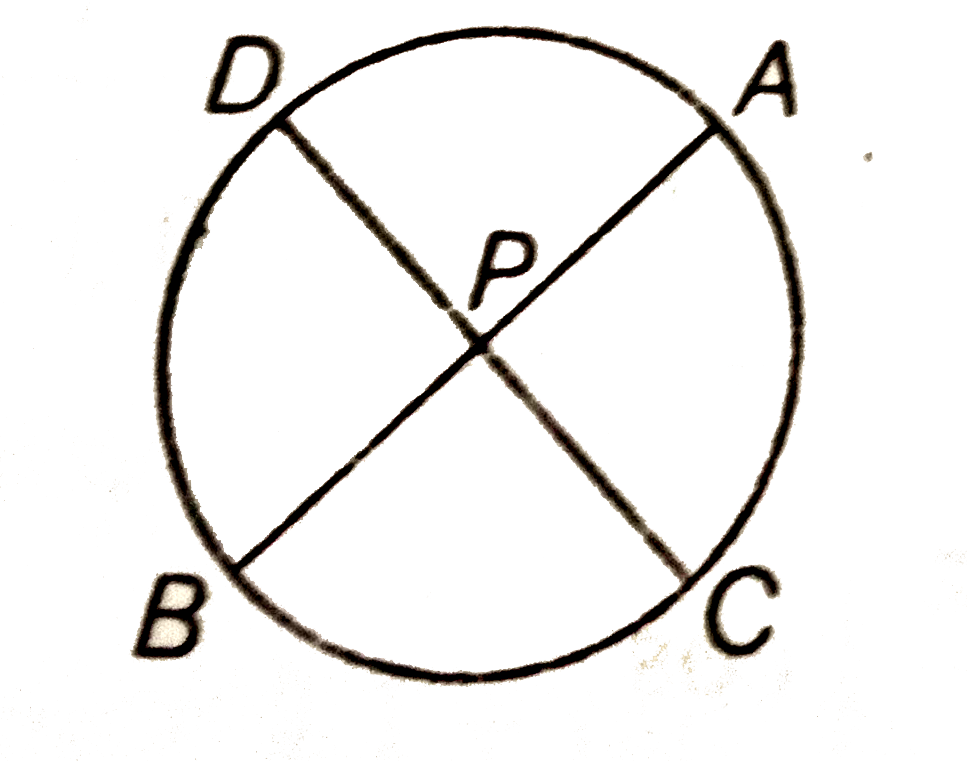
On the basis of above information , answer the questions
If logPAx=2,logPBx=3,logxPC=4, then logPD x is equal toView SolutionTwo tangents are drawn from point P(h,0),h<0 to the circle x2+y2−4x=0, which touches the circleat A&B and rhombus PACB is then completed.On the basis of above information, answer the following questions : If point P be such that C lies on the circle, then h is equal to
View Solution
Knowledge Check
- Question 1 - Select One
PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
Area of ΔAPB isA25√32B25√3C75√32D75√34 - Question 2 - Select One
PA and PB are two tangents drawn from point P to circle of radius 5 . A line is drawn from point P which cuts at C and D such that PC=5 and PD=15 and ∠APB=θ.
On the basis of above information answer the questions .
Value of sin2θ+cos4θ+sin5θ+tan7θ+cot8θ is equal toA4√3−12B4√3−12√3C4√3+12√3D4√3+12 - Question 3 - Select One
If 3sin2x−7sinx+2=0,x∈[0π2]andfn(θ)=sinnθ+cosnθ .
On the basis of above information , answer the questions
The value of sin5x+sin4x1+2cos3x isA3+2√29B3+4√29C4√2−29D4√2−39
Consider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
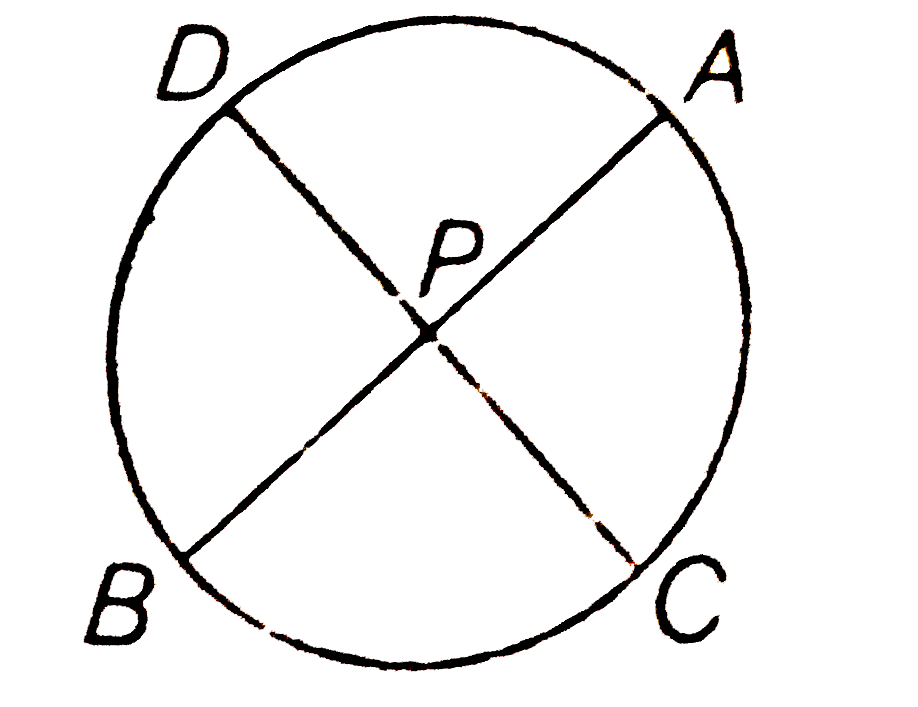
On the basis of above information , answer the questions
If PA=|cosθ+sinθ|andPB=|cosθ−sinθ|, then maximum value of (PC)(PD) , is equal toView SolutionConsider a circle , in which a point P is lying inside the circle such that (PA)(PB)=(PC)(PD) ( as shown in figure ) .
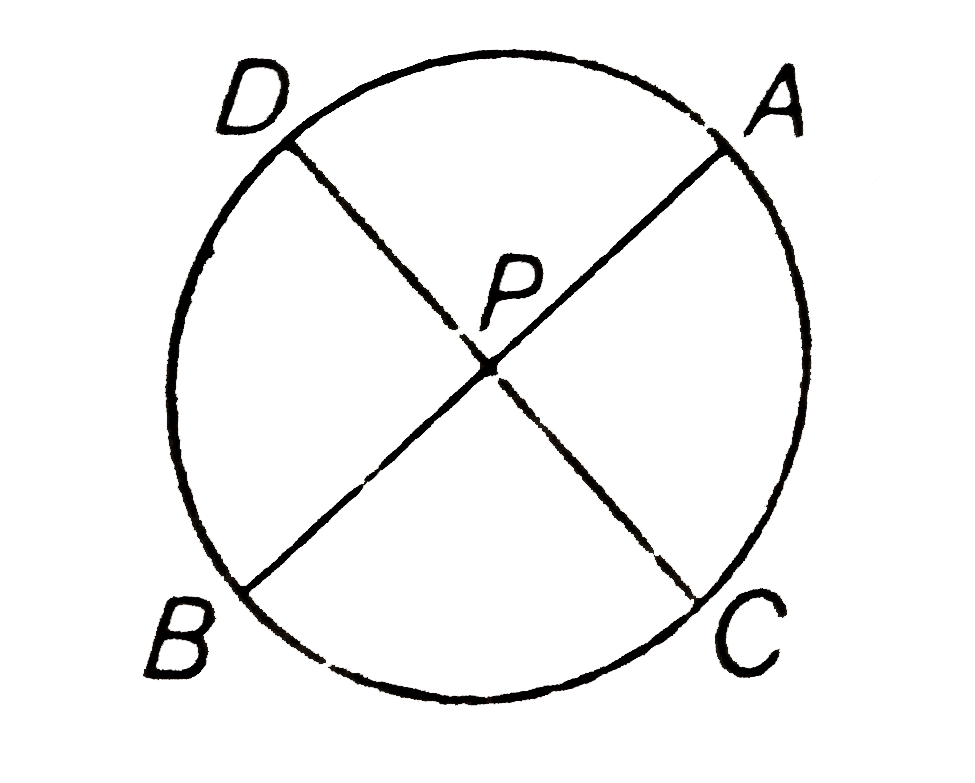
On the basis of above information , answer the questions
Let PA=4 , PB=3 cm and CD is diameter of the circle having the length 8. If PC>CD , then PCPD is equal toView SolutionThe number of solution of the equation sin2θ−2cosθ+4sinθ=4∈[0,5π] is equal to 3 (b) 4 (c) 5 (d) 6
View SolutionNumber of solutions of 5cos2θ−3sin2θ+6sinθcosθ=7 in the interval [0,2π) is:
View SolutionThe method of eliminating 'θ' from two given equations involving trigonometrical functions of 'θ'. By using given equations involving 'θ' and trigonometrical identities, we shall obtain an equation not involving 'θ'.
On the basis of above information answer the following questions.
After eliminating 'θ' from equations xcosθa+ysinθb=1 and xsinθ−ycosθ=√(a2sin2θ+b2cos2θ), we getView Solution

