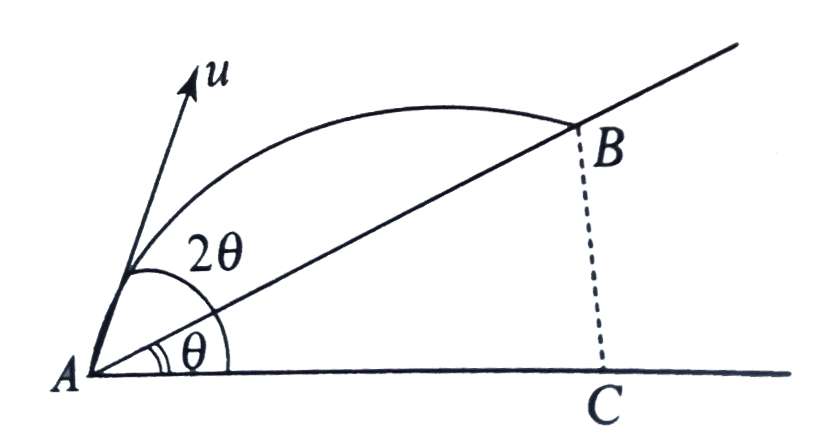
A particle is projected from point A on plane AB, so that $A B = \frac{2 u^{2} tan θ}{g \sqrt{3}}$ in the figure as shown. If u is the velocity of projection, find angle $θ$ .

A

$30^{\circ}$

B

$60^{\circ}$

C

$90^{\circ}$

D

$45^{\circ}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Time of flight $t = \frac{2 u sin (2 θ - θ)}{g cos θ} = \frac{2 u}{g} tan θ$
$A B = \frac{2 u^{2} tan θ}{g \sqrt{3}} = \frac{u}{\sqrt{3}} t$ ..(i)
$u_{H} = u cos 2 θ$
$A C = u_{H} . t = u cos 2 θ t$
$A B = \frac{A C}{cos θ} = \frac{u cos 2 θ t}{cos θ}$ …(ii)
Compare equations (i) and (ii)
$\frac{cos 2 θ}{cos θ} = \frac{1}{\sqrt{3}}$ or $θ = 30^{\circ}$

Updated on:21/07/2023

|

Class 12 PHYSICS QUESTION BANK

Similar Questions

Knowledge Check

Figure shows that particle A is projected from point P with velocity u along the plane and simultaneously another particle B with velocity v at an angle $α$ with vertical. The particles collide at point Q on the plane. Then

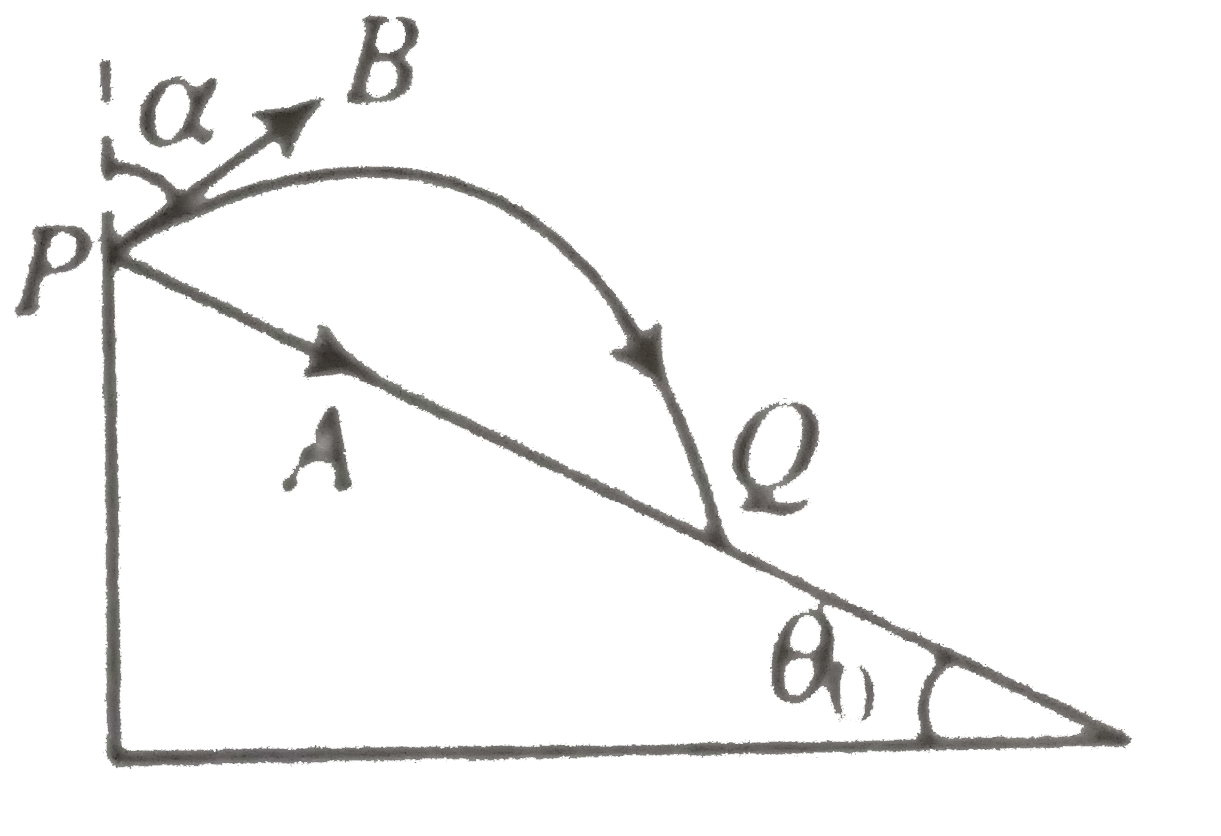A $v sin (α - θ_{0}) = u$
B $v cos (α - θ_{0}) = u$
C $v = u$
DNone of these
A particle is projected at point A from an inclination plane with inclination angle $θ$ as shown in figure. The magnitude of projection velocity is $\to u$ and its direction is perpendicular to the plane. After some time it passes from point B which is in the same horizontal level of A, with velocity $\to v$ . Then the angle between $\to u and \to v$ will be

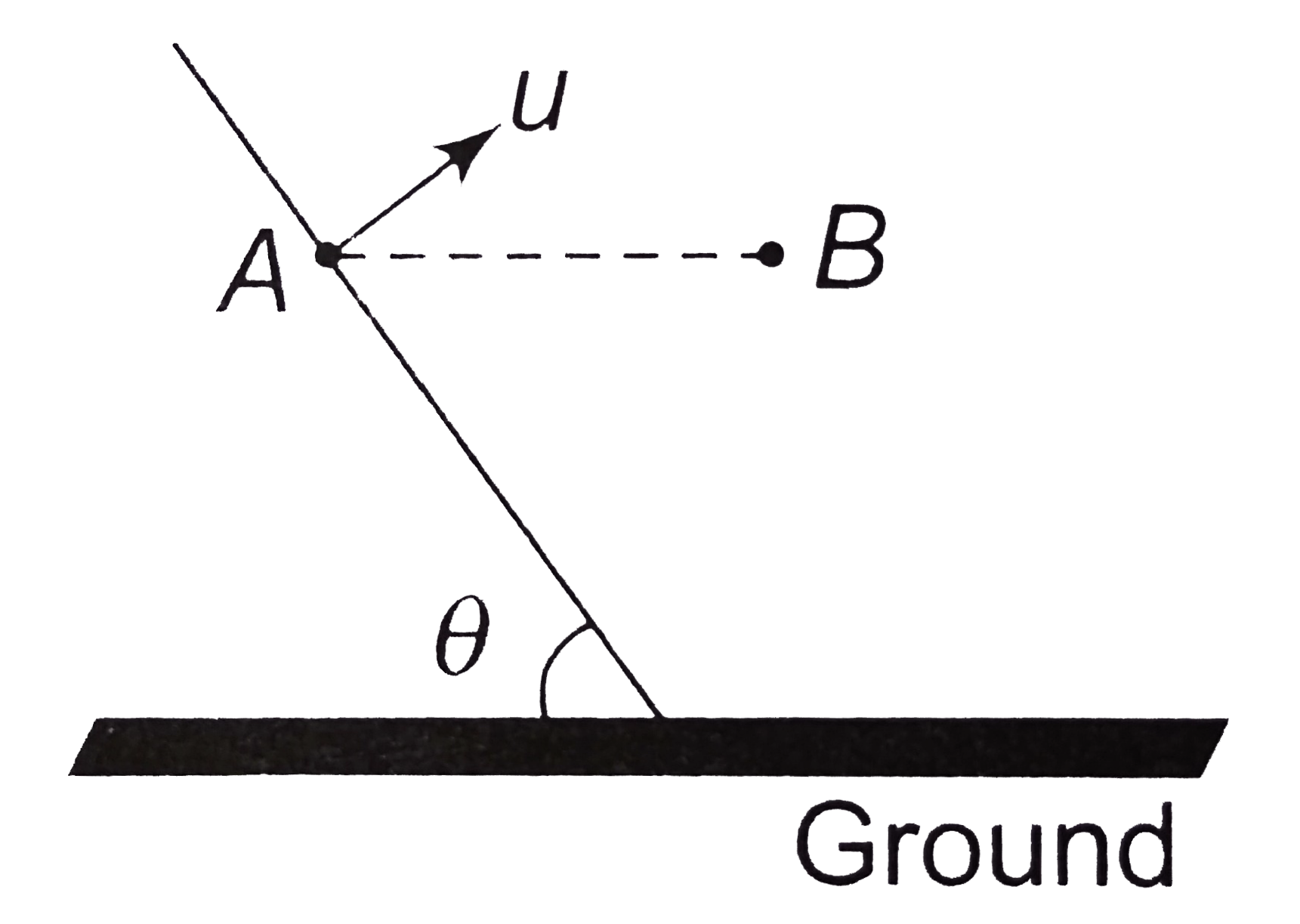A $θ$
B $2 θ$
C $π - 2 θ$
D $90 + θ$
A particle is projected with initial speed u at an angle $θ$ above the horizontal . Let A be the point of projection , B the point where velocity makes an angle $θ / 2$ above the horizontal and C the highest point of the trajectory .
Radius of curvature of the trajectory at point C is
A $\frac{u^{2} {cos}^{3} θ}{g^{2}}$
B $\frac{2 u^{2} {cos}^{2} θ}{g}$
C $\frac{u^{2} {cos}^{2} θ}{g}$
D $\frac{u^{2} {cos}^{3} θ}{g}$

Recommended Questions

Home
Ask Doubt
Profile