There different objective of masses and are allowed to fall from rest and from the same point O along three difference frictionless path .The speeds of three objects on reaching the ground will be
The correct Answer is:b
To solve the problem of determining the speeds of the three objects m1,m2, and m3 when they reach the ground after falling from the same height h along different frictionless paths, we can use the principle of conservation of energy.
1. Identify the Initial and Final States:
- The objects are released from rest at a height h.
- Initial velocity u=0 for all objects.
- Final velocity v will be determined when they reach the ground.
2. Apply the Conservation of Mechanical Energy:
- The potential energy at the height h will convert into kinetic energy when the objects reach the ground.
- The potential energy (PE) at height h is given by:
PE=mgh
- The kinetic energy (KE) when the object reaches the ground is given by:
KE=12mv2
3. Set Up the Energy Conservation Equation:
- At the top (height h), the total energy is purely potential:
mgh=12mv2
4. Simplify the Equation:
- Since mass m appears on both sides of the equation, we can cancel it out (assuming m≠0):
gh=12v2
5. Solve for Final Velocity v:
- Rearranging the equation gives:
v2=2gh
- Taking the square root of both sides:
v=√2gh
6. Conclusion:
- The final speed v of each object when they reach the ground is:
v=√2gh
- This result shows that the final speed is independent of the mass of the objects and depends only on the height h and the acceleration due to gravity g.
7. Ratio of Speeds:
- Since all three objects m1,m2, and m3 have the same final speed:
v1:v2:v3=1:1:1
Final Answer:
The speeds of the three objects when they reach the ground will be in the ratio 1:1:1.
---
- The objects are released from rest at a height h.
- Initial velocity u=0 for all objects.
- Final velocity v will be determined when they reach the ground.
- The potential energy at the height h will convert into kinetic energy when the objects reach the ground.
- The potential energy (PE) at height h is given by:
PE=mgh
- The kinetic energy (KE) when the object reaches the ground is given by:
KE=12mv2
- At the top (height h), the total energy is purely potential:
mgh=12mv2
- Since mass m appears on both sides of the equation, we can cancel it out (assuming m≠0):
gh=12v2
- Rearranging the equation gives:
v2=2gh
- Taking the square root of both sides:
v=√2gh
- The final speed v of each object when they reach the ground is:
v=√2gh
- This result shows that the final speed is independent of the mass of the objects and depends only on the height h and the acceleration due to gravity g.
- Since all three objects m1,m2, and m3 have the same final speed:
v1:v2:v3=1:1:1
The speeds of the three objects when they reach the ground will be in the ratio 1:1:1.
Topper's Solved these Questions
- MOCK TESTBook:A2ZChapter:MOCK TESTExercise:General Kinematics
Explore 30 Videos
- MOCK TESTBook:A2ZChapter:MOCK TESTExercise:Motion With Constant Acceleration
Explore 15 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBook:A2ZChapter:KINETIC THEORY OF GASES AND THERMODYNAMICSExercise:Chapter Test
Explore 29 Videos
- MOTION IN TWO DIMENSIONBook:A2ZChapter:MOTION IN TWO DIMENSIONExercise:Chapter Test
Explore 29 Videos
Similar Questions
Two bodies of masses M and m are allowed to fall from the same height . If air resistance for each body be same , will the two bodies reach the ground simultaneously ?
View SolutionTwo bodies of masses M and m are allowed to fall from the same height. If air resistance for each body be same, will the two bodies reach the ground simultaneously ?
View Solution
Knowledge Check
An object of mass m is allowed to fall from rest along a rough inclined plane. The speed of the object on reaching the bottom of the plane is proportional to:
Am0BmCm2Dm−1An electron of mass m and charge e is accelerated from rest through a potential difference V in vacuum. The final speed of the electron will be
AV√e/mB√eV/mC√2eV/mD2eV/mAssertion: Two bodies of masses M and m(M>m) are allowed to fall from the same height if the air resistance for each be the same then both the bodies will reach the earth simultaneously.
Reason: For same air resistance, acceleration of both the bodies will be same.AStatement-1 is True , Statement-2 is True, Statement-2 is a correct explanation for Statement-1.BStatement-1 is True , Statement-2 is True, Statement-2 is NOT a correct explanation for Statement-1.CStatement-1 is True, Statement-2 is FalseDStatement-1 is False, Statement-2 is True.
A block of mass m is dropped from height h above the ground. Find out the speed of the block when it reaches the ground.
View SolutionThree masses m1,m2andm3 are attached to a string -pulley system as shown . All the three masses are held at rest and then released If m3 remains at rest , then
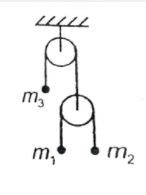
View Solutioni) If the net force acting on a body is zero, will it remain necessarily at rest?
(ii) Two bodies of mass M and m are allowed to fall from the same height. If air resistance for each body be same, will the two bodies reach the ground simultaneously?
View SolutionA sphere of mass m held at a height 2R between a wedge of same m and a rigid wall, is released from, Assuming that all the surfaces are frictionless. Find the speed of the bodies when the sphere hits the ground.
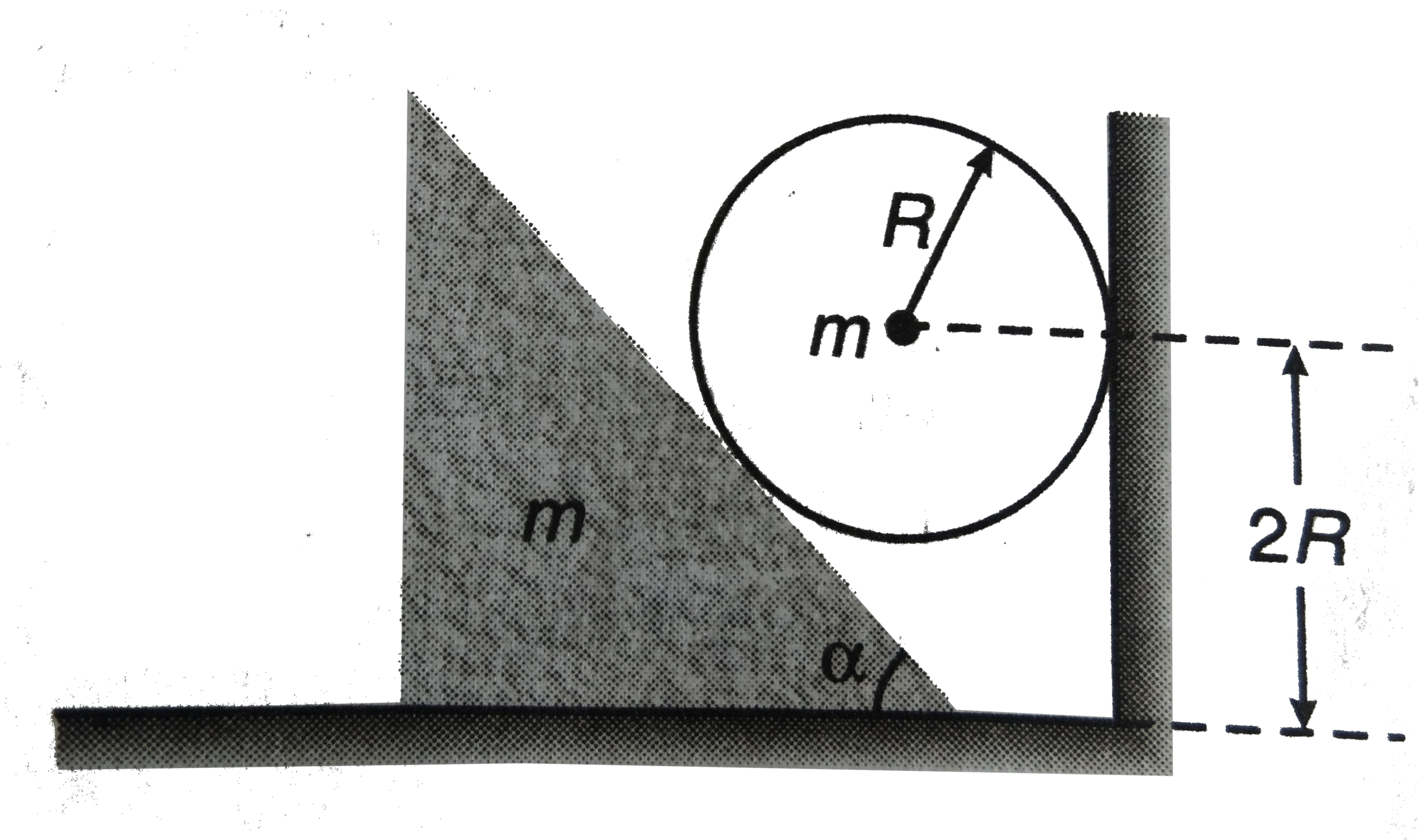 .
.
View SolutionThree block of masses m1,m2 and m3 kg are placed in contact with each other on a frictionless table. A force F is applied on the heaviest mass m1, the acceleration of m2 will be
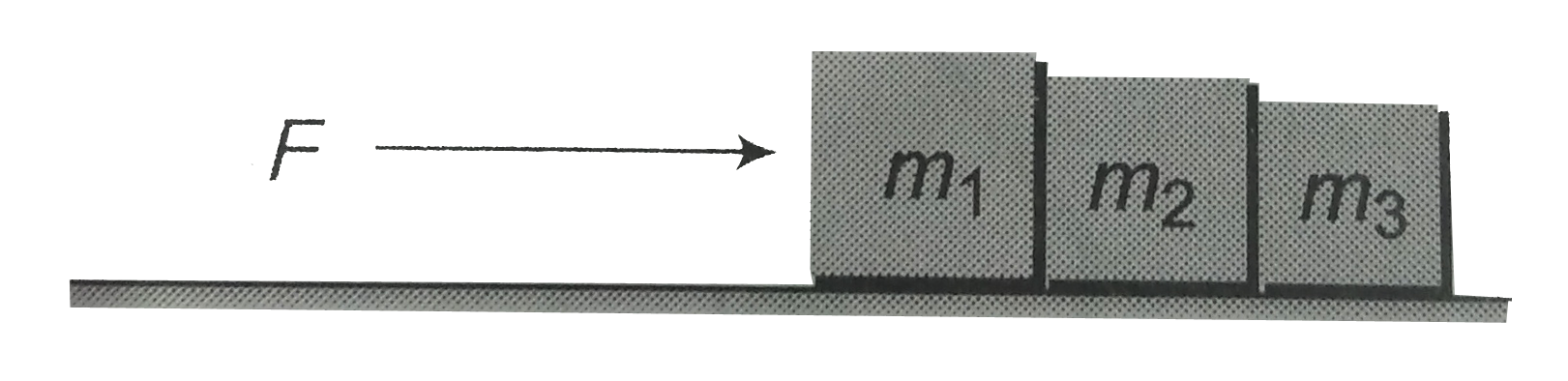
View Solution
