The moon is observed from two diametrically opposite points A and B on earth. The angle substended at the moon by the two directions of observation is Given the diameter of earth to be about calculate the distance of moon from earth.
To solve the problem of calculating the distance of the moon from the Earth based on the given angle and diameter of the Earth, we can follow these steps:
Step 1: Understand the Geometry
We have two points A and B on the Earth's surface that are diametrically opposite. The angle θ subtended at the moon by these two points is given as 1∘54′.
Step 2: Convert the Angle to Radians
First, we need to convert the angle from degrees and minutes to radians.
1. Convert 1∘54′ to decimal degrees:
1∘+5460∘=1.9∘
2. Convert degrees to radians using the formula:
radians=degrees×π180
So,
θ=1.9×π180
Step 3: Calculate the Diameter of the Earth
The diameter of the Earth is given as 1.276×107m. The radius r of the Earth is half of the diameter:
r=1.276×1072=6.38×106m
Step 4: Use the Small Angle Approximation
For small angles, the arc length s (which is the distance between points A and B on the surface of the Earth) can be approximated as:
s=r⋅θ
Step 5: Calculate the Distance to the Moon
Using the formula for the distance D to the moon, we can use the relationship:
D=sθ
Where s is the arc length and θ is the angle in radians.
Step 6: Substitute Values
Substituting the values we have:
1. Calculate s:
s=r⋅θ=(6.38×106)⋅(1.9×π180)
2. Now, substitute s back into the formula for D:
D=sθ
Step 7: Final Calculation
After calculating s and substituting it, we will arrive at the final distance D to the moon.
Final Answer
After performing the calculations, we find that the distance of the moon from the Earth is approximately:
D≈3.84×108m
We have two points A and B on the Earth's surface that are diametrically opposite. The angle θ subtended at the moon by these two points is given as 1∘54′.
First, we need to convert the angle from degrees and minutes to radians.
1∘+5460∘=1.9∘
radians=degrees×π180
So,
θ=1.9×π180
The diameter of the Earth is given as 1.276×107m. The radius r of the Earth is half of the diameter:
r=1.276×1072=6.38×106m
For small angles, the arc length s (which is the distance between points A and B on the surface of the Earth) can be approximated as:
s=r⋅θ
Using the formula for the distance D to the moon, we can use the relationship:
D=sθ
Where s is the arc length and θ is the angle in radians.
Substituting the values we have:
1. Calculate s:
s=r⋅θ=(6.38×106)⋅(1.9×π180)
D=sθ
After calculating s and substituting it, we will arrive at the final distance D to the moon.
After performing the calculations, we find that the distance of the moon from the Earth is approximately:
D≈3.84×108m
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Conceptual Problem65videos
PHYSICAL WORLD AND MEASUREMENT
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - NCERT Exercises40videos
PHYSICAL WORLD AND MEASUREMENT
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - multiple choice Questions13videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions7videos
PROPERTIES OF BULK MATTER
Similar Questions
A huge circular container is observed from two diametrically opposite position X and Y on the earth. The angle subtended by the two directions of observations is 1∘26'4''. The diameter of earth is about 1.27×107m. What is the distance of container from earth?
View SolutionWhat is the average distance of moon from earth ?
View Solution
Knowledge Check
- Question 1 - Select One or More
What is the approximate distance (in km) of the moon from Earth?
A3,84,400B2,80,500C5,60,000D6,00,000 - Question 2 - Select One
If the distance of the moon from earth is and the period of revolution is Tm,then the mass of the earth is
A4π2r2mGTmB4π2r3mGT2mC4π2rmGTmD4π2rmGT2m
The moon is observed from two diametrically extremes A and B on earth. The angle of parallax is found to be 1∘54'. If the diameter of the earth is about 1.276×107 m, estimate the distance of the moon from the earth.
View SolutionThe moon subtends an angle of 57 minutes at two diametrically opposite points on earth. Calculate the distance of the moon from the earth. Given, diameter of earth =1.276×107m.
View SolutionA distant star is observed from two diametrically opposite points on earth. If both the points are at distance 1.27×107m and angle subtended by both the points at star is 0.80'', calculate the distance between earth and the star.
View SolutionAssuming the distance of the earth from the moon to be 38,400 km and the angle subtended by the moon at the eye of a person on the earth to be 31’, find the diameter of the moon.
View SolutionThe angle subtended by the moon's diameter at a point on the earth is about 0.50∘ . Use this and the fact that the moon is about 384000km away to find the approximate diameter of the moon .
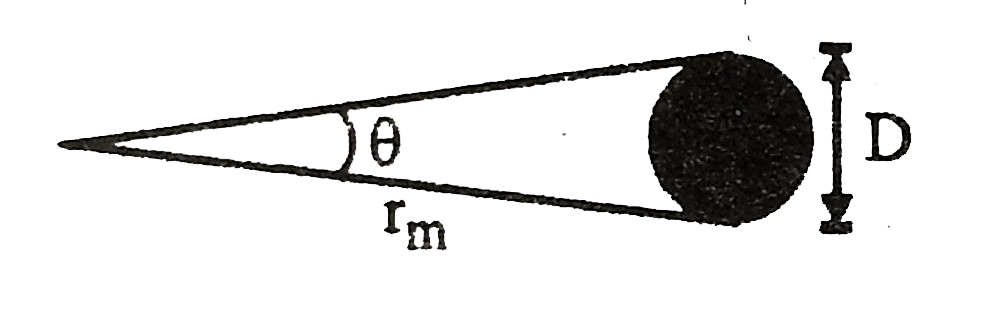 View Solution
View SolutionThe moons distance from the earth is 360,000kms and its diameter subtends an angle of 31 at the eye of the observer. Find the diameter of the moon.
View Solution

