The velocity of a body of mass 2kg as a function of t is given by υ(t)=2tˆi+t2ˆj Find the momentum and force acting on it at time t=2s.
Video Solution
Text Solution
Here, m=2kg
υ(t)=2tˆi+22ˆj
t=2s
→υ=4ˆi+4ˆj=4(ˆi+ˆj)
∴→p=−−→mυ=2(4ˆi+4ˆj)=[8ˆi+8ˆj]kgms−1
Also, →a=d→vdt=ddt[atˆi+t2ˆj]
→a=2ˆi+2tˆj
At t=2s,→a2ˆi+4ˆi
→F=m→a=2(2ˆi+4ˆj)=(4ˆi+8ˆi)N.
|
Updated on:21/07/2023
Similar Questions
Knowledge Check
- Question 1 - Select One
The position (x) of a particle of mass 1 kg moving along X-axis at time t is given by (x=12t2) metre. Find the work done by force acting on it in time interval from t=0 to t=3s.
A4.2 JB5.4 JC4.6 JD4.5 J - Question 2 - Select One
The time dependence of the position of a particle of mass m = 2 is given by →r(t)=2tˆi−3t2ˆj Its angular momentum, with respect to the origin, at time t = 2 is :
A−34(ˆk−ˆi)B−48ˆkC36ˆkD48(ˆi+ˆj) - Question 3 - Select One
velocity of a body of mass 2kg moving in x−y plane is given by →v=(2ˆi+4tˆj)m/s, where t is the time in second. The power delivered to the body by the resultant force acting on it at t=5sec is.
A80 WB160 WC40 WD100 W
The position (x) of a particle of mass 2 kg moving along x-axis at time t is given by x=(2t3) metre. Find the work done by force acting on it in time interval t=0 to t=2 is :-
View SolutionThe mass of a body is 2.5 Kg. it is in motion and its velocity υafter time t is υ=t33+t22+1
Calculate the force acting on the body at the time t =3 s.View SolutionThe position time graph of a body of mass 2kg is as given in What is the impulse on the body at t=0 s and t=4s ?
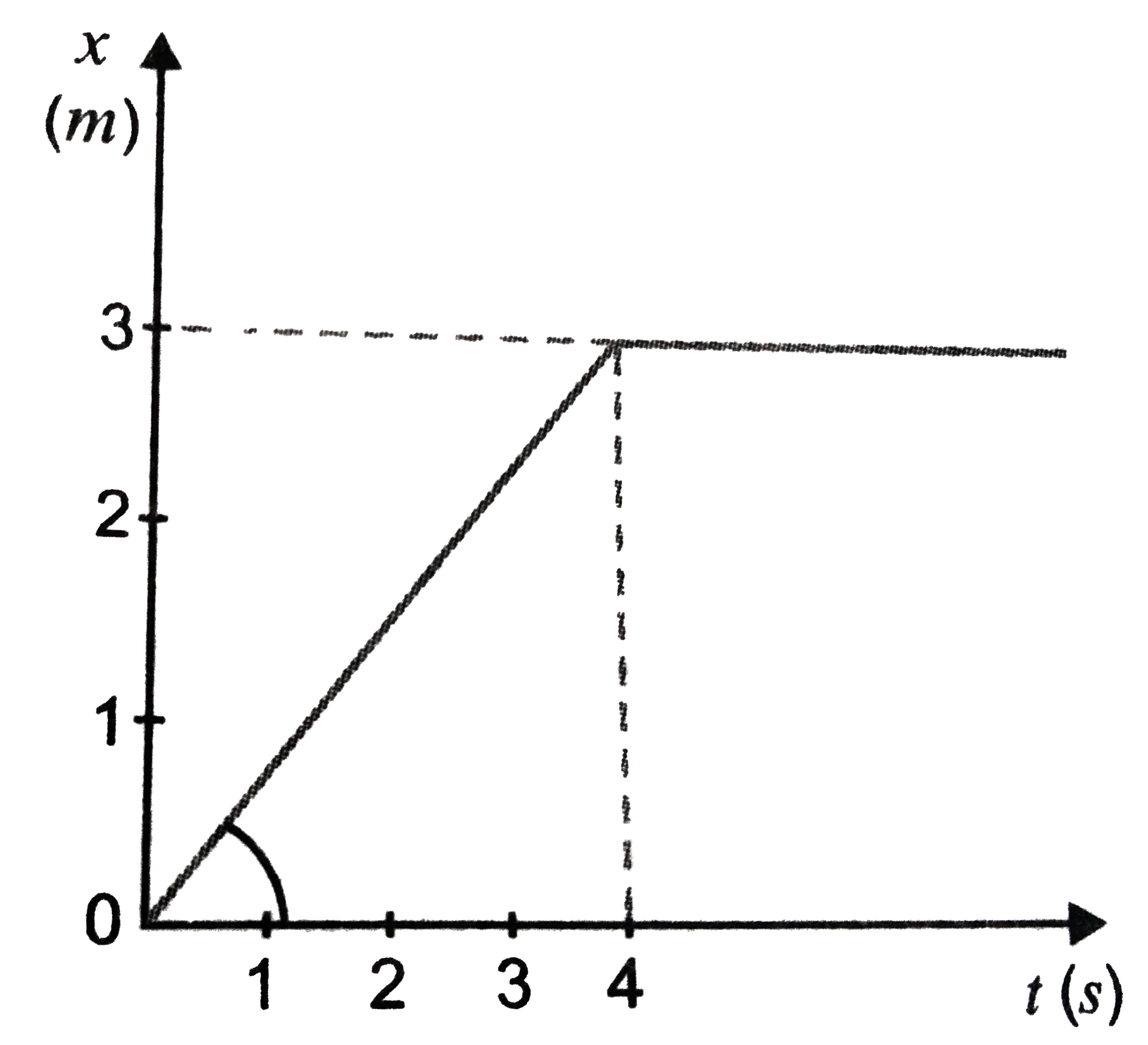 . View Solution
. View SolutionThe displacement of a body of mass 2 kg varies with time t as S=t2+2t, where S is in m and t is in s. The work done by all the forces acting on the body during the time interval t=2 to t=4 s is
View SolutionThe velocity vector of the motion described by the position vector of a particle, r=2tˆi+t2ˆj is given by
View Solution

