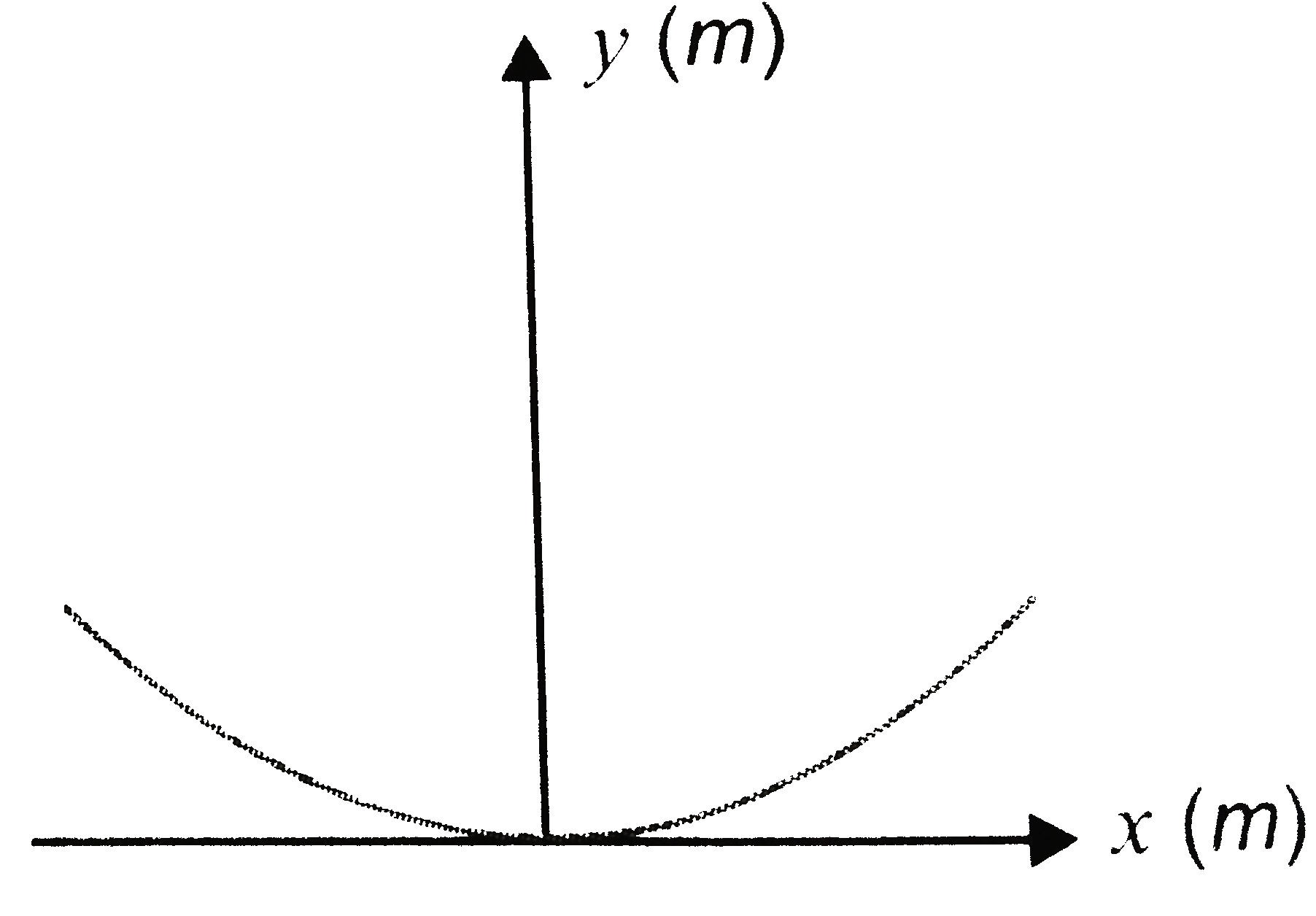
A parabolic bow1 with its bottom at origin has the shape y=x220 where x and y are in metre The maximum height at which a small mass m can be placed on the bowl without slipping is (coeff of static friction 0.5
 .
.
1.25Nm
2.5m
1.0m
4.0m
The correct Answer is:a
As y=x220,dydx=2x20
Angle of repose θr is such that
μ=tanθr=dydx=2x20=x10
x=10μ=10×0.5=5m
Corresponding value of y=x220=5220=1.25m .
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Integer Type Questions7videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions17videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Problems for practice112videos
LAWS OF MOTION
- BOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension4videos
KINEMATICS
- BOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks5videos
MATHEMATICAL TOOLS
Similar Questions
A particle moves with a uniform speed of 2m/s in x-y plane according to the equation y=4x2 , where x and y are in metre. The acceleration of the particle along y -axis while passing through the origin has magnitude?
View SolutionA block of mass 'm' is placed on a rough surface with a vertical cross section of y=x36. If the coefficient of friction is 0.5, the maximum height above the ground at which the block can be placed without slipping is .
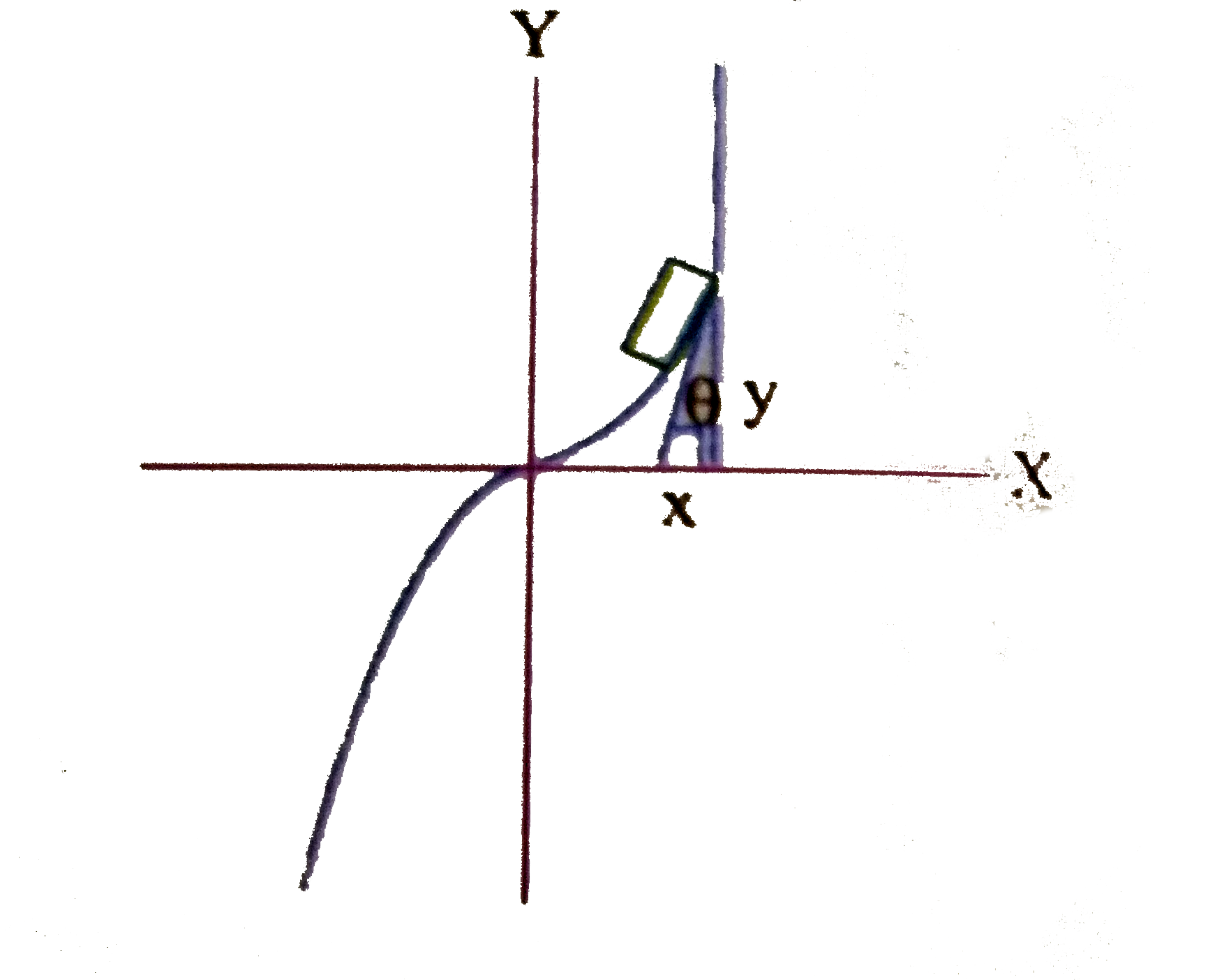 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A particle of mass 1 kg is moving along the line y = x + 2 (here x and y are in metres) with speed 2 m/s. The magnitude of angular momentum of particle about its origin is
A4kgm2s−1B2√2kgm2s−1C4√2kgm2s−1D2kgm2s−1 - Question 2 - Select One
A particle of mass 1 kg is moving along the line y=x+2 (here x and y are in metres) with speed 2m/s. The magnitude of angular momentum of paticle about origin is -
A4kg−m2/sB2√2kg−m2/sC4√2kg−m2/sD2kg−m2/s - Question 3 - Select One
If x2+x+y2−1=0, where x > 0 and y > 0 then maximum value of x√y is
A13√2B12√2C√2D2√2
If x2+x=1−y2 , where x>0,y>0, then find the maximum value of x√y.
View SolutionThe equation of a progressive wave is given by y=0.5sin(20x−400t) where xand y are in metre and tis in second. The velocity of the wave is
View SolutionThe equation of a travelling wave is given by
y = 0.5 sin(20x - 400t)
where x and y are in metre and t is in second. The velocity of the wave is :View SolutionThe equation of a travelling wave is given by
y = 0.5 sin(20x - 400t)
where x and y are in metre and t is in second. The velocity of the wave is :View SolutionA block of mass is placed on a surface with a vertical cross section given by y=x36. If the coefficient of friction is 0.5, the maximum height above the ground at which the block can be placed without slipping is:
View Solution

