Two perfectly elastic particles and of equal masses travelling along a line joining them with velocities and respectively collide. Their velocities after the elastic collision will be (in m/s) respectively
The correct Answer is:C
To solve the problem of two perfectly elastic particles A and B colliding, we will use the principles of conservation of momentum and conservation of kinetic energy. Here’s the step-by-step solution:
Step 1: Understand the Initial Conditions
- Let the mass of both particles A and B be m.
- The initial velocity of particle A (U1) = 15 m/s.
- The initial velocity of particle B (U2) = 10 m/s.
Step 2: Apply Conservation of Momentum
In an elastic collision, the total momentum before the collision is equal to the total momentum after the collision. Therefore, we can write:
\( m \cdot U1 + m \cdot U2 = m \cdot V1 + m \cdot V2 \)
Since the masses are equal, we can cancel m from the equation:
\( U1 + U2 = V1 + V2 \)
Substituting the values:
\( 15 + 10 = V1 + V2 \)
\( 25 = V1 + V2 \) (Equation 1)
Step 3: Apply Conservation of Kinetic Energy
In an elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision:
\( \frac{1}{2} m U1^2 + \frac{1}{2} m U2^2 = \frac{1}{2} m V1^2 + \frac{1}{2} m V2^2 \)
Again, canceling m and 12 gives us:
\( U1^2 + U2^2 = V1^2 + V2^2 \)
Substituting the values:
\( 15^2 + 10^2 = V1^2 + V2^2 \)
\( 225 + 100 = V1^2 + V2^2 \)
\( 325 = V1^2 + V2^2 \) (Equation 2)
Step 4: Solve the Equations
Now we have two equations:
1. \( V1 + V2 = 25 \)
2. \( V1^2 + V2^2 = 325 \)
From Equation 1, we can express \( V2 \) in terms of \( V1 \):
\( V2 = 25 - V1 \)
Substituting \( V2 \) into Equation 2:
\( V1^2 + (25 - V1)^2 = 325 \)
Expanding the equation:
\( V1^2 + (625 - 50V1 + V1^2) = 325 \)
Combining like terms:
\( 2V1^2 - 50V1 + 625 - 325 = 0 \)
\( 2V1^2 - 50V1 + 300 = 0 \)
Dividing the entire equation by 2:
\( V1^2 - 25V1 + 150 = 0 \)
Step 5: Use the Quadratic Formula
To find \( V1 \), we will use the quadratic formula:
\( V1 = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \)
Where \( a = 1, b = -25, c = 150 \):
\( V1 = \frac{25 \pm \sqrt{(-25)^2 - 4 \cdot 1 \cdot 150}}{2 \cdot 1} \)
\( V1 = \frac{25 \pm \sqrt{625 - 600}}{2} \)
\( V1 = \frac{25 \pm \sqrt{25}}{2} \)
\( V1 = \frac{25 \pm 5}{2} \)
Calculating the two possible values:
1. \( V1 = \frac{30}{2} = 15 \) m/s
2. \( V1 = \frac{20}{2} = 10 \) m/s
Step 6: Find \( V2 \)
Using \( V2 = 25 - V1 \):
1. If \( V1 = 15 \) m/s, then \( V2 = 25 - 15 = 10 \) m/s.
2. If \( V1 = 10 \) m/s, then \( V2 = 25 - 10 = 15 \) m/s.
Conclusion
Thus, after the collision, the velocities of particles A and B will be:
- Velocity of particle A (V1) = 10 m/s
- Velocity of particle B (V2) = 15 m/s
Final Answer
The final velocities after the elastic collision are:
- Particle A: 10 m/s
- Particle B: 15 m/s
- Let the mass of both particles A and B be m.
- The initial velocity of particle A (U1) = 15 m/s.
- The initial velocity of particle B (U2) = 10 m/s.
In an elastic collision, the total momentum before the collision is equal to the total momentum after the collision. Therefore, we can write:
\( m \cdot U1 + m \cdot U2 = m \cdot V1 + m \cdot V2 \)
Since the masses are equal, we can cancel m from the equation:
\( U1 + U2 = V1 + V2 \)
Substituting the values:
\( 15 + 10 = V1 + V2 \)
\( 25 = V1 + V2 \) (Equation 1)
In an elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision:
\( \frac{1}{2} m U1^2 + \frac{1}{2} m U2^2 = \frac{1}{2} m V1^2 + \frac{1}{2} m V2^2 \)
Again, canceling m and 12 gives us:
\( U1^2 + U2^2 = V1^2 + V2^2 \)
Substituting the values:
\( 15^2 + 10^2 = V1^2 + V2^2 \)
\( 225 + 100 = V1^2 + V2^2 \)
\( 325 = V1^2 + V2^2 \) (Equation 2)
Now we have two equations:
1. \( V1 + V2 = 25 \)
2. \( V1^2 + V2^2 = 325 \)
\( V2 = 25 - V1 \)
\( V1^2 + (25 - V1)^2 = 325 \)
Expanding the equation:
\( V1^2 + (625 - 50V1 + V1^2) = 325 \)
Combining like terms:
\( 2V1^2 - 50V1 + 625 - 325 = 0 \)
\( 2V1^2 - 50V1 + 300 = 0 \)
Dividing the entire equation by 2:
\( V1^2 - 25V1 + 150 = 0 \)
To find \( V1 \), we will use the quadratic formula:
\( V1 = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \)
Where \( a = 1, b = -25, c = 150 \):
\( V1 = \frac{25 \pm \sqrt{(-25)^2 - 4 \cdot 1 \cdot 150}}{2 \cdot 1} \)
\( V1 = \frac{25 \pm \sqrt{625 - 600}}{2} \)
\( V1 = \frac{25 \pm \sqrt{25}}{2} \)
\( V1 = \frac{25 \pm 5}{2} \)
1. \( V1 = \frac{30}{2} = 15 \) m/s
2. \( V1 = \frac{20}{2} = 10 \) m/s
Using \( V2 = 25 - V1 \):
1. If \( V1 = 15 \) m/s, then \( V2 = 25 - 15 = 10 \) m/s.
2. If \( V1 = 10 \) m/s, then \( V2 = 25 - 10 = 15 \) m/s.
Thus, after the collision, the velocities of particles A and B will be:
- Velocity of particle A (V1) = 10 m/s
- Velocity of particle B (V2) = 15 m/s
The final velocities after the elastic collision are:
- Particle A: 10 m/s
- Particle B: 15 m/s
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Comprehension
9 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - Integer Type Questions
9 Videos
- WORK, ENERGY AND POWERBOOK - PRADEEPCHAPTER - WORK, ENERGY AND POWEREXERCISE - NCERT( Multiple choide questions-1)
21 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
Two balls of masses 10 kg and 20 kg approach each other with velocities 30 m/s and 20 m/s respectively . Calculate their velocities after they undergo a perfectly eleastic collision .
View SolutionA 10kg ball and a 20kg ball approach each other with velocities 20m/s and 10m/s respectively. What are their velocities after collision, if the collision is perfectly elastic ?
View Solution
Knowledge Check
Two elastic bodies P and Q having equal mases are moving along the same line with velocities of 16 m/s and 10 m/s respectively. Their velocities after the elastic collision will be in m/s :
A0 and 25B5 and 20C10 and 16D20 and 5Two equal masses m1 and m2 moving along the same straight line with velocites +3m/s and −5m/s respectively collide elastically. Their velocities after the collision will be respectively.
A+4m/s for bothB−3m/sand+5m/sC−4m/sand+4m/sD−5m/sand+3m/sTwo particles A and B of masses 10 kg and 38 kg, respectively, are moving along the same straight line with velocities 15 m/s and 3 m/s, respectively, in the same direction. After elastic collision, the velocities of A and B are vAand vB, respectively, in the direction of initial motion. Then
 AvA=20m/s,vB=8msBvA=–4m/s,vB=8m/sCvA=16ms,vB=28m/sDvA=–5m/s,vB=10m/s
AvA=20m/s,vB=8msBvA=–4m/s,vB=8m/sCvA=16ms,vB=28m/sDvA=–5m/s,vB=10m/s
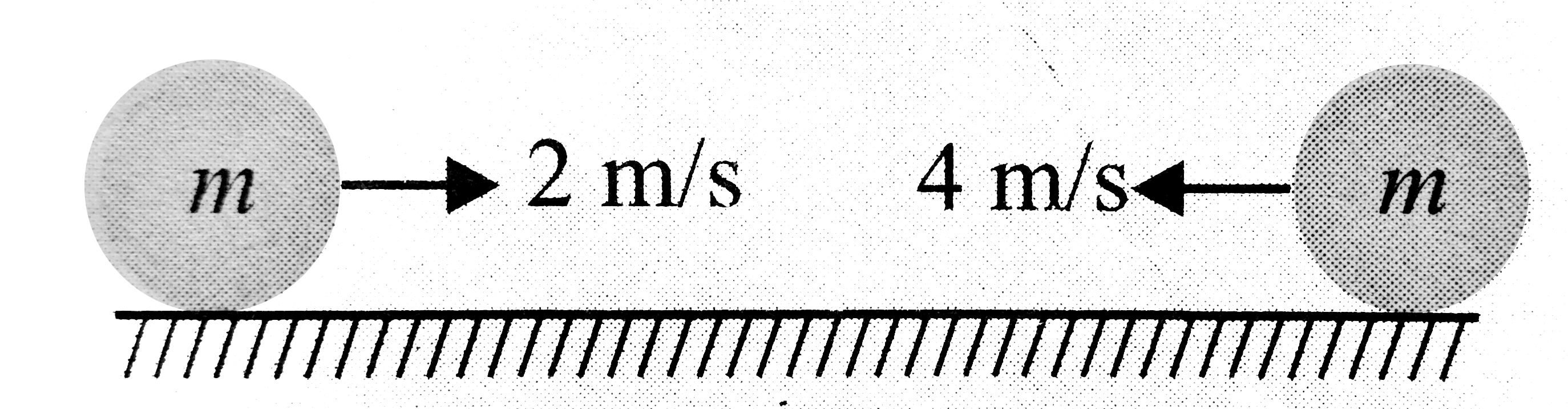
Two identical balls are approaching towards each other on a straight line with velocity 2m/s and 4m/s, respectiely. Find the final velocities after elastic collision between them.
View SolutionTwo identical bodies A and B moving with velocities 13 m/s and - 15 m/s respectively collide head-on elastically. What will be their velocities after collision?
View SolutionTwo identiacal balls A and B having velocities of 0.5 m/s and 0.3 m/s respectively collide elastically in one dimension. The velocities of B and A after the collision respectively will be
View SolutionTwo identical balls AandB having velocity of 0.5m/sand−0.3m/s respectively collide elastically in one dimension. The velocities of BandA after the collision respectively will be
View SolutionTwo identical balls AandB having velocity of 0.5m/sand−0.3m/s respectively collide elastically in one dimension. The velocities of BandA after the collision respectively will be
View Solution
