A very small particle rests on the top of a hemisphere of radius 20cm. Calculate the smallest horizontal velocity to be given to it if it is to leave the hemisphere without sliding down its surface, take g=9.8m/s2.
The particle will leave the hemisphere, when normal reaction R becomes zero. In that event,
mυ2r=mg
∴υ=√rg=√20100×9.8=√1.96=1.4m/s
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - CONCEPTUAL PROBLEMS II.
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - VERY SHORT ANSWER QUESTIONS I.
2 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - CONCEPTUAL PROBLEMS I.
2 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
- THERMODYNAMICSBOOK - PRADEEPCHAPTER - THERMODYNAMICSEXERCISE - Assertion- Reason Type Questions
19 Videos
Similar Questions
A particle of mass m is released from the top of a smooth hemisphere of radius R with the horizontal speed μ. Calculate the angle with verticle where it loses contact with the hemisphere.
View SolutionA particle rests on the top of a smooth hemisphere of radius r. It is imparted a horizontal velocity of √ηgr. Find the angle made by the radius vector joining the particle with the vertical at the instant the particle losses contact with the sphere.

View Solution
Knowledge Check
A small particle rest on the top of a hemisphere of radius 20 cm .The smallest horizontal velocity os given to it , if it is to leave the hemisphere without sliding down to its surface ,is
A0.7m/sB1.4m/sC2.8m/sD5.6m/sA partcle rests on the top of a hemisphere of radius R. Find the smallest horizontal velocity that must be imparted to the particle if it is to leave the hemisphere without sliding down :
A√gRB√2gRC√3gRD√5gRA partcle rests on the top of a hemisphere of radiu R. Find the smallest horizontal velocity that must be imparted to the particle if it is to leave the hemisphere without sliding down it-
A√gRB√2gRC√3gRD√5gR
Find the horizontal velocity of the particle when it reach the point Q. Assume the block to be frictionless. Take g=9.8m/s2.
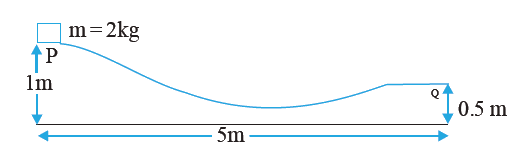
View SolutionA particle slides down from the top outside smooth surface of a fixed sphere of radius a=10m . The initial horizontal velocity to be imparted to the particle at the top is 5Km/s ,if it leaves the surface at a point whose vertical height above the centre of sphere is 3a/4. Find the value of K
View SolutionA particle is moving along a circle of radius 20cm, with a linear velocity of 2m//s. Calculate the angular velocity of the particle.
View SolutionA body rest on the top of a hemisphere of radius R. What will be the least horizontal velocity imparted to it , if it has to leave the hemisphere without sliding down?
View SolutionA small disc is on the top of a hemisphere of radius R. What is the smallest horizontal velocity v that should fbe given to the disc for it to leave the hemisphere and not slide down it?[There is no friction]
View Solution
