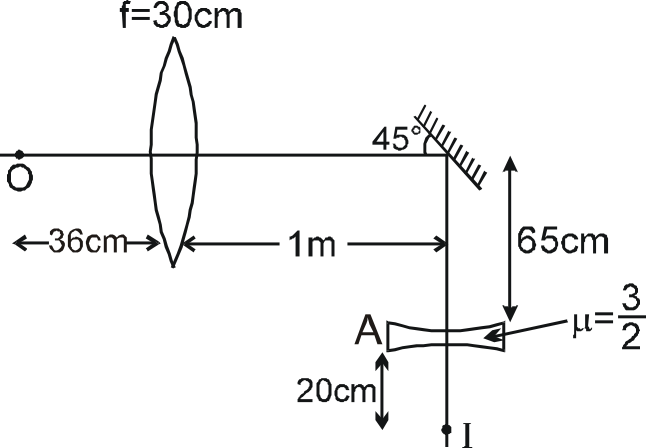
The final image of the object O shown in the figure is formed at point 20 cm below a thin equi-concave
lens, which is at a depth of 65 cm from principal axis. From the given geometry, calculate the radius of
curvature in cm of lens kept at "A". (Refractive index of equi-convex lens is 1.5 and placed in air.
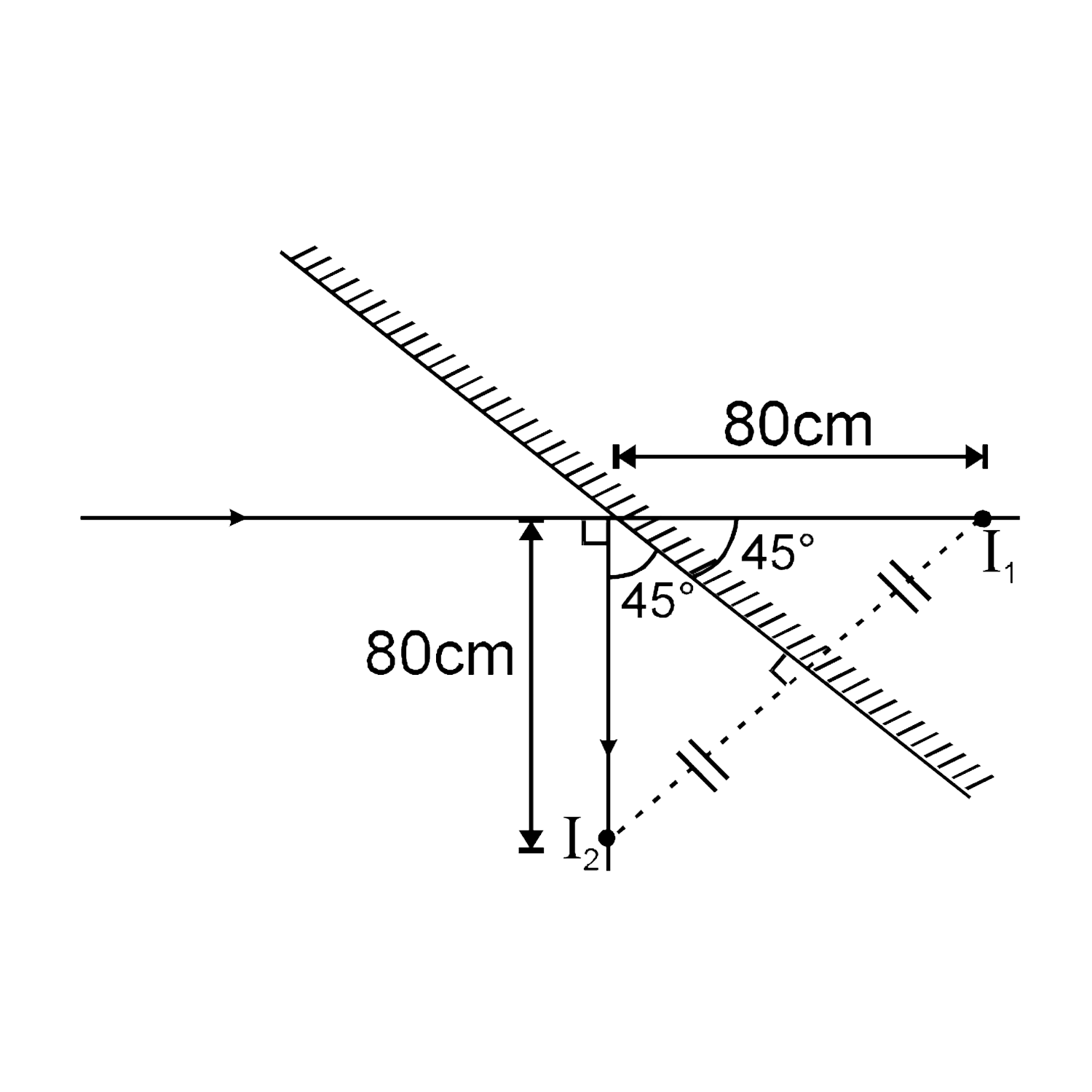
Image formation due to convex lens
1v−1−36=130⇒v=30×366=180cm
This image will act like a virtual object for mirror and after reflection from mirror its image (shown by I2 ) will be formed at 80 cm below optical axis of convex lens. ltbr> For concave lens, this image will be object at a position of 15 cm below the lens for final image formed by concave lens
120−115=1f 1f=−5300
Also
1f=(μ−1)(−1R−1R)
or −5300=(32−1)(−2R)⇒R=3005
Topper's Solved these Questions
Similar Questions
A plano convex lens of radius of curvature 30 cm and refractive index 1.5 is kept in air. Find its focal length (in cm).
View SolutionSurfaces of a thin equi-convex glass lens have radius of curvature R. Paraxial rays are incident on it . If the final image is formed after n internal reflections, calculate distance of this image from pole of the lens. Refractive index of glass is μ.
View Solution
Knowledge Check
The power in dioptre of an equi-convex lens with radii of curvature of 10cm and refractive index 1.6 is
A+12B+18C+1.2D+1.8The size of the image of an object,which is at infinity, as formed by a convex lens of focal length 20 cm is placed between the convex lens and the image at the distance of 26 cm from the convex lens, the new size of the image is
A1.25 cmB2.5 cmC1.05 cmD2 cmA bi-convex lens is formed with two thin plano-convex lenses as shown in the figure. Refractive index n of the first lens is 1.5 and that of the second lens is 1.2. Both the curved surface are of the same radius of curvature R=14 cm. For this bi-convex lens, for an object distance of 40 cm, the image distance will be
 A−280.0cmB40.0 cmC21.5 cmD13.3 cm
A−280.0cmB40.0 cmC21.5 cmD13.3 cm
An object 50cm tall is placed on the principal axis of a convex lens. Its 20cm tall image is formed on the screen placed at a distance of 10cm from the lens. Calculate the focal length of the lens.
View SolutionAn object is placed at a distance of 20cm from a equi-concave lens of focal length 30cm, whose one surface is silvered. If the radius of curvature of the silvered surface is 60cm, find the position and nature of the image.
View SolutionAn object 50 cm tall is placed on the principle axis of a convex lens. Its 20 cm tall image is formed on the screen placed at a distance of 10 cm from the lens. Calculate the focal length of the lens.
View SolutionThe radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
View SolutionA thin equi-convex lens having radius of curvature 10 cm is placed as shown in figure. Calculate focal length of the lens, if parallel rays are incident as shown.
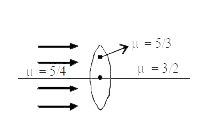
View Solution
