In a series RLC AC circult, the frequency of source can be varied. When frequency is varied gradually in one direction from $f_{1}$ to $f_{2}$ , the power is found to be maximum at $f_{1}$ . When frequency is varied gradually at the other direction from $f_{1}$ to $f_{3}$ , the power is found to be same at $f_{1}$ and $f_{3}$ . Match the proper entries from column-2 to column-1 using the codes given below the columns, (consider $f_{1} > f_{2}$ )
$\begin{matrix} Column-I & Column-II when the frequency is equal to & The circuit is or can be AM: arithmatic mean GM:geometic mean (P) AM of f_{1} and f_{2} & (1) capacitance (Q) GM of f_{1} and f_{3} & (2) inductive (R) AM of f_{1} and f_{3} & (3) resistive (S) GM of f_{1} and f_{3} & (4) at resonance \end{matrix}$

$\begin{matrix} P & Q & R & S (A) & 4 & 3 & 2 & 4 \end{matrix}$

$\begin{matrix} P & Q & R & S (B) & 3 & 1 & 3 & 2 \end{matrix}$

$\begin{matrix} P & Q & R & S (C) & 2 & 1 & 2 & 3 \end{matrix}$

$\begin{matrix} P & Q & R & S (D) & 1 & 3 & 3 & 4 \end{matrix}$

Text Solution

Verified by Experts

Power $i^{2} R$ graph of 'I' v s f is

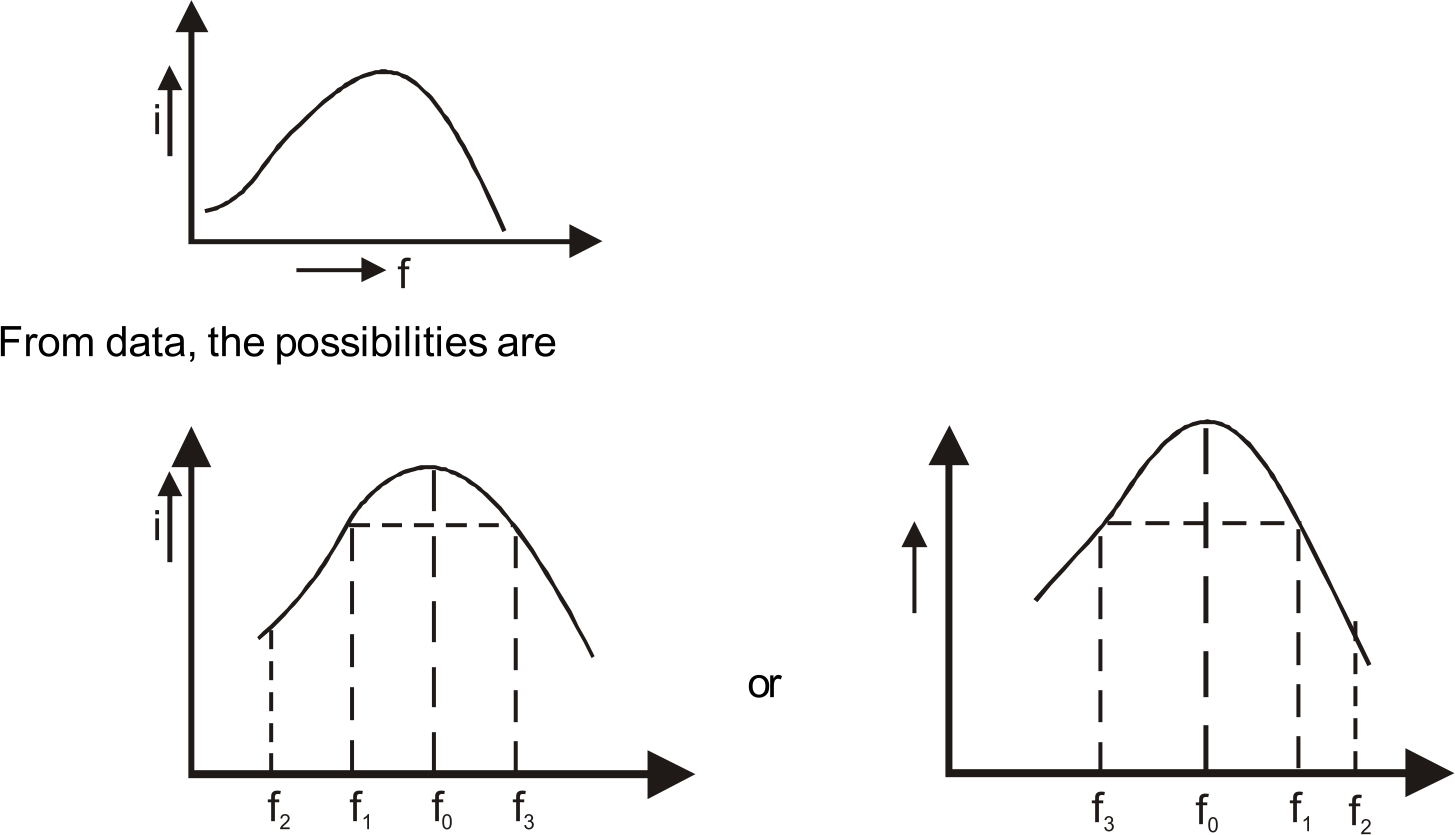
$f_{0}$ is resonant frequency $\Rightarrow$ means as circuit being resistive.
The circuit is capacitive when $f < f_{0}$ and inductive when $f > f_{0}$
Power at $f_{1}$ and $f_{3}$ same $\Rightarrow$ is same $\Rightarrow$ z same
$\Rightarrow 2 π f_{1} L - \frac{1}{2 π f_{1} C} = \frac{1}{2 π f_{3} C} - 2 π f_{3} L$
$\Rightarrow 2 π L (f_{1} + f_{3}) = \frac{1}{2 π C} (\frac{1}{f_{1}} + \frac{1}{f_{3}})$
$\Rightarrow f_{1} f_{3} = \frac{1}{4 π^{2} L C} \Rightarrow \sqrt{f_{1} f_{3}} = \frac{1}{2 π \sqrt{L C}} = \frac{ω_{0}}{2 π}$
$A M > G M \Rightarrow \frac{f_{1} + f_{3}}{2} > f_{0}$
$\Rightarrow$ inductive at frequency = $\frac{f_{1} + f_{3}}{2}$

Updated on:21/07/2023

Let $f_{1}$ be the frequency
A $f_{1} - f_{2} = f_{3}$
B $f_{2} - f_{1} = f_{3}$
C $f_{3} = \frac{1}{2} (f_{1} + f_{2})$
D $f_{1} + f_{2} = f_{3}$ .
If the frequency of the source e.m.f. in an AC circuit is n, the power varies with a frequency:
A $n$
B $2 n$
C $n / 2$
Dzero
If the frequency of the source e.m.f. in an AC circuit is n, the power varies with a frequency:
An
B2n
Cn/2
Dzero