If y=f(x)andx=g(y) are inverse functions of each other, then
A
g''(y)=1f''(x)
B
g''(y)=f''(x)(f''(x))3
C
g''(y)=f'(x)(f'(x))3
D
None of these
Text Solution
The correct Answer is:B
|
Topper's Solved these Questions
- DIFFERENTIATION BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIATION EXERCISE - Exercise (Statement I And Ii Type Questions)
10 Videos
- DIFFERENTIATION BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIATION EXERCISE - Exercise (Passage Based Questions)
16 Videos
- DIFFERENTIATION BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIATION EXERCISE - Exercise For Session 10
4 Videos
- DIFFERENTIAL EQUATION BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIAL EQUATION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
26 Videos
- DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS BOOK - ARIHANT MATHSCHAPTER - DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
7 Videos
Similar Questions
Knowledge Check
If y=f(x) and x=g(y) are inverse of each other. Then g'(y) and g(y) in terms of derivative of f(x) is
A−f(x)[f'(x)]3Bf(x)[f'(x)]2C−f(x)[f'(x)]2DNone of theseLet f(x)=x3+x+1 and let g(x) be its inverse function then equation of the tangent to y=g(x) at x = 3 is
Ax−4y+1=0Bx+4y−1=0C4x−y+1=0D4x+y−1=0If g is inverse of function f and f'(x)=sinx, then g'(x)=
Acosec{g(x)}Bsin{g(x)}C1sin{g(x)}Dnone of these
If f(x)andg(x) are mirror image of each other through y=x and such that f(x)=ex+x then the value of g'(1) is
View SolutionLet f(x)=x3+x+1 and let g(x) be its inverse function then equation of the tangent to y=g(x) at x = 3 is
View SolutionIf a and b vary inversely to each other, then find the values of x, y, z.
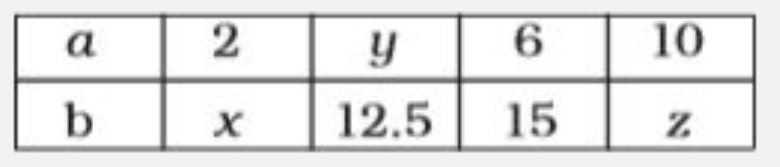
View SolutionLet a curve y=f(x) be defined parametrically as y=t3+t2+1,x=t2+2,t>0. If g(x) is inverse function of f(x) then g'(3) is
View SolutionIf g(y) is inverse of function f:R→R given by f(x)=x+3, then g(y)=
View Solution
