Solve the following questions.
Diagonals of a quadrilateral ABCD intersect in point Q. if 2 QA = QC, 2 QB = QD, then prove that DC = 2AB.
Step by step video & image solution for Solve the following questions. Diagonals of a quadrilateral ABCD intersect in point Q. if 2 QA = QC, 2 QB = QD, then prove that DC = 2AB. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
- BOOK - TARGET PUBLICATIONCHAPTER - SIMILARITY EXERCISE - Additonal Problems for Practice(Based on Practice Set 1.4)8videos
SIMILARITY
- BOOK - TARGET PUBLICATIONCHAPTER - QUESTION FROM STD. IXEXERCISE - Statistics14videos
QUESTION FROM STD. IX
- BOOK - TARGET PUBLICATIONCHAPTER - STATISTICSEXERCISE - Problem Set-621videos
STATISTICS
Similar Questions
Knowledge Check
- Question 1 - Select One
Diagonals AC and BD of a quadrilateral intersect at O. It AO:OC=1:2=BO:ODandAB=16cm then DC is
A16cmB8cmC4cmD32cm
In Fig 9.25, diagonals AC and BD of quadrilateral ABCD intersect at O such that OB = OD.If AB=CD , then show that
View SolutionIn the figure, diagonals AC and BD of quadrilateral ABCD intersect at 0 such that OB = OD. If AB = CD, then show that

DA I I CB or ABCD is a parallelogram.View SolutionThe diagonals AC and BD of a quadrilateral ABCD intersect at point 'O'. If BO = OD, then prove that the areas of ΔABCandΔADC are equal
View SolutionIn the figure, diagonal AC of a quadrilateral ABCD bisects the angles A and C. Prove that AB = AD and CB = CD.
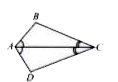 View Solution
View SolutionIn the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect point O. Prove that :
AB+BC+CD+DA<2(AC+BD)
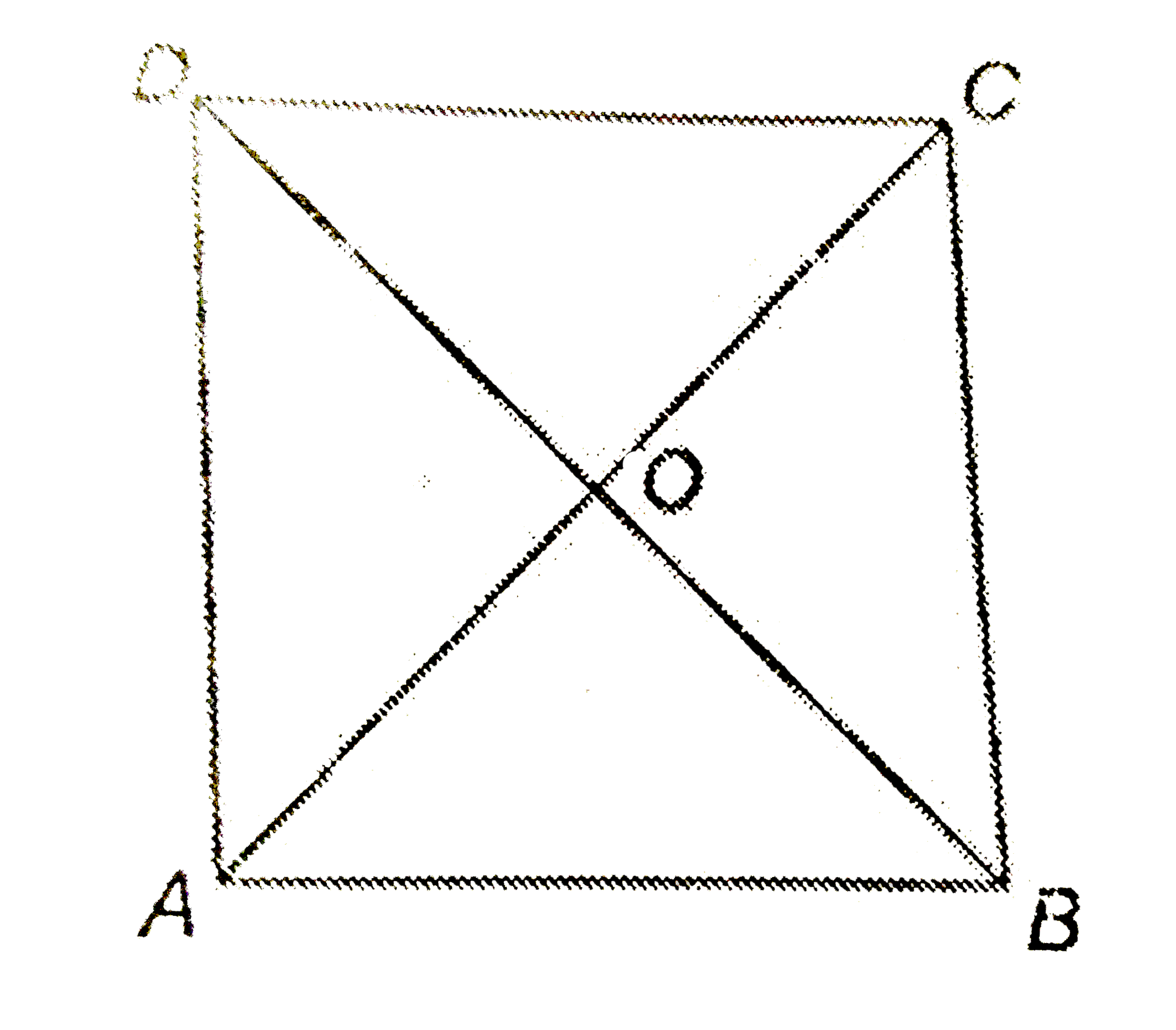 View Solution
View SolutionIn the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect at O.
If BO=OD, prove that
ar(△ABC)=ar(△ADC).
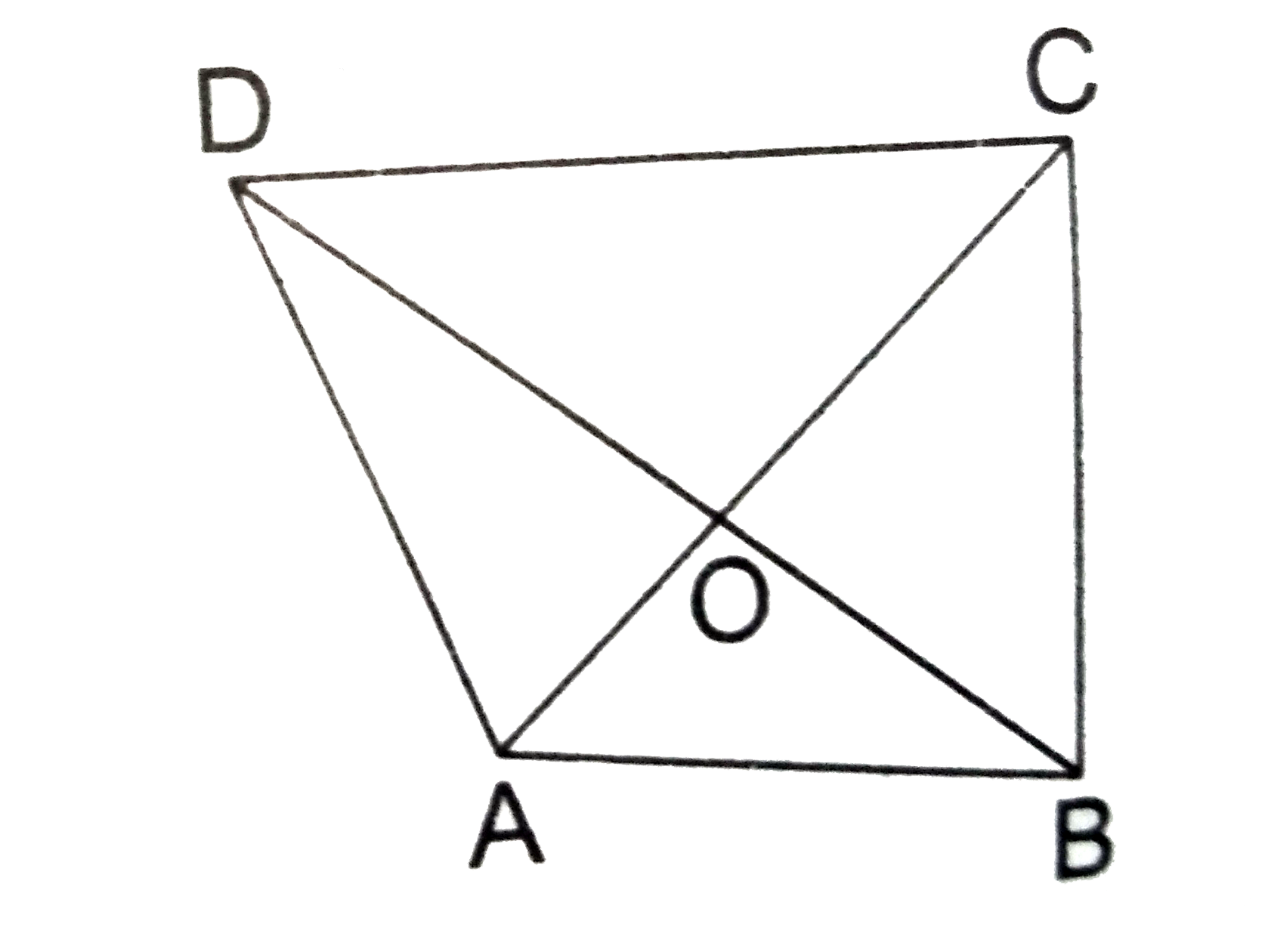 View Solution
View SolutionThe diagonal of a quadrilateral ABCD intersect each other at the point D such that AOBO=CODO. Show that ABCD is a trapezium.
View Solution

