If the angle of incidence and angle of emergence of a light ray falling on a glass slab are i and e respectively, prove that, i = e.
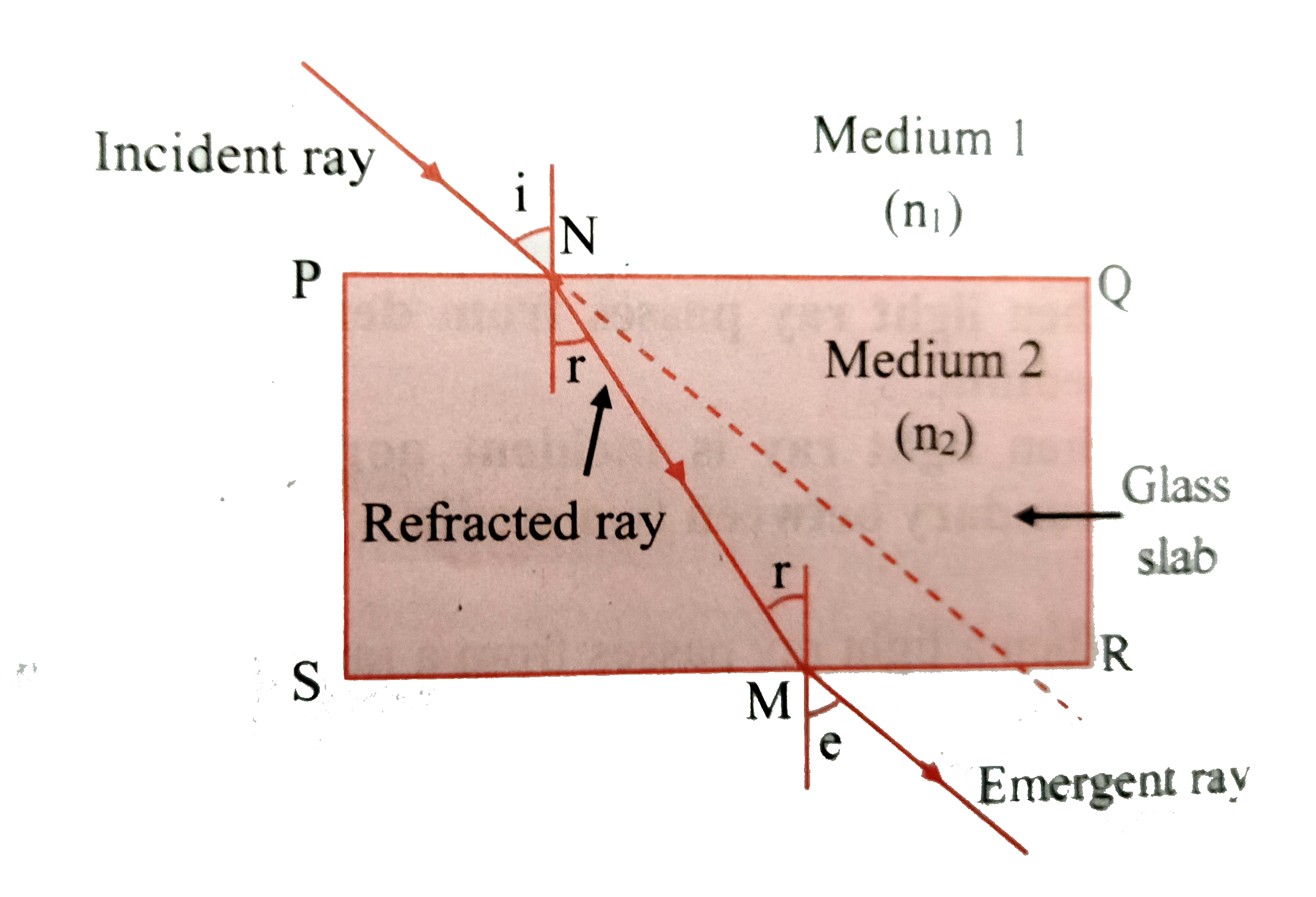
i. In the above figure,
i = angle of incidence,
r = angle of refraction,
e = angle of emergence.
At point N, using Snell's law,
glassnair=sinisinr ….(1)
At point M, using Snell's law,
airnglass=sinrsine ......(2)
But glassnair=1airnglass
∴ sinisinr=1sinrsine ....[From (1) and (2)]
∴ sinisinr=sinesinr
∴ sini=sine
∴ ∠i=∠e
ii. Hence, for the refraction of light through a glass slab angle of incidence is equal to angle of emergence, i.e., i=e.
Topper's Solved these Questions
- Refraction of lightBook:TARGET PUBLICATIONChapter:Refraction of lightExercise:Give reasons
Explore 2 Videos
- Refraction of lightBook:TARGET PUBLICATIONChapter:Refraction of lightExercise:Distinguish between
Explore 1 Video
- Refraction of lightBook:TARGET PUBLICATIONChapter:Refraction of lightExercise:Match the following
Explore 1 Video
- MODEL QUESTION PAPER PART-2Book:TARGET PUBLICATIONChapter:MODEL QUESTION PAPER PART-2Exercise:QUESTIONS
Explore 2 Videos
- SOCIAL HEALTH Book:TARGET PUBLICATIONChapter:SOCIAL HEALTH Exercise:CHAPTER ASSESSMENT
Explore 3 Videos
Similar Questions
With a neat labeeled diagram, prove that if the angle of incidence and angle of emergence of a light ray falling on a glass slab are I and e respectively, then i=e.
View SolutionProve that i = e, If the angle of incidence and angle of emergence subtended by a light ray falling on a surface of glass slab are i and e respectively. How much angular deviation is suffered by the incident ray.
View Solution
Knowledge Check
In the experiment on tracing the path of a ray of light passing through a rectangular glass slab, the correct setting of the protractor (D), for measuring the angle of incidence (∠I), and the angle of emergence (∠e) correspond, respectively, to diagrams :
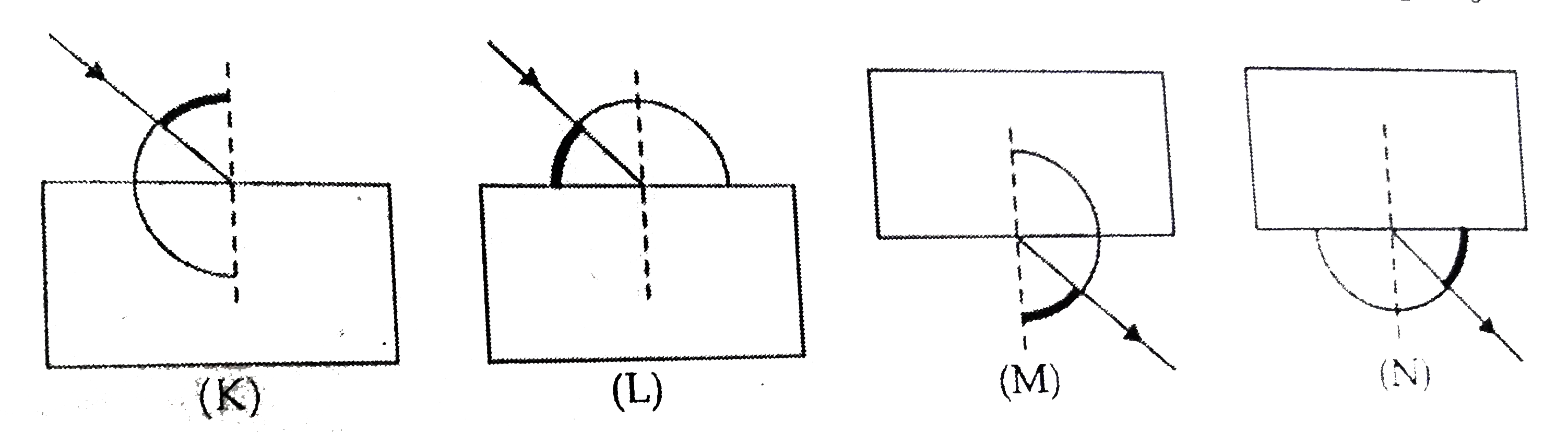 AK and MBK and NCL and MDL and N
AK and MBK and NCL and MDL and NThe angle between the incident and the emergent ray from a glass slab is
A90∘B0∘Cless than 90∘Dmore than 90∘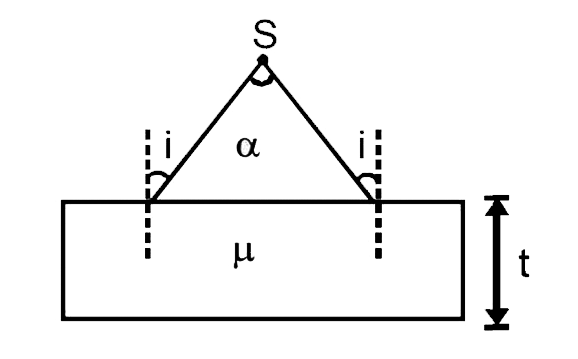
A diverging beam of light from a point source S having devergence angle α, falls symmetrically on a glass slab as shown. The angles of incidence of the two extreme rays are equal. If the thickness of the glass slab is t and the refractive index n, then the divergence angle of the emergent beam isAzeroBαCsin−1(1n)D2sin−1(1n)
Prove that the angle of emergence and angle of incidence are equal in case of refraction through a glass slab
View SolutionWhen the angle of incidence is 60∘ on the surface of a glass slab, it is found that the reflected ray is completely polarised. The velocity of light in glass is
View SolutionA diverging beam of light from a point source S having divergence angle α, falls symmetrically on a glass slab as shown. The angles of incidence of the two extrem rays are equal. If the thickness of the glass slab is t and the refractive index n, then the divergence angle of the emergent beam is
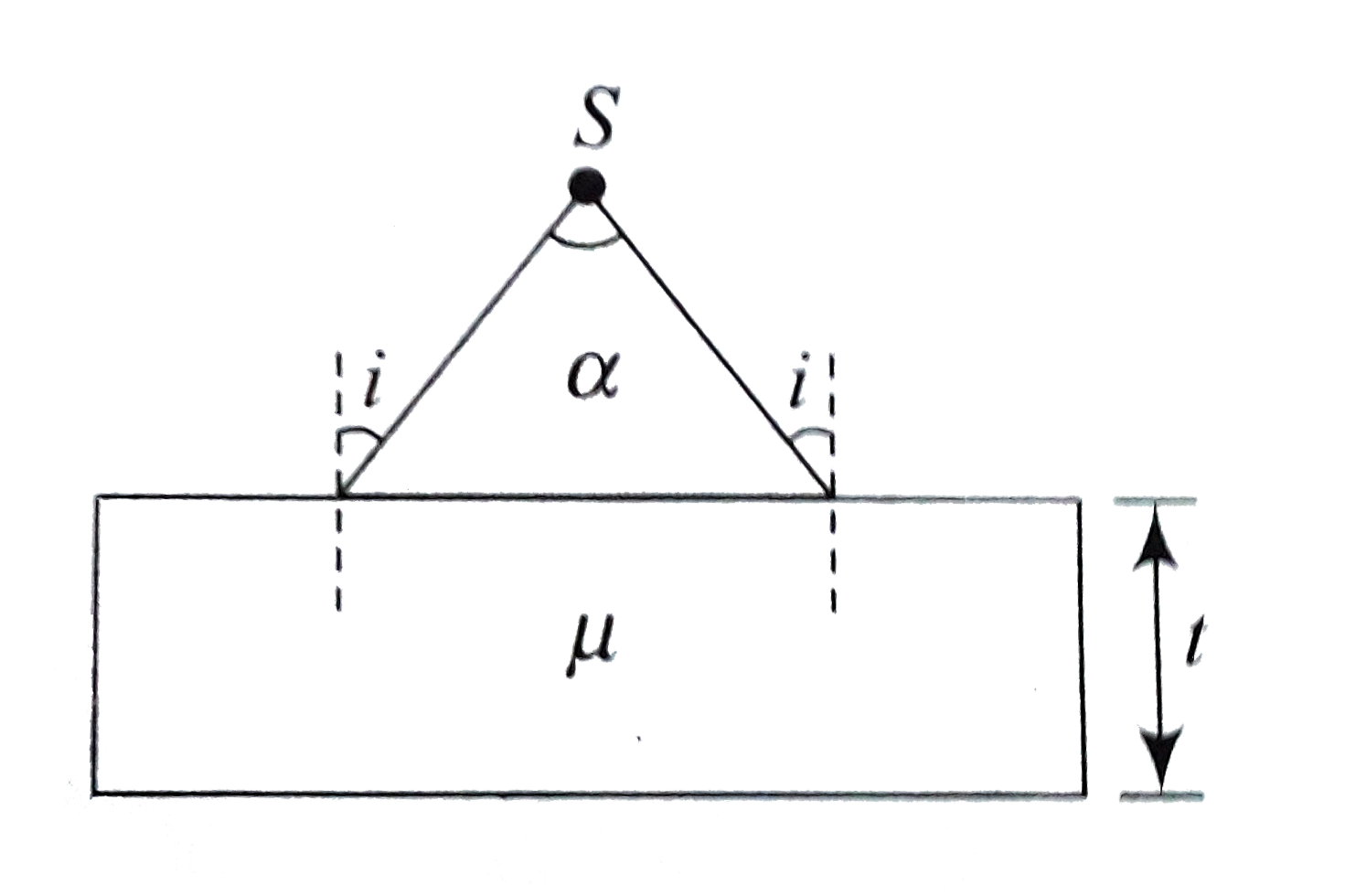
View SolutionIn the glass slab experiment, a student measures the angle of incidence (∠i) in air and finds it to be 40∘. He also measured the corresponding angle of refraction as well as the angle of emergence for this ray of light but also their record. The most likely record of readings for the angle of incidence and angle of emergence wil be :
View SolutionA student performs the experiment on tracing the path of a ray of light passing through a rectangular glass slab for different angles of incidence. He measures the angle of incidence ∠i, angle of refraction ∠r and angle of emergence ∠e for all his observations. He would find that in all cases :
View Solution