If P is the circumcentre of an acute angled triangle ABC with circumredius R. D is the midpoint of BC. Show that the perimetre of △ABC=2R(sinA+sinB+sinC).
Video Solution
|
Answer
Step by step video & image solution for If P is the circumcentre of an acute angled triangle ABC with circumredius R. D is the midpoint of BC. Show that the perimetre of triangleABC = 2R(sinA + sinB + sinC). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
ABC is right angled triangle, right angled at C . D is the midpoint of BC. Then , tanθtanϕ =
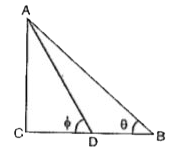 A1B1√2C12D2
A1B1√2C12D2Let ABC be an acute-angled triangle and let D be the midpoint of BC. If AB = AD, then tan(B)/tan(C) equals
A√2B√3C2D3The area of △ABC is 88cm2. If D is the midpoint of BC and E is the midpoint of AB, then the area (in cm2) of △BDE is:
A44B22C11D5.5
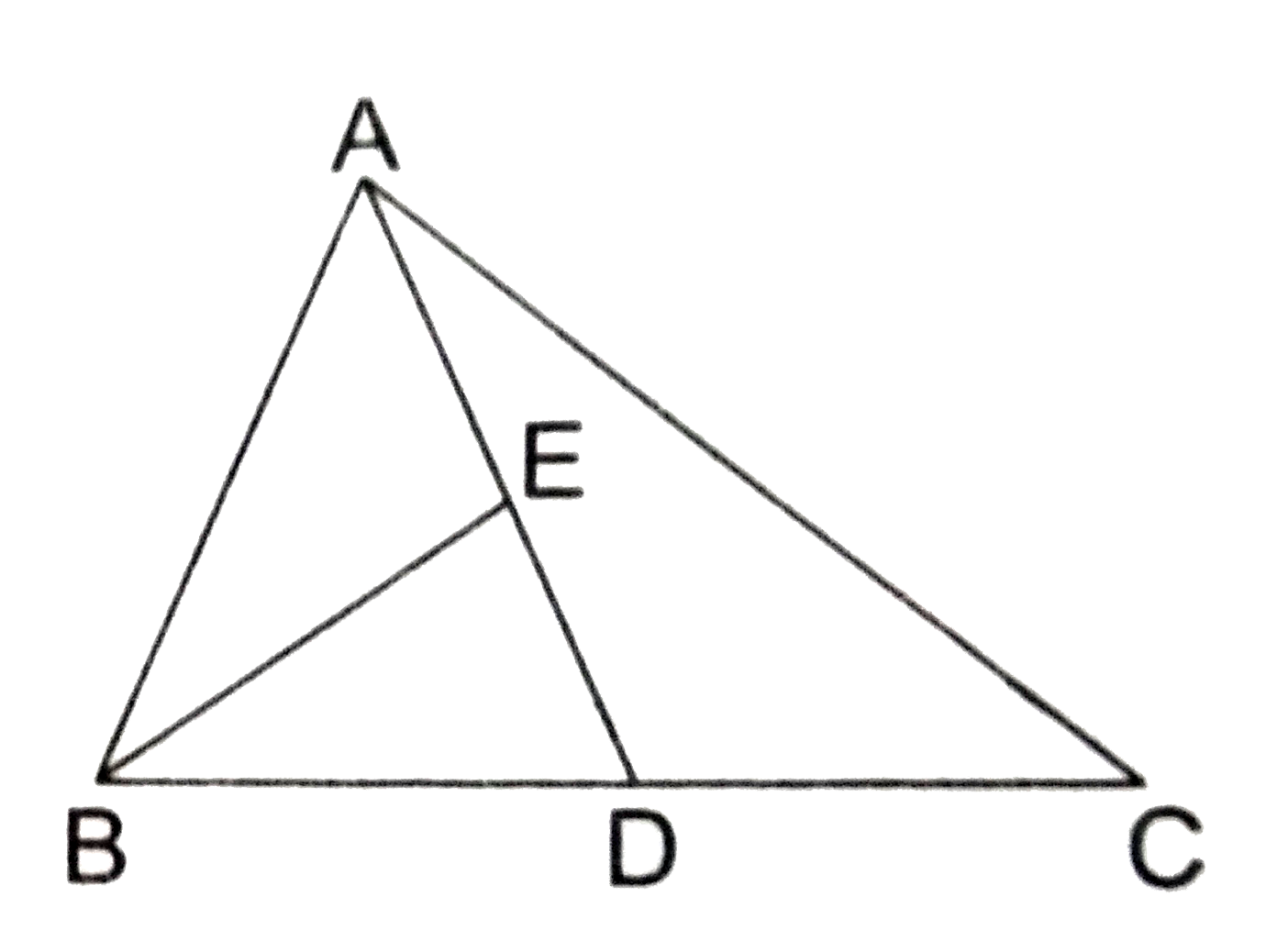
ABC is a triangle in which D is the midpoint of BC and E is the midpoint of AD.
Prove that ar(△BED)=14ar(△ABC).
View SolutionIn ΔABC if r1=2r2=3r3 and D is the mid point of BC then cos∠ADC=
View SolutionIf the lengths of the sides of a right- angled triangle ABC, right angled at C, are in arithmetic progression, then the value of 5(sinA+sinB) is
View SolutionIn any triangle ABC, if cosA=sinB2sinC, then :
View SolutionIn any triangle ABC, if cosA=sinB2sinC, then :
View Solution
