In the following four situations charged particles are equal distance from the origin. Arrange them the magnitude of the net electric field at origin greatest first
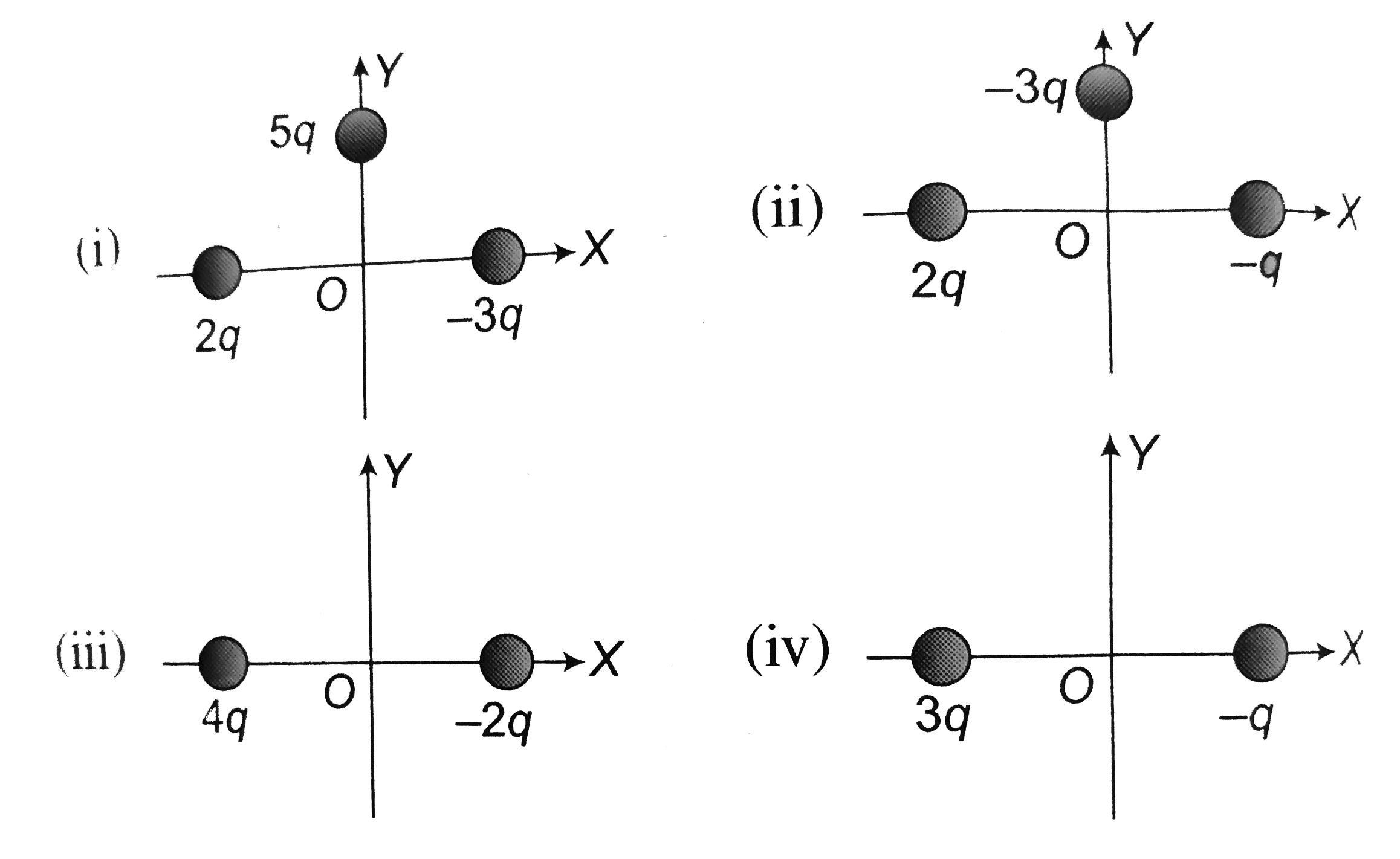
(i)>(ii)>(iii)>(iv)
(ii)>(i)>(iii)>(iv)
(i)>(iii)>(ii)>(iv)
(iv)>(iii)>(ii)>(i)
The correct Answer is:c
If electric field due to charge |q| at origin is E then field due to charges |2q|,|3q|,|4q| and |5q| are respectively 2E,3E,4E and 5E
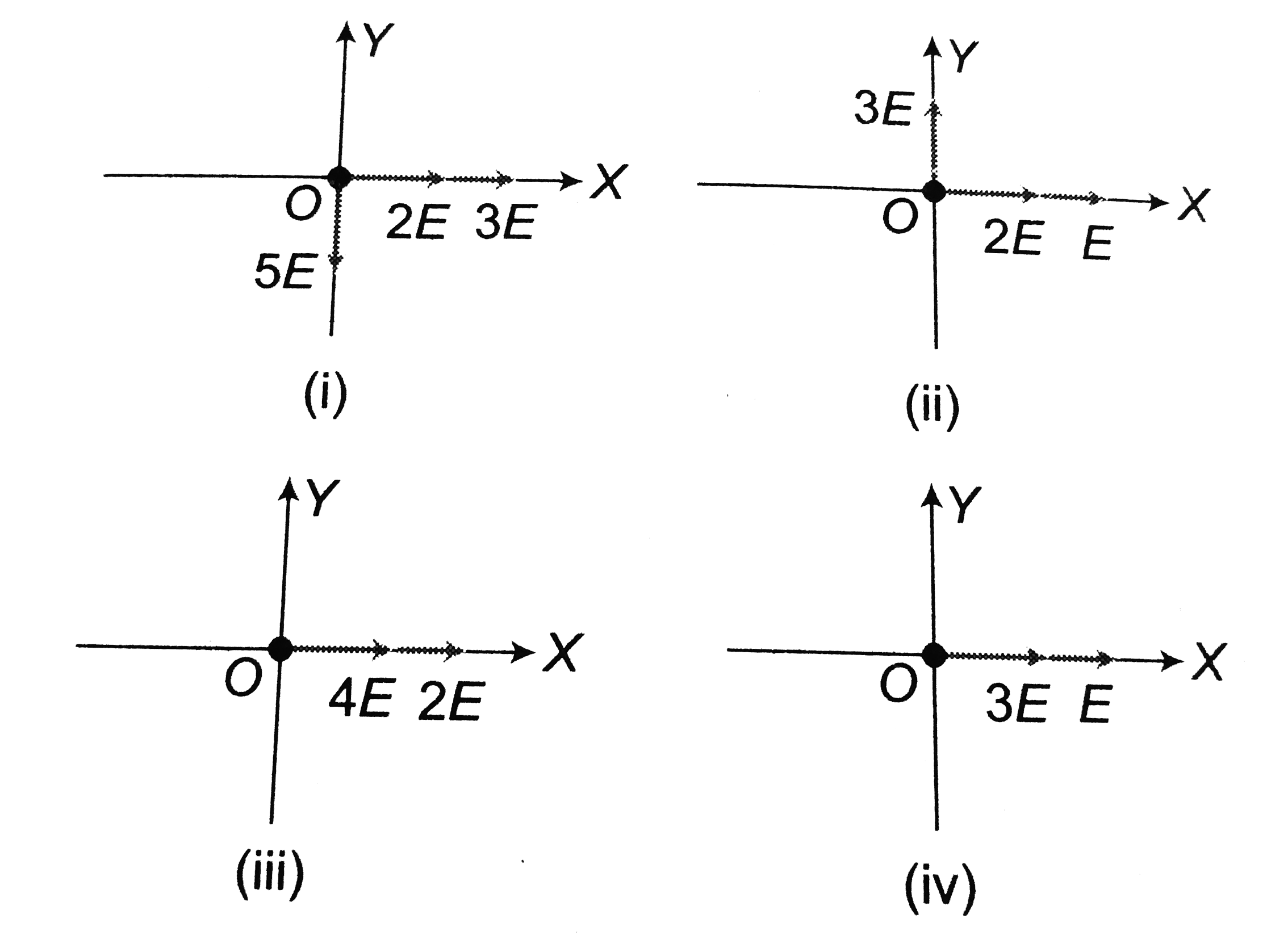
E(i)=√(5E)2+(5E)2=5√2E,
E(ii)=√(3E)2+(3E)2=3√2E,
E(iii)=4E+2E=6E and E(iv)=3E+E=4E
⇒E(i)>E(iii)>E(ii)>E(iv)
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Electric Field And Motion37videos
ELECTRIC CHARGE, FIELD & FLUX
- BOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Electric Field And Gauss'S Law25videos
ELECTRIC CHARGE, FIELD & FLUX
- BOOK - A2ZCHAPTER - ELECTRIC CHARGE, FIELD & FLUXEXERCISE - Section D - Chapter End Test29videos
ELECTRIC CHARGE, FIELD & FLUX
- BOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section D - Chapter End Test30videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - A2ZCHAPTER - ELECTRIC POTENTIAL & CAPACITANCEEXERCISE - Section D - Chapter End Test29videos
ELECTRIC POTENTIAL & CAPACITANCE
Similar Questions
In fig. a plastic rod in the form of circular are with charge +Q unformly distributed on it produces an electric fieldof magnitude E at the centre of curvature (at the origin). In fig., more circular rods with identical umform charges +Q are addes until the circle is complete. A fifth arrangement (which would be labeled e) is like taht in d except that the roed in the fourth quadrant has charge-Q. Rank all the five arrangements according to the magnitudes of the electric field at the center of curvature, greatest first.
 View Solution
View SolutionThe volume charge density of a sphere carrying charge Q and of radius R is proportional to the square of the distance from the centre. The ratio of the magnitude of the electric field at a distance 2R from the centre to that at a distance of R2 from the centre is
View Solution
Knowledge Check
- Question 1 - Select One
The figure show four situations in which charged particles are at equal distances from the origin. If E1,E2,E3andE4 be the magnitude of the net electic field at the origin in four situations i, ii, iii and iv respectively then
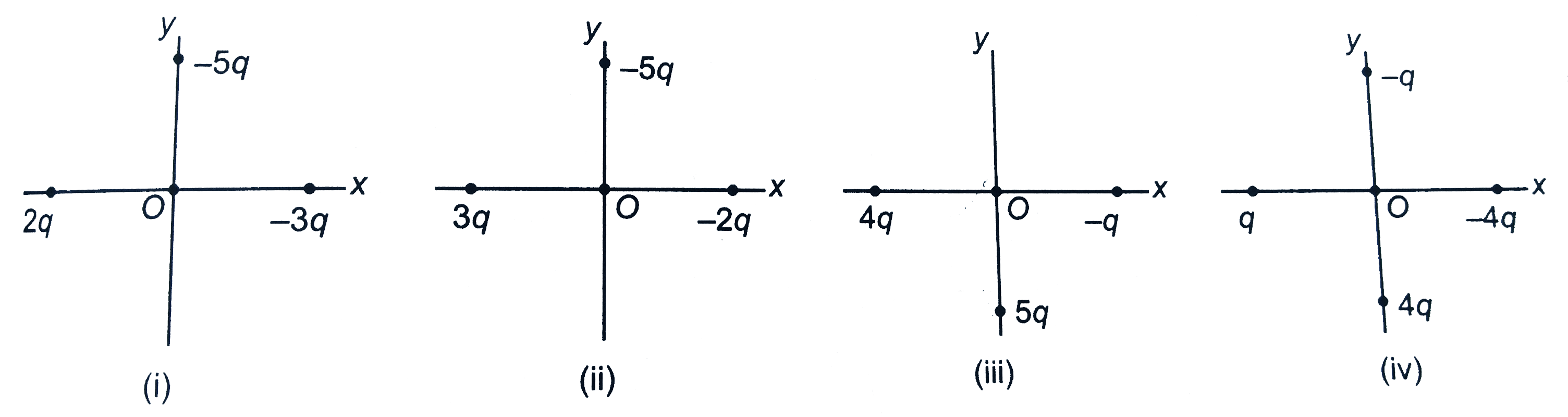 AE1=E2=E3=E+4BE1=E2>E3>E4CE1<E2<E3=E4DE1>E2=E3>E4
AE1=E2=E3=E+4BE1=E2>E3>E4CE1<E2<E3=E4DE1>E2=E3>E4 - Question 2 - Select One
A sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
A1B2C4D8 - Question 3 - Select One
All the particles of a body situated at distance d from the origin. The distance of the center of mass of the body from the origin is
A=dB≤dC>dD≥d
The electric field due to a point charge at a distance 6 m from it is 630 N/C. The magnitude of the charge is
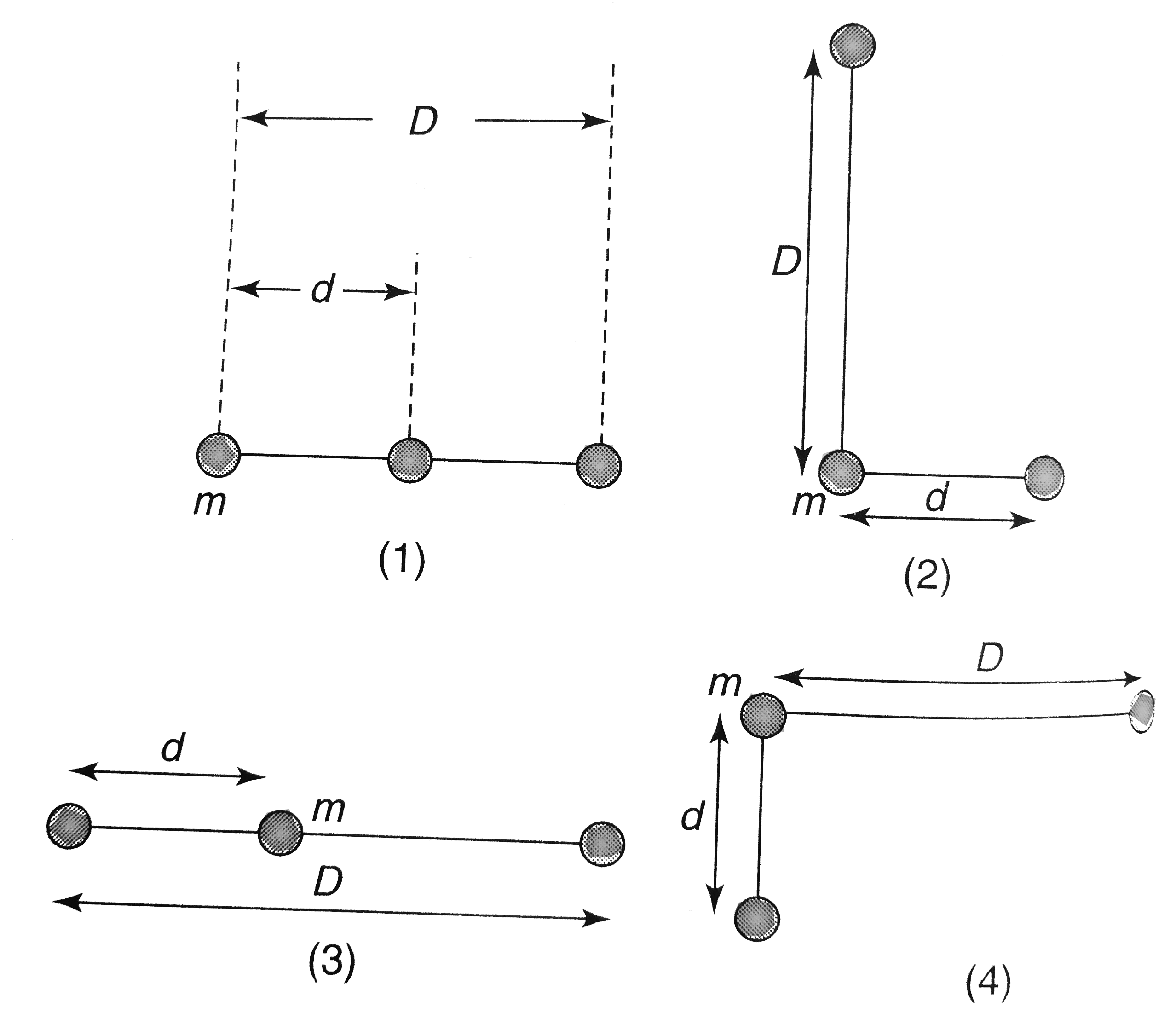
View SolutionThe figure shows four arrangement of three particles of equal masses. Rank the arrangement according to the magnitude of the net gravitational force on the particle m, greatest first.
 View Solution
View SolutionAll the particles of a body are situated at a distance R from the origin. The distance of the centre of mass of the body from the origin is
View SolutionA charge q is divided into three equal parts and placed symmetrically on a circle of radius r. The same charge is divided into four equal parts and placed symmetrically on the same circle. The electric field intensities at the centre of the circle in two situations are zero
Q. If a charge (part charge) is removed from one location in both the situations, the ratio of magnitudes of the electric field intensities at the centre isView SolutionThe figure shows four arrangements of three particles of equal masses. (a) Rank the arrangements accroding to the magnitude of the net gravitational force on the particlelabeled m, greatest first. (b) In arrangement 2, is the direaction of the net force closer of the line of length d or to theline of length D ?
View Solution

