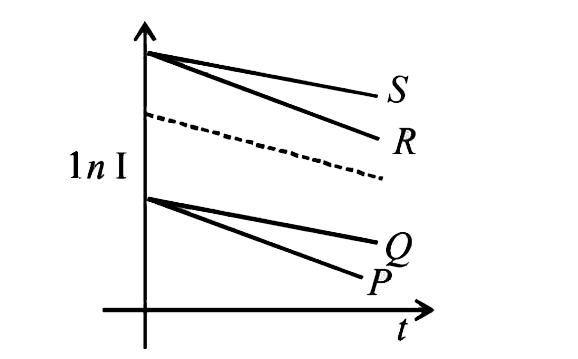
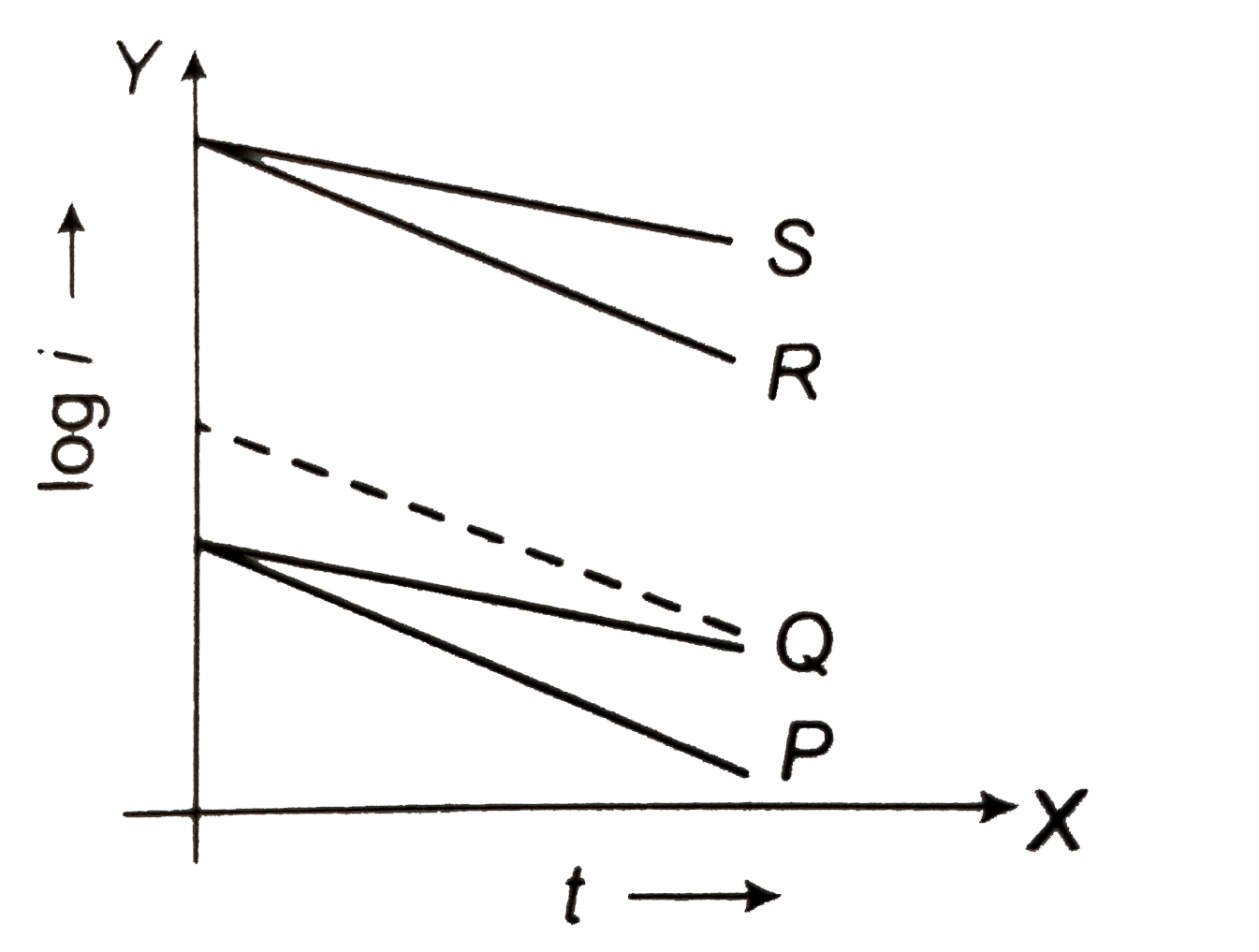
A capacitor is charged using an external battery with a resistance x in series. The dashed line showns the variation of In I with respect to time. If the resistance is changed to 2x, the new graph will be
P
Q
R
S
The correct Answer is:B
(b) I=εRe−t/RC
logeI=loge(ε)R−tRClogee⇒1nI=1n(εR)=tRC
Slope =−tRCy intercept =1n(εlor)
If resistance is made doubled, both of the above decrease in magnetude.
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Ammeter And Voltmeter
41 Videos
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Potentiometer And Meter Bridge
45 Videos
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Kircoff'S Laws And Simple Circuits
64 Videos
- ATOMIC PHYSICSBOOK - A2ZCHAPTER - ATOMIC PHYSICSEXERCISE - Section D - Chapter End Test
30 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
Two capacitors of capacitance 2μF and 3μF respectively are charged to potential difference 20 V and 40 V respectively. Now the capacitors are connected in series with a resistance such that the positively charged plate of one capacitor is connected to the positively charged plate of the other. The initial current through the resistance is I0. The potential difference across the 2μF capacitor at the instant the current has reduced to I02 is_______ V.
View SolutionA capacitor of capacity 1μF is connected in a closed series circuit with a resistance or 107 ohms, an open key and a cell of 2 V with negligible internal resistance :
(i) When the key is switched on at time t=0, find,
(a) The time constant for the circuit.
(b) The charge on the capacitor at steady state.
(c) Time taken to deposit charge equal to half of charge that will deposit at steady state.
(ii) If after completely charging the capacitor, the cell is shorted by zero resistance at time t=0, find the charge on the capacitor at t=50 s. (Given : e−5=6.73×10−3,ln2=0.693)
View Solution
Knowledge Check
The equivalent resistance of a series combination of two resistances is X ohm. If the resistance are of 10 ohm and 40 ohm respectively, the value of X will be
A10 ohmB20 ohmC50 ohmD40 ohmA capacitor of capacitance 200μF is connected to a battery through a resistance of 5kΩ at time t = 0. Charge stored in the capacitor in first second in larger than the charge stored in the next
A1sB10sC100 sD1,000 sA capacitor C is connected to the two equal resistances as shown in fig. What is the ratio of the time constant during charging and discharging of the capacitance?
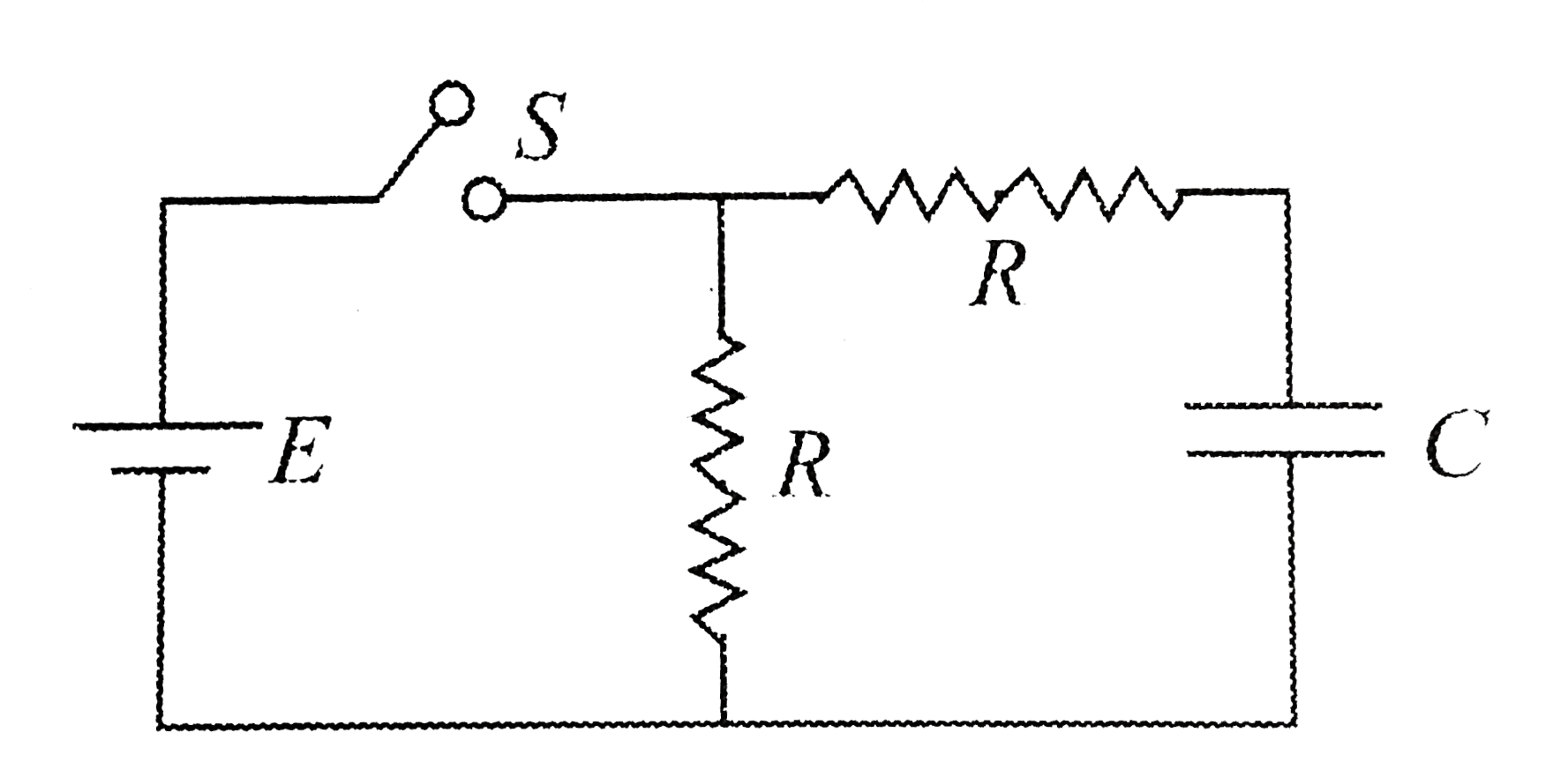A1:1B2:1C1:2D4:1
The equivalent resistances of a series combination of two resistances is XΩ. If the resistances are of 10Ωand40Ω respectively. The value of X will be:
View SolutionIf an uncharged capacitor is charged by connecting it with an ideal battery and a resistance R as shown. Then heat generated in resistor R by the time capacitor stores energy 18CV2 is :
View SolutionIn an RC circuit while charging, the graph of 1ni versis time is as shown by the dotted line in the diagram figure. Where i is the current. When the value of the resistance is doubled, which of the solid curve best represents the variation of 1ni versus time
View SolutionAn initially uncharged capacitor C is being charged by a battery of emf E through a resistance R upto the instant when the capacitor is charged to the potential E/2, the ratio of the work done by the battery to the heat dissipated by the resistor is given by :-
View SolutionA 2μF capacitor that was initially uncharged is connected to a battery of EMF 100V and a resistance and the switch is closed. The heat generated in the resistance until the capacitor becomes fully charged is (in mJ):
View Solution