Two rod A and B of the same material and dame length have radii r1andr2 respectively. When they are rigidly fixed at one end and twisted by the same couple applied at the other end, find the ratio of the angles of twist at the ends of A and B.
If a wire of length l, and radius r is fixed at one end and a torque τ is applied at the other end produces angle of twist θ in the wire, then
τ=πGr4θ2lorθ=2τlπGr4
i.e., θ∝1r4soθ1θ2=r42r41.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Long Answer questions33videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Solved Examples159videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Very short answer questions163videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance18videos
PHYSICAL WORLD AND MEASUREMENT
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)25videos
RAY OPTICS
Similar Questions
Two wires of same material and same length have radii r1andr2 respectively. Compare their resistances.
View SolutionTwo cylinders A and B of the same matieral have same length, their radii being in the ratio of 1:2 repectively. They are joined end to end as shown in figure. The upper end of A is rigidly fixed. The lower end of B is twised through an angle θ, the angle of twist of the cylinder A is:
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
Two rods A and B of the same material and length have radii r1 and r2respective. When they are rigidly fixed at one end and twisted by the same torque applied at the other end, the ratio [the angle of twist at the end of Athe angle of twist at the end of B] equal to
Ar21r22Br31r32Cr42r41Dr41r42 - Question 2 - Select One
Two wires A and B of same length and of the same material have the respective radii r1 and r2. Their one end is fixed with a rigid support, and at other end equal twisting couple is applied. Then the ratio of the angle of twist at the end of A and the angle of twist at the end of B will be
Ar21r22Br22r22Cr42r41Dr41r42 - Question 3 - Select One
Two wires of same material and length have the radii of their cross sections as r and 2r, respectively. The ratio of their resistances
A2:1B4:1C1:4D3:2
Two wires of same material and length have the radii of their cross sections as r and 2r, respectively. The ratio of their resistances
View SolutionTwo vibrating strings of the same material but lengths L and 2L have radii 2r and r respectively. They are stretched under the same tension. Both the strings vibrate in their fundamental modes, the one of length L with frequency n1 and the other with frequency n2 the ratio n1/n2 is given by
View SolutionTwo vibrating strings of the same material but lengths L and 2L have radii 2r and r respectively. They are stretched under the same tension. Both the string vibrate in their fundamental nodes, the one of length L with freuqency v1 and the other with frequency v2.theraiov_(1)//v_(2) is given by
View SolutionTwo pieces of wire, A and B of the same material have their lengths in the ratio 1:3 and their diameters are in the ratio 2:1 . If they are stretched by the same force, their elongations will be in the ratio
View SolutionTwo wires of the same material (Young's modulus=Y) and same length L but radii R and 2R respectively are joined end to end and a weight w is suspended from the combination as shown in the figure. The elastic potential energy in the system is
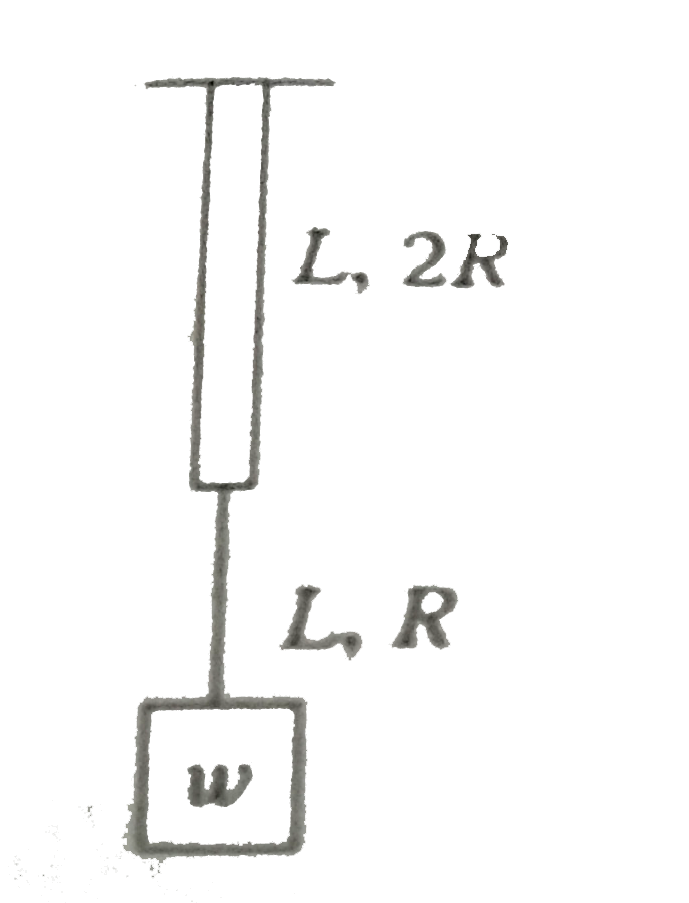 View Solution
View Solution

