Two wires of the same material (Young's modulus=Y) and same length L but radii R and 2R respectively are joined end to end and a weight w is suspended from the combination as shown in the figure. The elastic potential energy in the system is
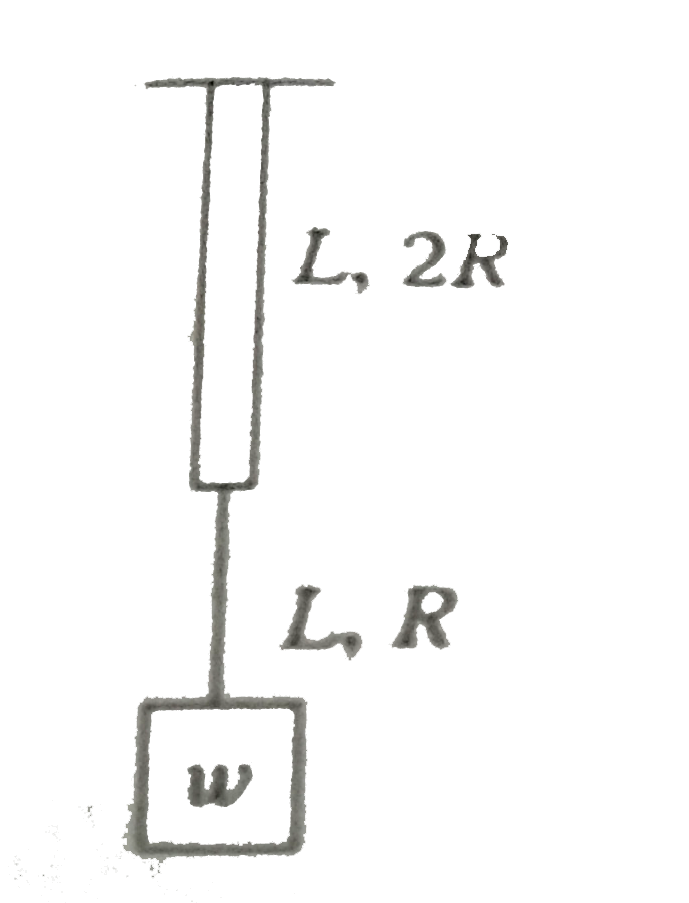
3w2L4πR2Y
3w2L8πR2Y
5w2L8πR2Y
w2LπR2Y
The correct Answer is:C
(c) We have Δl1=wL(4πR2)Y, and Δl2=wLπR2Y
The elastic potential energy in the system
∴U=12K1(Δl1)2+12K2(Δl2)2 (K=YAL)
=12×Y(πrR2)L×[wL4πR2Y]2+12×Y(πR2)L×[wLπR2Y]2
5w2L8πR2Y
Topper's Solved these Questions
- ELASTICITYBOOK - DC PANDEYCHAPTER - ELASTICITYEXERCISE - Match the columns
4 Videos
- ELASTICITYBOOK - DC PANDEYCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- ELASTICITYBOOK - DC PANDEYCHAPTER - ELASTICITYEXERCISE - Check point 12.3
15 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEYCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
434 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
Similar Questions
Two wires of same material and same length have radii r1andr2 respectively. Compare their resistances.
View SolutionTwo sphere S1andS2 made of the same material and having radii 2r and r, respectively, are immersed in water and suspended from either end of a beam as shown in figure.
The beam is in equilibrium when x:y=1:3 Determine the density of the object.

View Solution
Knowledge Check
Two wires of same material have same length but their radii are 0.1 cm and 0.2 cm. Then the value of Young's modulus is,
Ahigher for thick wireBhigher for thin wireCsame for the two wiresDdifferent for the two wiresTwo wires of same material and length have the radii of their cross sections as r and 2r, respectively. The ratio of their resistances
A2:1B4:1C1:4D3:2Two wires of same material and length have the radii of their cross sections as r and 2r, respectively. The ratio of their resistances
A2:1B4:1C1:4D3:2
Two rod A and B of the same material and dame length have radii r1andr2 respectively. When they are rigidly fixed at one end and twisted by the same couple applied at the other end, find the ratio of the angles of twist at the ends of A and B.
View SolutionTwo Steel wires of the same length but radii r and 2r are connected together end to end and tied to a wall as shown.

The force stretches the combination by 10mm. How far does the midpoint A move? (in mm)
View SolutionA block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View SolutionTwo rods A and B of the same material and length have radii r1 and r2respective. When they are rigidly fixed at one end and twisted by the same torque applied at the other end, the ratio [the angle of twist at the end of Athe angle of twist at the end of B] equal to
View SolutionTwo wires made from the same material have their lengths L and 2L and the radii 2r and r respectively. If they are stretched by the same force, their extensions are e1 and e2. The ratio e1e2 is
View Solution
