An air chamber of volume V has a neck area of cross section A into which a ball of mass m just fits and can move up and down without any friction, figure. Show that when the ball is pressed down a little and released, it executes SHM. Obtain an expression for the time periodof oscillations assuming pressure volume variatinos of air to be isothermal.
To solve the problem step by step, we will analyze the motion of the ball in the air chamber and derive the expression for the time period of oscillation when the ball is pressed down and released.
Step 1: Understand the System
We have an air chamber with a volume V and a neck with a cross-sectional area A. A ball of mass m fits into the neck and can move up and down without friction. When the ball is pressed down a distance Y and released, we need to show that it executes Simple Harmonic Motion (SHM).
Step 2: Change in Volume
When the ball is pressed down by a distance Y, the volume of air in the chamber decreases. The change in volume ΔV can be expressed as:
ΔV=A⋅Y
Step 3: Bulk Modulus and Pressure Change
The bulk modulus E of the air is defined as:
E=−PΔVV=−P⋅VΔV
Where P is the pressure. Substituting ΔV:
E=−P⋅VA⋅Y
Rearranging gives us the pressure P:
P=−E⋅A⋅YV
Step 4: Force on the Ball
The force exerted by the pressure on the ball can be calculated as:
F=P⋅A=−E⋅A⋅YV⋅A=−E⋅A2⋅YV
This force acts as the restoring force, which is directed opposite to the displacement Y.
Step 5: Establishing the SHM Condition
The restoring force can be expressed in the form:
Frestoring=−kY
where k=E⋅A2V. This shows that the restoring force is proportional to the displacement Y and acts in the opposite direction, confirming that the motion is SHM.
Step 6: Time Period of Oscillation
The time period T of SHM is given by:
T=2π√mk
Substituting k:
T=2π√mE⋅A2V
This simplifies to:
T=2π√mVE⋅A2
Final Expression
Thus, the expression for the time period of oscillation when the ball is pressed down and released is:
T=2π√mVE⋅A2
We have an air chamber with a volume V and a neck with a cross-sectional area A. A ball of mass m fits into the neck and can move up and down without friction. When the ball is pressed down a distance Y and released, we need to show that it executes Simple Harmonic Motion (SHM).
When the ball is pressed down by a distance Y, the volume of air in the chamber decreases. The change in volume ΔV can be expressed as:
ΔV=A⋅Y
The bulk modulus E of the air is defined as:
E=−PΔVV=−P⋅VΔV
Where P is the pressure. Substituting ΔV:
E=−P⋅VA⋅Y
Rearranging gives us the pressure P:
P=−E⋅A⋅YV
The force exerted by the pressure on the ball can be calculated as:
F=P⋅A=−E⋅A⋅YV⋅A=−E⋅A2⋅YV
This force acts as the restoring force, which is directed opposite to the displacement Y.
The restoring force can be expressed in the form:
Frestoring=−kY
where k=E⋅A2V. This shows that the restoring force is proportional to the displacement Y and acts in the opposite direction, confirming that the motion is SHM.
The time period T of SHM is given by:
T=2π√mk
Substituting k:
T=2π√mE⋅A2V
This simplifies to:
T=2π√mVE⋅A2
Thus, the expression for the time period of oscillation when the ball is pressed down and released is:
T=2π√mVE⋅A2
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - NCERT CHAPTER26videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Higher Order Thinking Skills13videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - NCERT EXERCISES WITH SOLUTIONS19videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks5videos
MATHEMATICAL TOOLS
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance18videos
PHYSICAL WORLD AND MEASUREMENT
Similar Questions
One end of a U-tube containing mercury is connected to a suction pump and the other end to atmosphere. A small pressure difference is maintained between the two columns. Show that, when the suction pump is removed, the column of mercury in the U-tube executes simple harmonic motion.
View SolutionAn air chamber of volume v has a neck area of cross section a into which a ball of mass m just fits and can move up and down without any friction. Show that when the ball is pressed down a little and released for the time priod of oscillation, assuming pressure-volume variations of the air to be isothermal.
View Solution
Knowledge Check
- Question 1 - Select One
An air chamber of volume V has a neck of cross-sectional area a into which a light ball of mass m just fits and can move up and down without friction. Consider diameter of the ball to be equal to that of the neck of the chamber. The ball is then pressed down a little and released. Let the bulk modulus of air is B. The time period of the oscillation of the ball is:
AT=2π√Ba2mVBT=2π√BVma2CT=2π√mBVa2DT=2π√mVBa2 - Question 2 - Select One
An air chamber of volume V has a long of cross sectional area A. A ball of mass m is fixed sympthlly in the track The ball modulus of air is B ball is pressed down slightly and released, the time period of the oscillation is
A2π√mV2BA2Bπ√2mVBA2C2π√mVBA2Dπ2√mBA2 - Question 3 - Select One or More
Figure shows initial state of an ideal gas trapped in a container with conducting walls and a piston (mass m) which can move without any friction. The container is placed on point supports and its wall are conducting. Assuming that atmospheric pressure is P0 and the mass of the ideal gas is negligible as compared to the mass of the piston and the mass of container. Take the cross section area of piston to be A. The piston is slowly lifted by an external agent and held in its position. Let M be the maximum mass of container so that it may "lift off" while pulling the piston upwards and Pi be the pressure of ideal gas in initial state. Pick the correct choice:
 APi=P0+mgABPi=P02+mg2ACM=m2DM=P0A2g−m2
APi=P0+mgABPi=P02+mg2ACM=m2DM=P0A2g−m2
An air chamber of volume V has a neck of crosssectional area "a' into which a light ball of mass "m' just fits and can move up and down without friction. The diameter of the ball is equal to that of the neck of the chamber. The ball is pressed down a little and released. If the bulk modulus of air is B the time period of the oscillation of the ball is 2πmxVyBza9. The value of xyq is
View SolutionA ball of radius r fits tightly inside a tube attached to a container. There is no friction between the tube wall and the ball. Volume of air inside the container is V0 when the ball is in equilibrium. Density of the material of the ball is d and atmospheric pressure is P0. If the ball is displaced a little from its equilibrium position and released, find time period of its oscillation. Assume that temperature in the container remains constant and that air is an ideal gas.
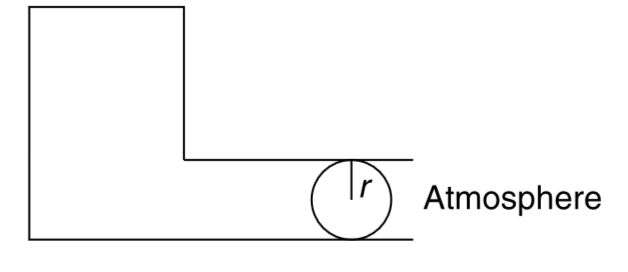 View Solution
View SolutionA hollow cylindrical shell of radius R has mass M. It is completely filled with ice having mass m. It is placed on a horizontal floor connected to a spring (force constant k) as shown. When it is disturbed it performs oscillations without slipping on the floor.
(a) Find time period of oscillation assuming that the ice is tightly pressed against the inner surface of the cylinder.
(b) If the ice melts into non viscous water, find the time period of oscillations. (Neglect any volume change due to melting of ice)
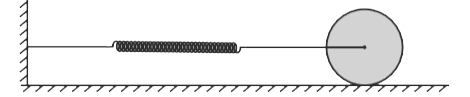 View Solution
View Solution(i) A cylindrical container has area of cross section equal to 4A and it contains a non viscous liquid of density 2ρ. A wooden cylinder of cross sectional area A and length L has density ρ. It is held vertically with its lower surface touching the liquid. It is released from this position. Assume that the depth of the container is sufficient and the cylinder does not touch the bottom.
(a) Find amplitude of oscillation of the wooden cylinder.
(b) Find time period of its oscillation.
Two cubical blocks of side length a and 2a are stuck symmetrically as shown in the figure. The combined block is floating in water with the bigger block just submerged completely. The block is pushed down a little and released. Find the time period of its oscillation. Neglect viscostiy.
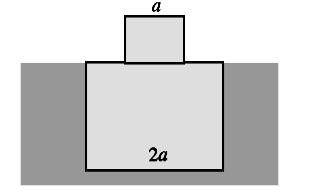 View Solution
View SolutionA tube of mass M hangs from a thread and two balls of mass m slide inside it without friction (see figure). The balls are released simultaneously from the top of the ring and slide down on opposite sides. θ defines the positions of balls at any time as shown in figure
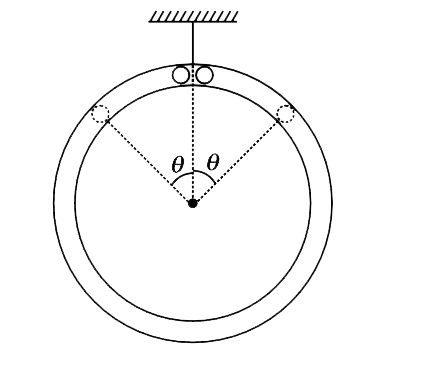
(a) Show that ring will start to rise if m≥3M2.
(b) If M=0 , find the angle θ at which the tube begins to rise.View Solution

