A spring of constant k=0.5N/m and an attached mass m oscillates on a smooth horizontal table. When the mass is at position x1=0.1m, its velocity is v1=−1ms−1 , and at x2=0.2m, it has velocity v2=0.5ms−1. Find m and the amplitude A of the motion.
The kinetic energy of body attached with spring of spring constant k at any point x is givey by total energy − potential energy = kinetic energy
i.e., 12kA2−12kx2=12mv2
where A is the amplitude of oscillation.
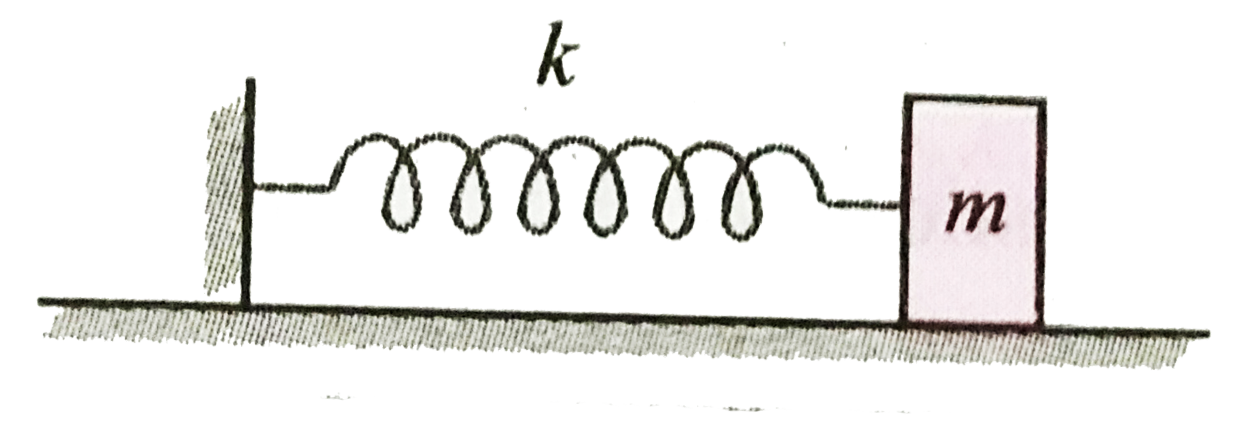
As per question,
12k(A2−x21)=12mv21
or 12kA2=12mv21+12kx21 ...(i)
and 12kA2=12mv22+12kx22 ...(ii)
From (i) and (ii),
12m(v22−v21)=192k(x21−x22)
or m(v22−v21)=k(x21−x22)
or m[(0.5)2−(−1)2]=0.5[0.1)2−(0.2)2]
or m×0.75=0.5×0.03
or m=0.5×0.030.75=0.02kg
From (i),
A=[mv21+kx21k]1/2
=[0.02(−1)2+0.5×(0.1)20.5]1/2=0.22m.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Multiple Choice Question-I21videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Multiple Choice Question-II14videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Fill In The Blanks20videos
OSCILLATIONS AND WAVES
- BOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks5videos
MATHEMATICAL TOOLS
- BOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance18videos
PHYSICAL WORLD AND MEASUREMENT
Similar Questions
A mass M attached to a spring of spring constant 0.7 N/m oscillates on a smooth horizontal table. When displacement is 0.3 m, velocity is 2 ms−1. And when displacement is 0.4 m, velocity is 0.3 m/s. Calculate the vlaue of M.
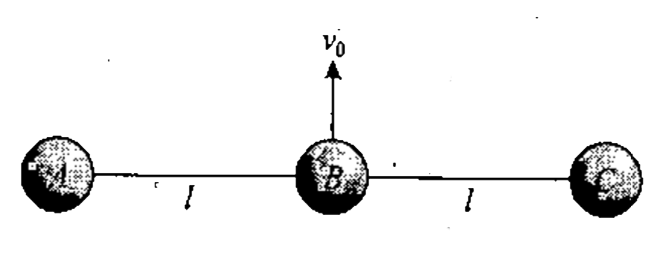
View SolutionThree identical balls eah of mass m=0.5 kg are connected with each other as shown in figure and rest over a smooth horizontal table At moment t=0, ball B is imparted a horizontal velocity v0=9ms−1 calculate velocity of A just before it collides with ball C.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A particle A of mass 10/7kg is moving in the positive direction of x−aξs. At initial position x=0, its velocity is 1ms−1, then its velocity at x=10m is (use the graph given)
 A(a) 4ms−1B(b) 2ms−1C(c) 3√2ms−1D(d) 1003ms−1
A(a) 4ms−1B(b) 2ms−1C(c) 3√2ms−1D(d) 1003ms−1 - Question 2 - Select One
Two particles of equal mass have coordinates (2m,4m,6m) and (6m,2m,8m). Of these one particle has a velocity v1=(2i)ms−1 and another particle has a velocity v2=(2j)ms−1 at time t=0. The coordinate of their center of mass at time t=1s will be
A(4m,4m,7m)B(5m,4m,7m)C(2m,4m,6m)D(4m,5m,4m) - Question 3 - Select One
If a lighter body (mass M1 and velocity V1 ) and a heavier body (mass M2 and velocity V2) have the same kinetic energy, then-
AM2V2<M1V1BM2V2=M1V1CM2V1=M1V2DM2V2>M1V1
What is the oscillation amplitude of a 4.00kg box oscillating on a spring with spring constant 100N/m if at time t= 1.00s the position is x = 0.129m and the velocity is v= 5.00m/s? At t= 0, what are (a) the position and (b) the velocity?
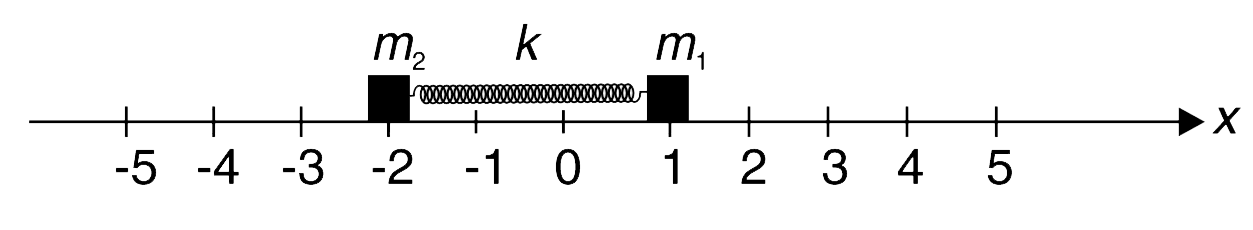
View SolutionTwo blocks of mass m1andm2 are connected to the ends of a spring. The spring is held compressed and the system is placed on a smooth horizontal table. The block of mass m1=2kg is kept at x=1cm mark and the other block is at x=2cm mark. The system is released from this position. It was observed that at the instant m1 was at x=5cm mark its velocity was zero and at that moment m2 was located at x=−4cm. Find mass m2 and unstretched length (l0) of the spring
 View Solution
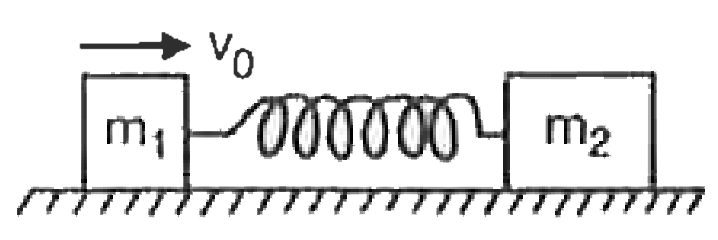
View SolutionTwo bodies with masses m1 = 1kg and m2= 2kg are connected by a spring of force constant k=24Nm−1. The left ball is imparted an initial velocity v0=12 cm/s. Find (a) the frequency of the oscillations (b) the energy and the amplitude of the osciallations.
 View Solution
View SolutionTwo balls with massesm1=1.0kg and m2=2.0kg are slipped on a thin smooth horizontal rod ( figure). The balls are interconnected by a light spring of stiffness x=24N/m. The left- hand ball is imparted the initial velocity v1=12cm/s. Find :
(a) the oscillation frequency of the system in the process of motion,
(b) the energy and the amplitude of oscillations
 View Solution
View SolutionIf a lighter body (mass M1 and velocity V1 ) and a heavier body (mass M2 and velocity V2) have the same kinetic energy, then-
View Solution

