A ray of light is inclined to one face of a prism at an angle of 50∘. The angle of prism is 60∘ and the ray deviates through an angle which the emergent ray makes with second face of the prism.
The correct Answer is:28∘
To solve the problem step by step, we will follow these steps:
Step 1: Understand the given information
- The angle of incidence (i) with the first face of the prism is given as 50∘.
- The angle of the prism (A) is 60∘.
- The angle of deviation (δ) is 42∘.
Step 2: Calculate the angle of incidence (i)
The angle of incidence (i) is measured from the normal to the surface. Since the ray is inclined at 50∘ to the face of the prism, we can find the angle of incidence using the relation:
i=90∘−50∘=40∘
Step 3: Use the formula for angle of deviation
The angle of deviation (δ) can be expressed using the formula:
δ=i+e−A
Where:
- e is the angle of emergence,
- A is the angle of the prism.
Substituting the known values into the equation:
42∘=40∘+e−60∘
Step 4: Solve for the angle of emergence (e)
Rearranging the equation to solve for e:
42∘=40∘+e−60∘
42∘=e−20∘
e=42∘+20∘=62∘
Step 5: Calculate the angle the emergent ray makes with the second face of the prism
The angle that the emergent ray makes with the second face of the prism (θ) can be found using:
θ=90∘−e
Substituting the value of e:
θ=90∘−62∘=28∘
Final Answer
The emergent ray makes an angle of 28∘ with the second face of the prism.
---
- The angle of incidence (i) with the first face of the prism is given as 50∘.
- The angle of the prism (A) is 60∘.
- The angle of deviation (δ) is 42∘.
The angle of incidence (i) is measured from the normal to the surface. Since the ray is inclined at 50∘ to the face of the prism, we can find the angle of incidence using the relation:
i=90∘−50∘=40∘
The angle of deviation (δ) can be expressed using the formula:
δ=i+e−A
Where:
- e is the angle of emergence,
- A is the angle of the prism.
42∘=40∘+e−60∘
Rearranging the equation to solve for e:
42∘=40∘+e−60∘
42∘=e−20∘
e=42∘+20∘=62∘
The angle that the emergent ray makes with the second face of the prism (θ) can be found using:
θ=90∘−e
Substituting the value of e:
θ=90∘−62∘=28∘
The emergent ray makes an angle of 28∘ with the second face of the prism.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(d)35videos
RAY OPTICS
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - (Test Your Grip)Multiple Choice (b)10videos
RAY OPTICS
- BOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(b)66videos
RAY OPTICS
- BOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Multiple choice questions7videos
PROPERTIES OF BULK MATTER
- BOOK - PRADEEPCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assertion- Reason Type questions20videos
SYSTEMS OF PARTICLES AND ROTATIONAL MOTION
Similar Questions
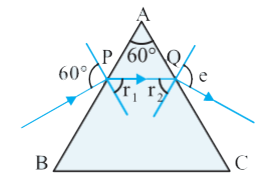
A ray of light is inclined to one face of a prism at an angle of 60∘. If angle of prism is 60∘ and the ray deviated through an angle of 42∘ find the angle which the emergent ray makes with second face of the prism.
View SolutionA ray of light is incident on one face of a prism (m=√3) at an angle of 60∘. The refracting angle of the prism is also 60∘. Find the angle of emergence .
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A ray of light is incident at an angle of 50∘ on one face of an equilateral prism . What is the angle ,which the emergent ray makes with the second face of the prism , if the deviation produced by the prism is 42∘ ?
A48∘B38∘C58∘D42∘ - Question 2 - Select One
A ray of light is incident on one face of a prism at an angle of 50∘ with the normal . The ray is deviated by the prism through 42∘ . What is the angle of the prism , if the angle of emergence is 51∘ ?
A56∘B57∘C58∘D59∘ - Question 3 - Select One
A ray of light is incident on one of the faces of the angle prism with an angle 40∘ with the surface. The angle of the prism is 60∘. The emergent ray is deviated through an angle 38∘. The angle of emergence is
A38∘B48∘C52∘D58∘
A ray of light is incident on one face of a prism (μ=1.5) at an angle of 60∘. The refracting angle of the prism is also 60∘. Find the angle of emergence and the angle of deviation. Is there any other angle of incidence, which will produce the same deviation?
View SolutionA ray of light is incident on one face of prism making an angle of 50∘ with the face. If emergent ray from the prism is made to retrace its path, what would be the angle of emergence ?
View SolutionA ray of light falls on one face of an equilateral prism at an angle of 40∘ and emerges out at an angole of 37∘ What is the angle of deviation through the prism ?
View SolutionA ray of light takes grazing incidence on the first face of a prism having critical angle C. Find the angle of prism is after refraction ray does not emerge out.
View SolutionA ray of light is incident at an angle of 60∘ on one face of a prism of angle 30∘. The ray emerging out of the prism makes an angle of 30∘ with the incident ray. The emergent ray is
View Solution

