Question

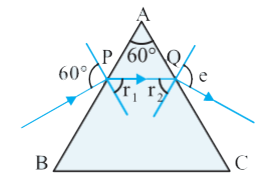
A ray of light is incident on one face of a prism $(\mu =1.5)$ at an angle of ${60}^{\circ }$. The refracting angle of the prism is also ${60}^{\circ }$. Find the angle of emergence and the angle of deviation. Is there any other angle of incidence, which will produce the same deviation?

Explanation

To solve the problem step by step, we will use Snell's law and the properties of the prism.

Step 1: Identify the given values
- Refractive index of the prism, \( \mu = 1.5 \)
- Angle of incidence, \( i = 60^\circ \)
- Refracting angle of the prism, \( A = 60^\circ \)

Step 2: Apply Snell's Law at the first surface
Using Snell's law at the first interface (air to prism):
\( \mu_1 \sin i = \mu_2 \sin r_1 \)
Where:
- \( \mu_1 = 1 \) (refractive index of air)
- \( \mu_2 = 1.5 \) (refractive index of prism)
- \( r_1 \) is the angle of refraction at the first surface.

Substituting the known values:
\( 1 \cdot \sin(60^\circ) = 1.5 \cdot \sin(r_1) \)
\( \sin(r_1) = \frac{\sin(60^\circ)}{1.5} = \frac{\sqrt{3}/2}{1.5} = \frac{\sqrt{3}}{3} = \sin(35^\circ) \)
Thus,
\( r_1 = 35^\circ \)

Step 3: Calculate the second angle of refraction \( r_2 \)
Using the property of the prism:
\( r_1 + r_2 = A \)
Substituting the known values:
\( 35^\circ + r_2 = 60^\circ \)
Thus,
\( r_2 = 60^\circ - 35^\circ = 25^\circ \)

Step 4: Apply Snell's Law at the second surface
Using Snell's law at the second interface (prism to air):
\( \mu_2 \sin r_2 = \mu_1 \sin e \)
Where \( e \) is the angle of emergence. Substituting the known values:
\( 1.5 \cdot \sin(25^\circ) = 1 \cdot \sin(e) \)
Calculating \( \sin(25^\circ) \):
\( \sin(25^\circ) \approx 0.4226 \)
Thus,
\( \sin(e) = 1.5 \cdot 0.4226 \approx 0.6339 \)
Calculating \( e \):
\( e \approx \arcsin(0.6339) \approx 39^\circ \)

Step 5: Calculate the angle of deviation \( D \)
The angle of deviation \( D \) is given by:
\( D = i + e - A \)
Substituting the known values:
\( D = 60^\circ + 39^\circ - 60^\circ = 39^\circ \)

Step 6: Check for any other angle of incidence that produces the same deviation
To find another angle of incidence that produces the same deviation, we can switch the values of the angle of incidence and the angle of emergence. Thus, if \( i = e \) and \( e = i \), we can check if it leads to the same deviation.

Final Answers:
- Angle of emergence \( e = 39^\circ \)
- Angle of deviation \( D = 39^\circ \)
- Yes, there is another angle of incidence which will produce the same deviation by switching the angles.