A wire AB is carrying a steady current of 12A and is lying on the table. Another wire CD carrying current 5A is held vertically above AB at a height of 1mm. Find the mass per unit length of the wire CD so that it remains suspended at the position when left free. Give the direction of the current flowing in CD with respect to that in AB. g=10ms−2.
To solve the problem, we need to find the mass per unit length of wire CD so that it remains suspended when left free. We will also determine the direction of the current in wire CD with respect to wire AB.
1. Identify the Given Values:
- Current in wire AB, I1=12A
- Current in wire CD, I2=5A
- Height between the wires, R=1mm=1×10−3m
- Acceleration due to gravity, g=10m/s2
2. Determine the Force per Unit Length Between the Wires:
The formula for the force per unit length F/L between two parallel wires carrying currents I1 and I2 is given by:
FL=μ0I1I22πR
where μ0 (the permeability of free space) is approximately 4π×10−7T m/A.
3. Set Up the Equation for Equilibrium:
For wire CD to remain suspended, the upward magnetic force must equal the downward gravitational force. Thus, we have:
FL=mg
where m is the mass per unit length of wire CD.
4. Substitute the Values into the Equation:
Equating the two forces gives:
μ0I1I22πR=mg
Rearranging for m:
m=μ0I1I22πRg
5. Plug in the Known Values:
Substitute μ0=4π×10−7T m/A, I1=12A, I2=5A, R=1×10−3m, and g=10m/s2:
m=(4π×10−7)(12)(5)2π(1×10−3)(10)
6. Simplify the Expression:
The π terms cancel out:
m=(4×10−7)(12)(5)2(1×10−3)(10)
=240×10−720×10−3=240×10−720×10−3=24020×10−4=12×10−4=1.2×10−3kg/m
7. Final Result:
The mass per unit length of wire CD is:
m=1.2grams/meter
8. Determine the Direction of Current in CD:
Since the currents in the two wires must be in opposite directions for the magnetic force to be attractive (and hence allow wire CD to be suspended), the direction of the current in wire CD must be opposite to that in wire AB.
Summary:
- The mass per unit length of wire CD is 1.2grams/m.
- The direction of the current in wire CD is opposite to that in wire AB.
- Current in wire AB, I1=12A
- Current in wire CD, I2=5A
- Height between the wires, R=1mm=1×10−3m
- Acceleration due to gravity, g=10m/s2
The formula for the force per unit length F/L between two parallel wires carrying currents I1 and I2 is given by:
FL=μ0I1I22πR
where μ0 (the permeability of free space) is approximately 4π×10−7T m/A.
For wire CD to remain suspended, the upward magnetic force must equal the downward gravitational force. Thus, we have:
FL=mg
where m is the mass per unit length of wire CD.
Equating the two forces gives:
μ0I1I22πR=mg
Rearranging for m:
m=μ0I1I22πRg
Substitute μ0=4π×10−7T m/A, I1=12A, I2=5A, R=1×10−3m, and g=10m/s2:
m=(4π×10−7)(12)(5)2π(1×10−3)(10)
The π terms cancel out:
m=(4×10−7)(12)(5)2(1×10−3)(10)
=240×10−720×10−3=240×10−720×10−3=24020×10−4=12×10−4=1.2×10−3kg/m
The mass per unit length of wire CD is:
m=1.2grams/meter
Since the currents in the two wires must be in opposite directions for the magnetic force to be attractive (and hence allow wire CD to be suspended), the direction of the current in wire CD must be opposite to that in wire AB.
- The mass per unit length of wire CD is 1.2grams/m.
- The direction of the current in wire CD is opposite to that in wire AB.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Conceptual Problems (d)2videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Very Short Answer Questions (a)2videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - ASSERTION-REASON TYPE QUESTIONS2videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - OPTICSEXERCISE - Multiple choice questions1videos
OPTICS
Similar Questions
A wire AB is carrying a steady current of 12 A and is lying on the table. Another wire CD carrying 5 A is held directly above AB at a height of 1mm. Find the mass per unit length of the wire CD so that it remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that in AB. [Take the value of g=10ms−2]
View SolutionA write AB is carrying a steady current of 10A and is lying on the table. Another wire CD carrying 6A is held directly above AB at a height of 2 mm. Find the mass per unit length of the wire CD so that it remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that in AB. [Take the value of g=10ms−2]
View Solution
Knowledge Check
- Question 1 - Select One
The resistance per unit length of the wires in segments AB, BC, CD, and AD are the same. The magnetic field due to the current at point O is
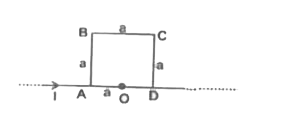 A3μ0l4√5πaB3μ0l√5πaCμ0l2√5πaDnone of the above
A3μ0l4√5πaB3μ0l√5πaCμ0l2√5πaDnone of the above - Question 2 - Select One
In the figure shown a current I1 is estabilshed in the log straight wire AB. Another wire CD carrying current I2 is placed in the plane of the paper. The line joining the ends of this wire is perpendicular to the wire AB. The resultant force on the wire CD is:
 AZeroBtowards negative x-axisCtowards positive y-axisDnone of these
AZeroBtowards negative x-axisCtowards positive y-axisDnone of these - Question 3 - Select One
In the figure shown a current I1 is established in the long straight wire AB. Another wire CD carrying currrent I2 is placed in the plane of the paper. The line joining the ends of this wire is perpendicular to the wire AB. The resultant force on the wire CD is :
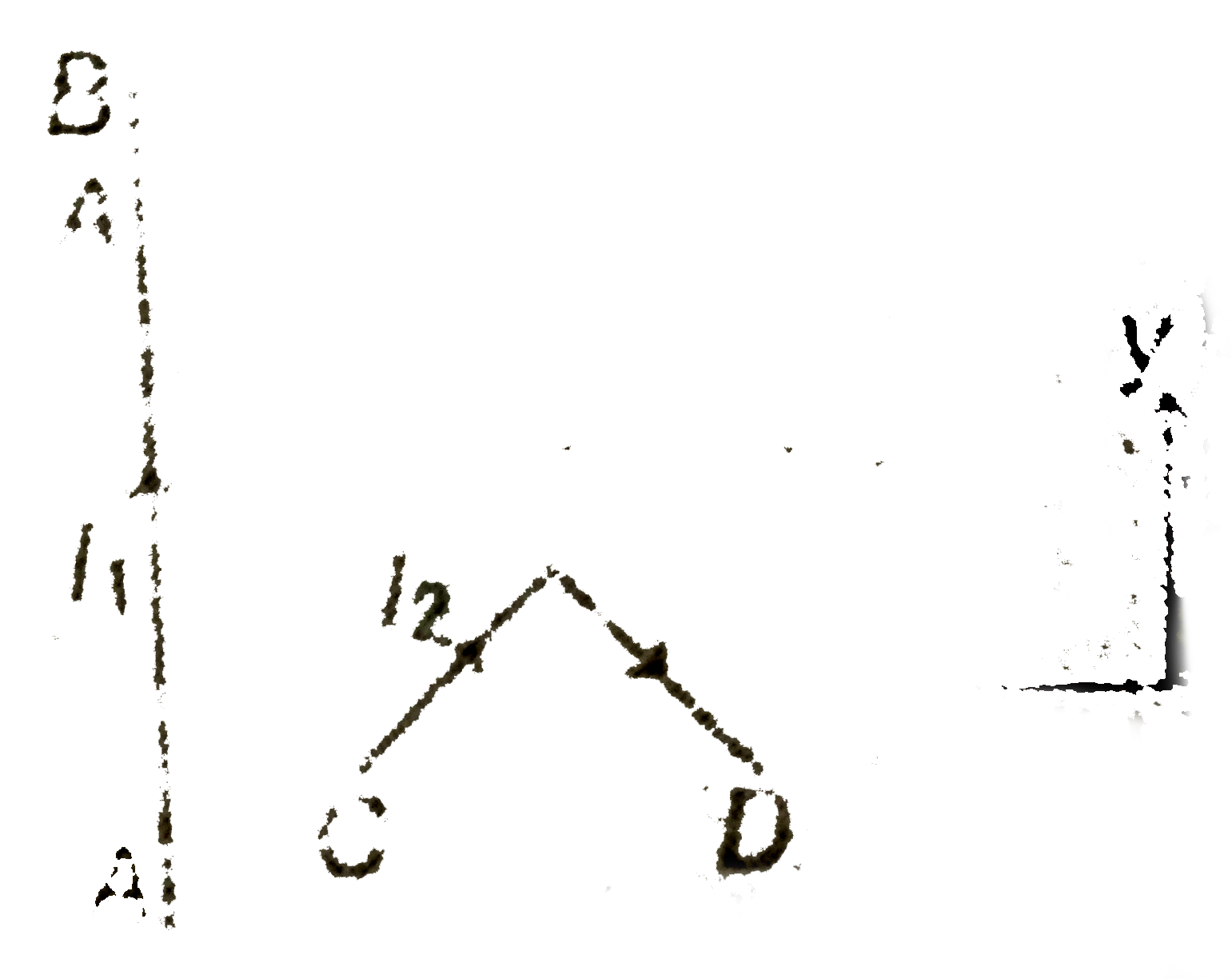 AzeroBtowards negative x-axisCtowards positive y-axisDNone of the above
AzeroBtowards negative x-axisCtowards positive y-axisDNone of the above
A wire AB is carrying current of 12 A and is lying on the table. Another wire CD carrying 5 A is held directly above AB at a height of 1 mm. Find the mass per unit length of the wire CD so that it remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that in AB . [Take the value of g=10ms−2]
View SolutionTwo long parallel straight wires, each carrying a current of 2 A are placed at distance of 3 cm. Calculate the force acting per unit length of each wire. Also, state the nature of force between the wires if the currents flow in the same direction in both the wires.
View SolutionA current carrying wire A is carrying current of 10 A from east to west and is fixed. Another wire B of maas 5 g, length 2 m and carrying a current 20 A is placed below the wire such that it remains suspended due to the magnetic force. Find the position of wire B and also indicate the direction of current in B. Take g=10m/s2.
View SolutionA straight long wire carrying current 50 A is placed on a horizontal surface. Another wire of unit length carrying 22 A current is balanced in air above at a distance of0.5 cm from the long wire. Calculate the mass of the wire.
View SolutionA conducting wire carrying 1 A of current is connected across a battery of 2V. Find the number of electrons flowing through the wire per second.
View Solution

