A magnetic field in a certain region is given by $B = B_{0} cos (ω t) ˆ k$ and a coil of radius a with resistance R is placed in the x-y plane with its centre at the origin in the magnetic field, Fig. Find the magnitude and the direction of the current at (a,0,0) $a t t = π / 2 ω, t = π / ω$ and $t = 3 π / 2 ω$ .

Video Solution

Text Solution

Verified by Experts

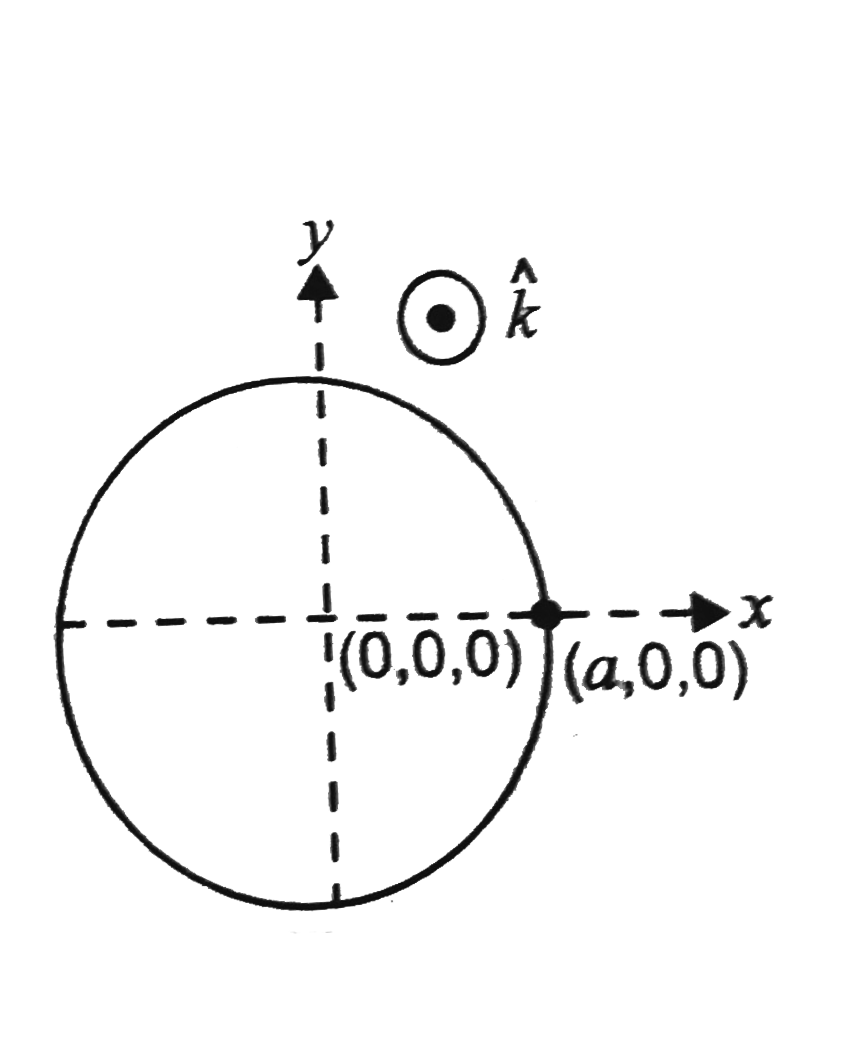
Here, $\to B = B_{0} cos (ω t) ˆ k$
i.e., magnetic field is alond positive z-axis. Fig
Magnetic flux linked with the ring
$ϕ = B A {cos 0}^{\circ} = B (0) cos ω t (π a^{2})$
emf induced in the ring, $e = - \frac{d ϕ}{d t} = - \frac{d}{d t} [B_{0} π a^{2} cos ω t] = B_{0} π a^{2} ω sin ω t$
current induced, $I = \frac{e}{R} = \frac{B_{0} π a^{2} ω sin ω t}{R}$
At $t = \frac{π}{2 ω}$ , $I = \frac{B_{0} π a^{2} ω}{R} \frac{sin (π)}{2} = \frac{B π a^{2} ω}{R}$ , along $ˆ j$
At $t = \frac{π}{ω}$ , $I = \frac{B_{0} π a^{2} ω}{R} sin π =$ zero
At $t = \frac{3 π}{2 ω}$ , $I = \frac{B_{0} π a^{2} ω}{R} sin (\frac{3 π}{R}) = - \frac{B_{0} π a^{2} ω}{R}$ , along $- ˆ j$
Note that the direction of current at any instant is as per Lenz's law.

Updated on:21/07/2023

If an alternating current is given by i = a sin ( $ω$ t) + b cos ( $ω$ t), then RMS value of current is :-
A $\frac{a}{\sqrt{2}} + \frac{b}{\sqrt{2}}$
B $\frac{a + b}{\sqrt{2}}$
C $\sqrt{\frac{a^{2} + b^{2}}{2}}$
D $\frac{a^{2} + b^{2}}{\sqrt{2}}$
A metallic ring of radius a and resistance R is held fixed with its axis along a spatially uniform magnetic field whose magnitude is $B_{0}$ sin $(ω t)$ . Neglect gravity. Then,
Athe current in the ring oscillates with a frequency of $2 ω$ .
Bthe joule hearting loss in the ring is proportional to $a^{2}$ ,
Cthe force per unit length on the ring will be proportional to $B_{0}^{2}$ .
Dthe net force on the ring is non-zero
A coil of resistance 400 $Ω$ is placed in a magnetic field. If the magnetic flux $ϕ$ (Wb) linked with the coil varies with time t (s) as $ϕ = 50 t_{2} + 4$ . The current in the coil at t=2 s is
A0.5 A
B0.1 A
C $2 A$
D $1 A$