An e.m. wave trevels in vacuum along z direction:
→E=(E1ˆi+E2ˆj)cos(kz−ωt). Choose the correct option from the following :
The associated magnetic field is given as →B=1c(E1ˆi+E2ˆj)cos(kz−ωt)
The associated magnetic field is given as →B=1c(E1ˆi−E2ˆj)cos(kz−ω)
The given electromagnetic field circular polarised
The given e.m. wave is plane polarised
The correct Answer is:(a,d)
In em wave, the electric field vector is given as, →E=(E1ˆi+E2ˆj)cos(kz−ωt)
In em wave, the associated magnetic field vector, →B=→Ec=E1ˆi+E2ˆjccos(kz−ωt)
Thus, option (a) is correct. As →Eand→B are perpendicular to each other and the propagation of em
wave is ⊥r to →E as well as →B, so the given em wave is plane polarised. Thus option (d) is true.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - (C) Problems for practice1videos
ELECTROMAGNETIC WAVES
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Multiple choice questions1videos
ELECTROMAGNETIC WAVES
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Value based questions5videos
ELECTROMAGNETIC WAVES
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Multiple Choice Questions1videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
- BOOK - PRADEEPCHAPTER - ELECTRONIC DEVICESEXERCISE - Fill in the Blanks1videos
ELECTRONIC DEVICES
Similar Questions
Identify A to E choose the correct option accordingly .
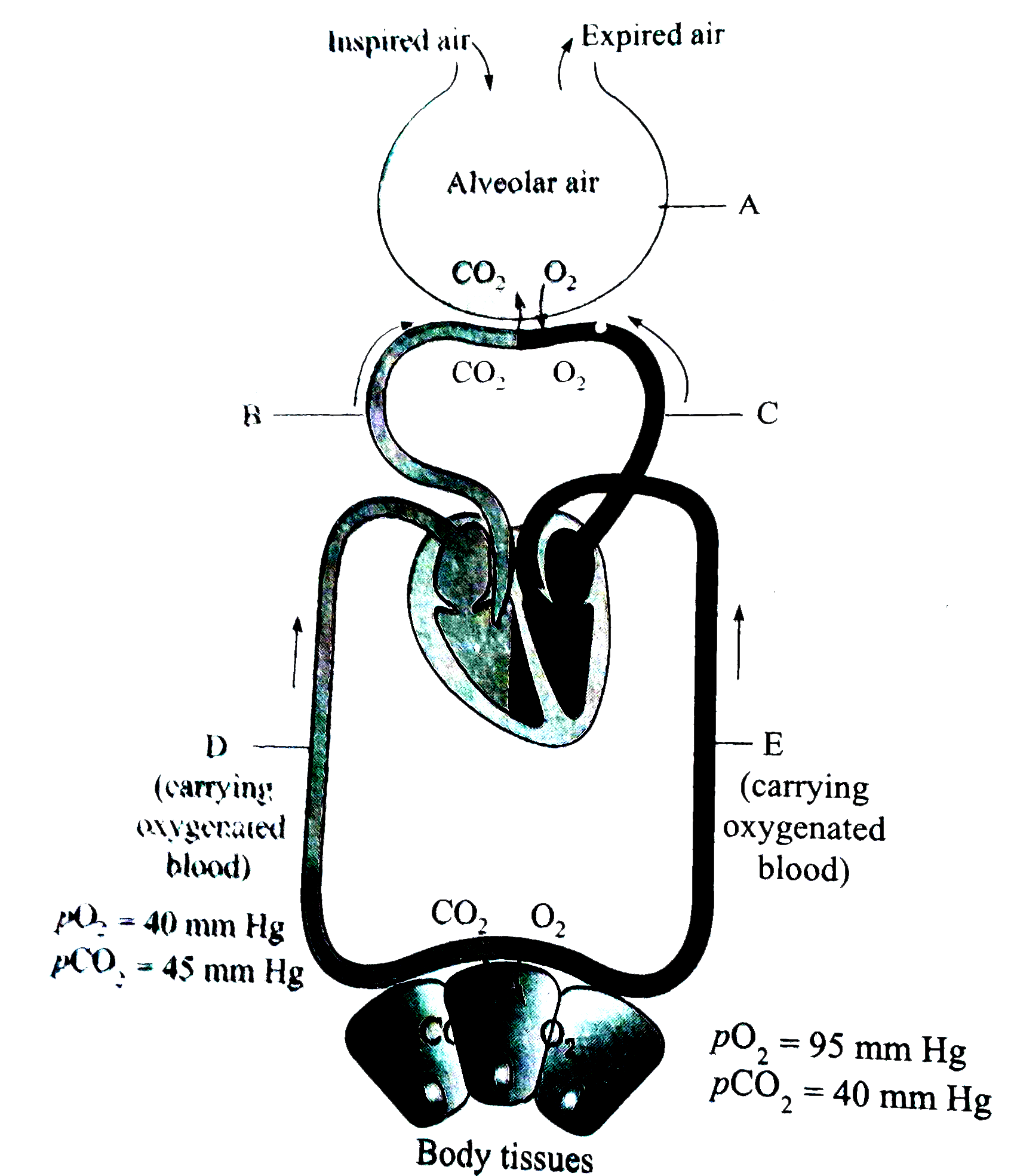 View Solution
View SolutionA plane EM wave travelling in vacuum along z-direction is given by E=E0 sin (kz−ωt)ˆi and B=B0 sin (kz−ωt)ˆj
(i) Evaluate ∫E.dl over the rectangular loop 1234 shown in figure.
(ii) Evaluate ∫B.ds over the surface bounded by loop 1234.
(iii) Use equation ∫E.dl=−dϕBdt to prove E0B0=c.
(iv) By using similar proces and the equation
∫B.dl=μ0I+ε0dϕEdt, prove that c1√μ0ε0
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
An electromagnetic wave travelling along z-axis is given as E=E0 cos (kz−ωt). Choose the correct options from the following
AThe associated magnetic field is given as →B=1cˆk×→EBThe e.m. wave field can be written in terms of the associated magnetic field as →E=c(→B×׈k)Cˆk.→E=0,ˆk.→B=0Dˆk×→E=0,ˆk×→B=0 - Question 2 - Select One or More
An electromagnetic wave travelling along z-axis is given as E=E0 cos (kz−ωt). Choose the correct options from the following
AThe associated magnetic field is given as B=1cˆk×E=1ω(ˆk×E)BThe electromagnetic field can be written in terms of the associated magnetic field as E=c(Bxˆk)Cˆk.E=0,ˆk.B=0Dˆk×E=0,ˆk×B=0 - Question 3 - Select One
choose e correct option w.r.t. leg- haemoglobin
AFound in root nodules of grassesBOxygen scavengerCProvides suitable conditions for nitrogenase activityDBoth (2) and (3)
A plane EM wave travelling in vacuum along z-direction is given by E=E0 sin (kz−ωt)ˆi and B=B0 sin (kz−ωt)ˆj
(i) Evaluate ∫E.dl over the rectangular loop 1234 shown in figure.
(ii) Evaluate ∫B.ds over the surface bounded by loop 1234.
(iii) Use equation ∫E.dl=−dϕBdt to prove E0B0=c.
(iv) By using similar proces and the equation
∫B.dl=μ0I+ε0dϕEdt, prove that c1√μ0ε0
 View Solution
View SolutionA plane EM wave travelling along z direction is described by
E=E0sin(kz−ωt)ˆiandB=B0sin(kz−ωt)ˆj.
show that
(i) The average energy density of the wave is given by uav=14ε0E20+14B20μ0.
(ii) The time averaged intensity of the wave is given by Iav=12cε0E20.View SolutionThe electric field of a plane electromagnetic wave propagating along the x direction in vacuum is →E=E0ˆjcos(ωt−kx) . The magnetic field →B, at the moment t=0 is :
View SolutionIf the wave-front of e.m.wave travelling in vacuum is given by , →r=ˆi+ˆj+ˆk, and find the angle made by the direction of propagation of e.m. wave with y-axis.
View Solution(a) An e.m. wave is travelling in a medium with a velocity →v=vˆi.Draw a sketch showing the propagation of the e.m. wave, indicating the direction of the oscillating electric and magentic fields.
(b) How are the magnitudes of the electric and magnetic fields related to the velocity of the e.m. wave?View Solution

