The photo electric current at distances r1andr2 of light source from photoelectric cell are I1andI2 respectively. Find the value of I1/I2.
To find the ratio of the photoelectric currents I1 and I2 at distances r1 and r2 from a light source, we can follow these steps:
1. Understand the Relationship Between Intensity and Distance:
The intensity I of light from a point source decreases with the square of the distance from the source. This is given by the inverse square law:
I∝1r2
where r is the distance from the light source.
2. Relate Intensity to Photoelectric Current:
The photoelectric current I produced is directly proportional to the intensity of the light incident on the photoelectric cell. Therefore, we can write:
I1∝1r21andI2∝1r22
3. Express the Currents in Terms of Intensities:
From the above relationships, we can express the currents as:
I1=k⋅1r21andI2=k⋅1r22
where k is a constant of proportionality.
4. Form the Ratio of the Currents:
To find the ratio I1I2, we can substitute the expressions for I1 and I2:
I1I2=k⋅1r21k⋅1r22=r22r21
5. Final Result:
Therefore, the ratio of the photoelectric currents at distances r1 and r2 is:
I1I2=r22r21
Summary:
The ratio of the photoelectric currents I1I2 is given by:
I1I2=r22r21
The intensity I of light from a point source decreases with the square of the distance from the source. This is given by the inverse square law:
I∝1r2
where r is the distance from the light source.
The photoelectric current I produced is directly proportional to the intensity of the light incident on the photoelectric cell. Therefore, we can write:
I1∝1r21andI2∝1r22
From the above relationships, we can express the currents as:
I1=k⋅1r21andI2=k⋅1r22
where k is a constant of proportionality.
To find the ratio I1I2, we can substitute the expressions for I1 and I2:
I1I2=k⋅1r21k⋅1r22=r22r21
Therefore, the ratio of the photoelectric currents at distances r1 and r2 is:
I1I2=r22r21
The ratio of the photoelectric currents I1I2 is given by:
I1I2=r22r21
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - NCERT Exerciese question
1 Video
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Short answer
1 Video
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Problems for Practice (B)
2 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Multiple Choice Questions
1 Video
Similar Questions
Find the value of 1+i2+i4+i6
View SolutionThe magnitude of gratitational field intensities at distance r1 and r2 from the centre of a uniform solid sphere of radius R and mass M are I1 and I2 respectively. Find the ratio of I1/I2 if (a) r1>R and r2>R and (b) r1<R and r2<R (c ) r1>R and r2<R.
View Solution
Knowledge Check
Find out values of i1,i2andi3
A0,1/5A,1/5AB1/5A,0,1/15AC0,1/15A,0D1/15A,1/15A,0In the given circuit currents I1 and I2 are
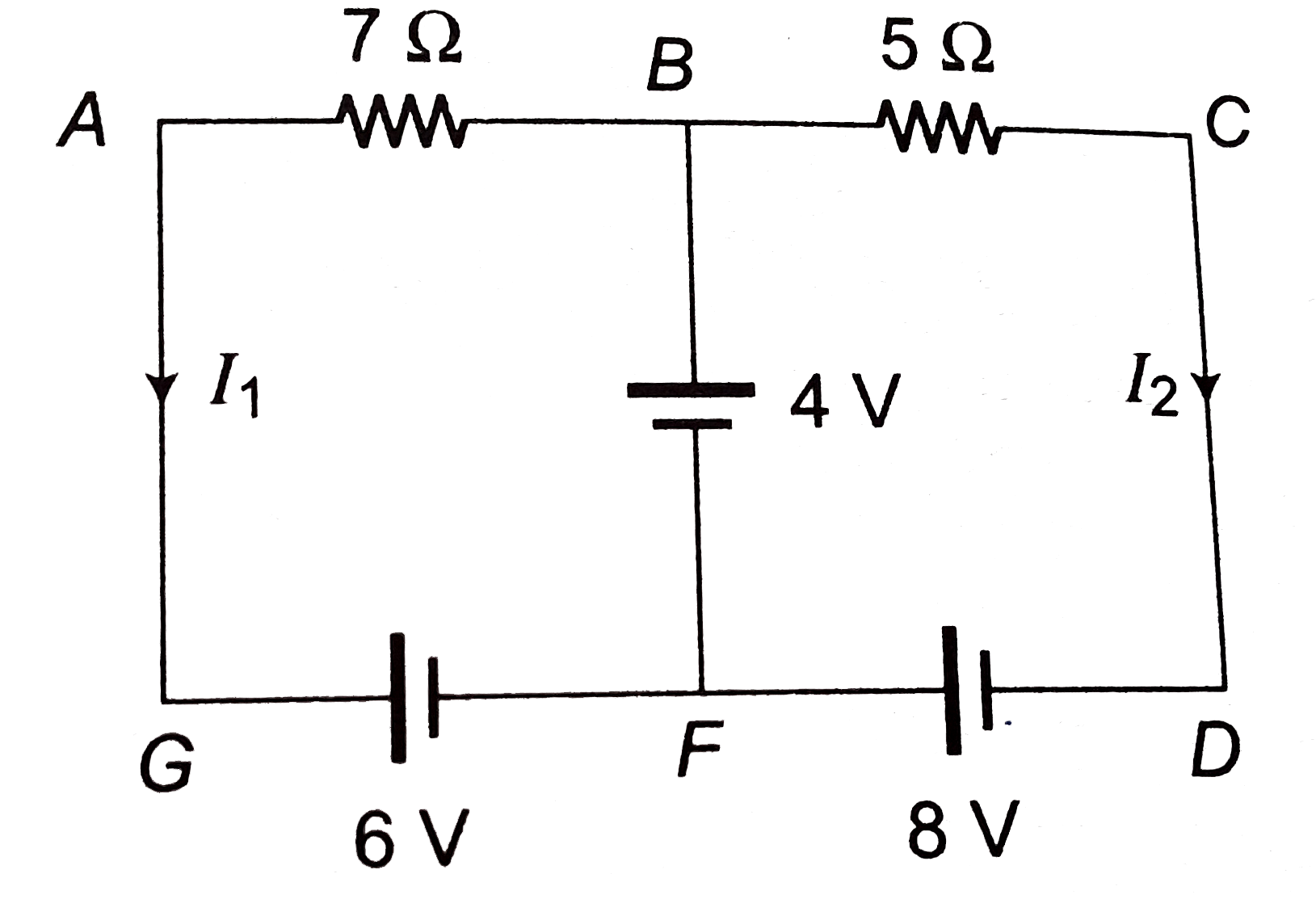 .A107A,127AB−27A,125AC87A,125AD87A,127A
.A107A,127AB−27A,125AC87A,125AD87A,127AIn the given circuit, if I1andI2 be the current in resistances R1andR2, respectively, then
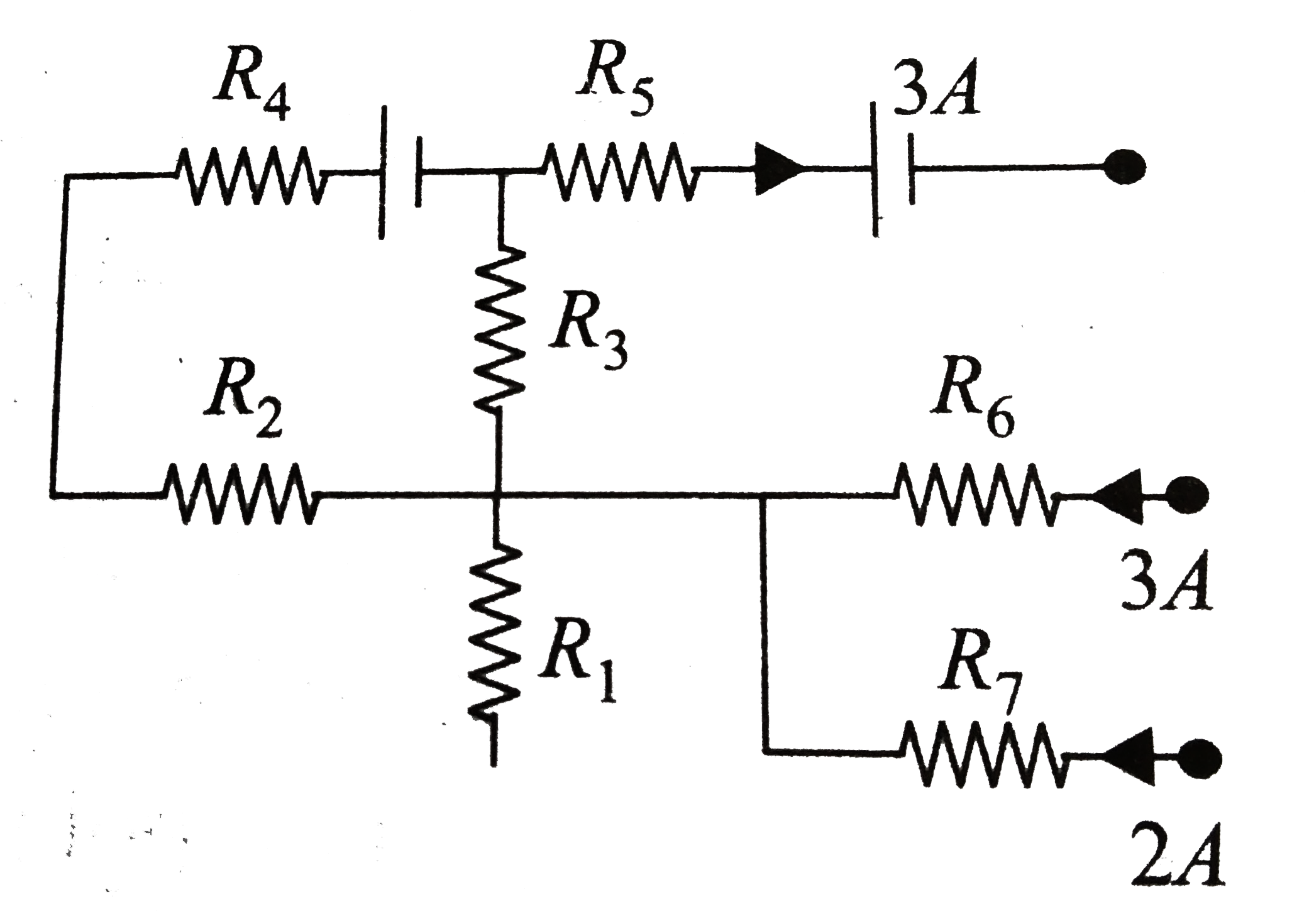 . AI1=3A,I2=2ABI1=0,I2=2ACI1=2AandI2 cannot be determined with given dataDnone of these
. AI1=3A,I2=2ABI1=0,I2=2ACI1=2AandI2 cannot be determined with given dataDnone of these
The magnitude of gravitational field at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M, respectively. Find the ratio of (I1)/(I2)) if r1>R and r2>R.
View SolutionFigure shows an LCR circuit. When the switch is closed, the currents through resistor R, inductor L, and capacitor C are I1,I2, and I3, respectively. Determine the values of I1,I2, and I3.
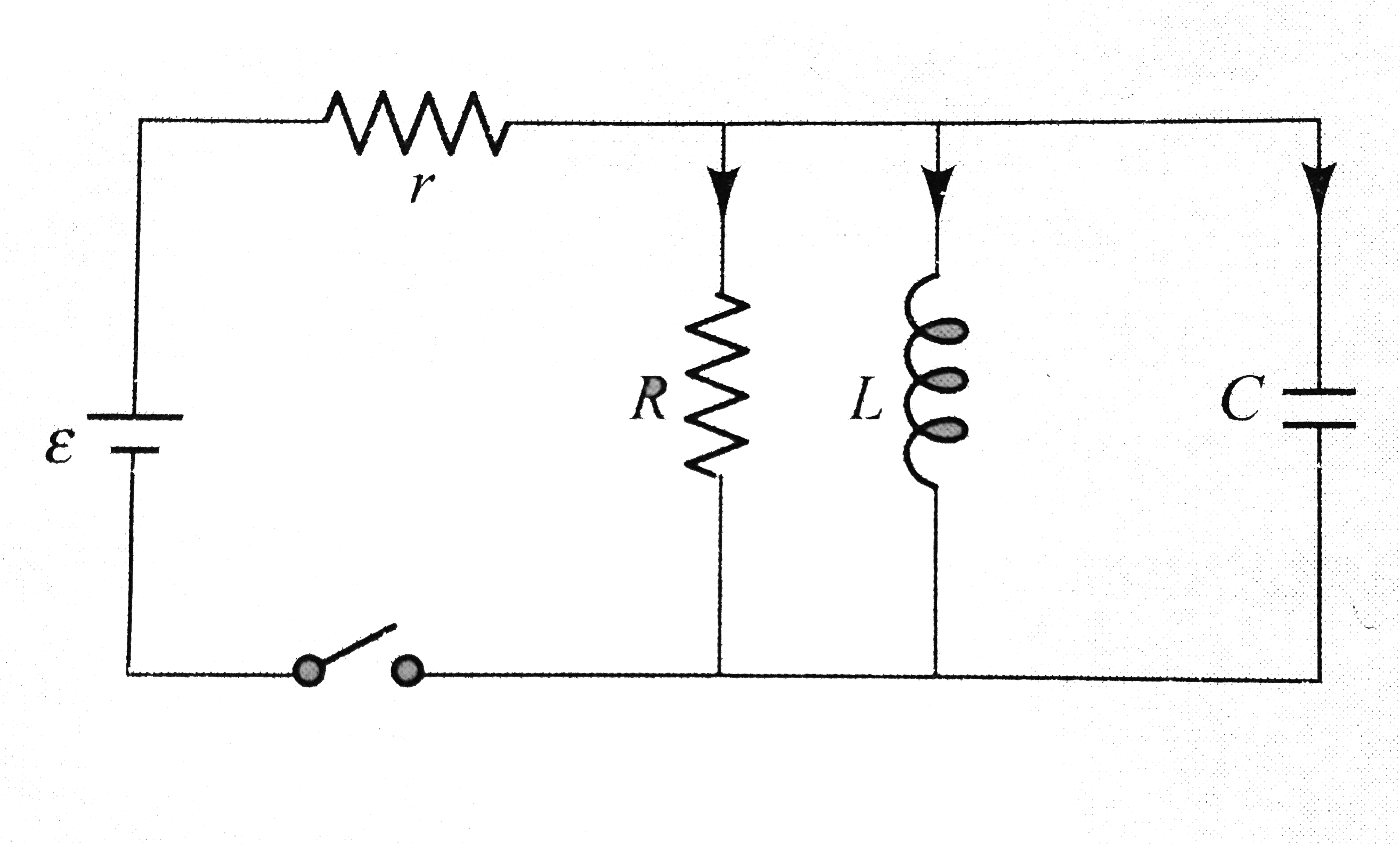
a. at t=0 b. at t=∞
View SolutionTwo sources of light whose ratio of intensities are I1I2=2x. Find the value of Imax−IminImax+Imin
View SolutionA battery of emf ε and internal resistance r sends currents I1 and I2, when connected to external resistance R1 and R2 respectively. Find the emf and internal resistance of the battery.
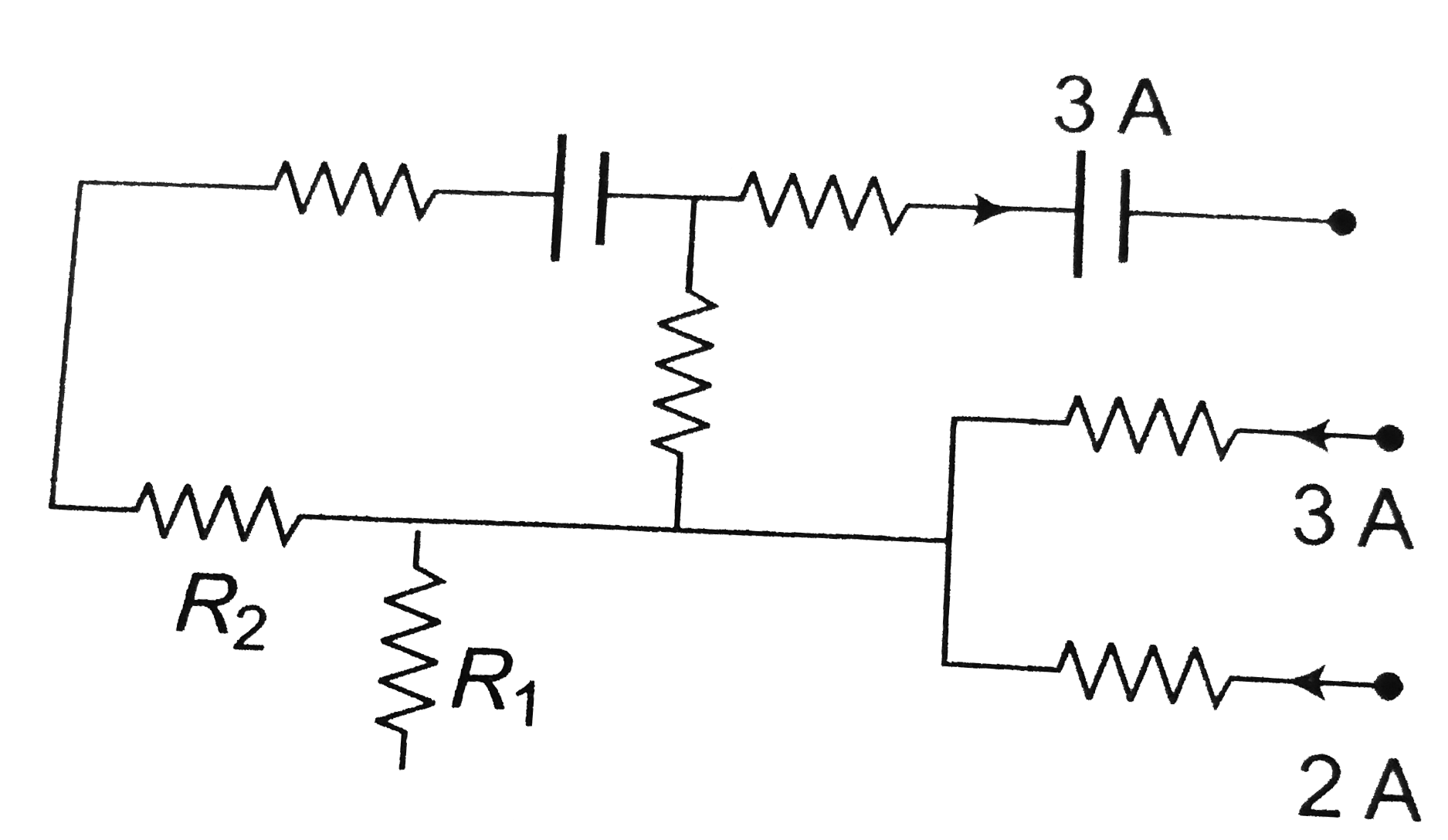
View SolutionIn the given circuit if I1 and I2 be the current in resistance R1 and R2 respectively then:
View Solution
