A neutron beam of energy E scatters from atoms on a surface with a spacing d=0.1nm. The first maximum of intensity in the reflected beam occures at θ=30∘. What is the kinetic energy of E of the beam in eV?
Here, d=0.1nm,θ=30∘,n=1. According to Bragg's law
2dsinθ=nλor2×0.1×sin30∘=1×λorλ=0.1nm=10−10m
p=hλ=6.62×10−3410−10=6.62×10−24kgms−1
KE=12p2m=12×(6.62×10−24)21.67×10−27J=12(6.62×10−24)2(1.67×10−27)×(1.6×10−19)eV=0.082eV
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - NCERT Exerciese question1videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Short answer1videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Problems for Practice (B)2videos
CURRENT ELECTRICITY
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Multiple Choice Questions1videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
Similar Questions
A beam of electrons of energy E scatters from a target having atomic specing of 1Å. The first maximum intensity occurs at θ=60∘. The E (in eV) is ___________
(Planck constant h=6.64×10−34Js,
1eV=1.6×1019J,
electron mass m=9.1×10−31kg)View SolutionA narrow beam of monoenergetic electrons falls normally on the surface of a Ni single crystal. The reflection maximum of fourth order is observed in the direction forming an anle θ=55∘ with the normal to the surface at the energy of the electrons equal to T=180eV. Calcualte the corresponding value of the interplanar distance.
View Solution
Knowledge Check
- Question 1 - Select One
In Davisson-Germer experiment, an electron beam of 60eV energy falls normally to the surface of the crystal and maximum intensity is obtained at an angle of 60∘ to the direction of incident beam. The inter- atomic distance in the lattice plane of the crystal is
A18ÅB3.6ÅC1.8 ÅD0.18Å - Question 2 - Select One
If light of wavelength of maximum intensity emitted from surface at temperature T1 Is used to cause photoelectric emission from a metallic surface, the maximum kinetic energy of the amitted electron is 6 ev, which is 3 time the work function of the metallic surface. If light of wavelength of maximum intensity emitted from a surface at temperature T2(T2=2T1) is used, the maximum kinetic energy of the photoelectrons emitted is
A2 eVB4 eVC14 eVD18 eV - Question 3 - Select One
The β-decay process, discovered around 1900, is basically the decay of a neutron (n), In the laboratory, a proton (p) and an electron (e−) are observed as the decay products of the neutron. Therefore, considering the decay of a neutron as a tro-body dcay process, it was observed that the electron kinetic energy has a continuous spectrum. Considering a three-body decay process i.e., n→p+e−+−ve, around 1930, Pauli explained the observed electron energy spectrum. Assuming the anti-neutrino (−Ve) to be massless and possessing negligible energy, and neutron to be at rest, momentum and energy conservation principles are applied. From this calculation, the maximum kinetic energy of the electron is 0.8×106eV. The kinetic energy carried by the proton is only the recoil energy.
What is the maximum energy of the anti-neutrino?AzeroBMuch less than 0.8×106eVCNearly 0.8×106eVDMuch larger than 0.8×106eV
The photoelectros emitted by an illuminted surface have a maximum kinetic energy of 3.0 eV. If the intensity of the light is tripled, What is the maximum kinetic energy of photoelectrons now?
View SolutionA neutron of kinetic energy 65 eV collides inelastically with a singly ionised helium atom at rest. It is scattered at an angle 90∘ with respect to original direction. If the energy of scattered neutron is 6.36 eV, find the frequency of emitted radiation from the helium atom after suffering collision.
View SolutionFig shows that energy levels of a mercury atom. Electrons with kinetic energies of 7.0 eV are introduced into mercury vapor. (a) Convert the energy levels to the system that sets the ground state at 0 eV. (b) What is the kinetic energy of an electron after an elastic collision with a mercury atom? (c ) What are the possible kinetic energies of an electron after an inelastic collision with a mercury atom? (d) What are the energies of the photons coming from the mercury vapor?
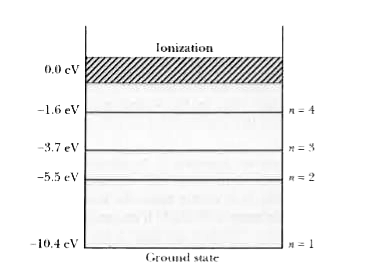 View Solution
View SolutionA light beam of 400nm falls on a metal surface of work function 1.1eV .Now light is replaced by another light beam of wavelength 590nm then ratio of maximum kinetic energy of photoelectrons in first and second case is? (i) 3:1 (1) 2:3
View SolutionA light beam of 400nm falls on a metal surface of work function 1.1eV .Now light is replaced by another light beam of wavelength 590nm then ratio of maximum kinetic energy of photoelectrons in first and second case is?
View Solution

