Two rings of same radius and mass are placed such that their centres are at a common point and their planes are perpendicular to each other. The moment of inertia of the system about an axis passing through the centre and perpendicular to the plane of one of the rings is (mass the ring =m, radius =r)
12MR2
MR2
32MR2
2MR2
The correct Answer is:C
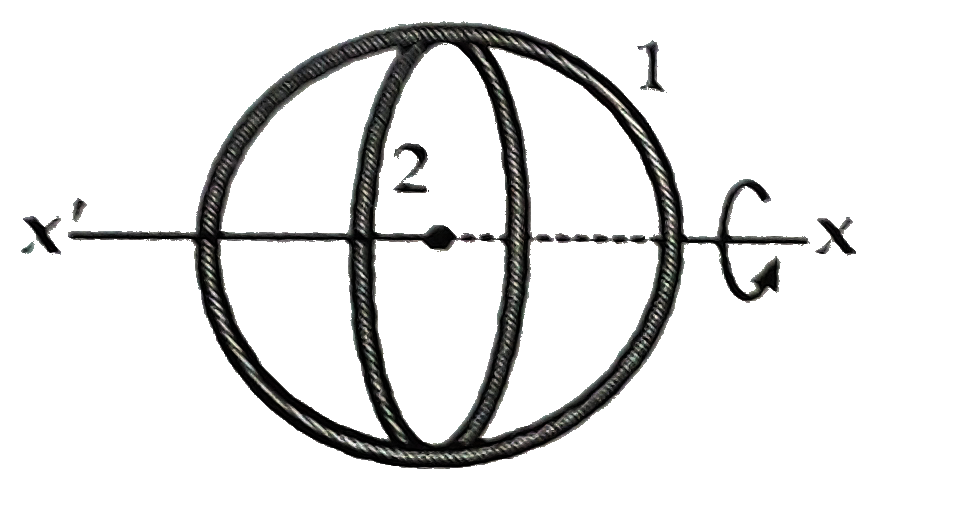
I1 = M.I. of ring about its diameter =12mR2
I2 = M.I. of ring about the axis normal to plane and passing through centre =mR2
Two rings are placed according to figure, Then
Ixx'=I1+I2=12mR2+mR2=32mR2.
Topper's Solved these Questions
Similar Questions
Calculate the moment of inertia of a disc of radius R and mass M, about an axis passing through its centre and perpendicular to the plane.
View SolutionTwo rings of the same radius R and M are placed such that their centres coincide and their planes are perpendicular to each other, the moment of inertia of the system about an axis passing through the diameters of both rings is
View Solution
Knowledge Check
- Question 1 - Select One
Two rings of same radius and mass are placed such that their centres are at a common point and their planes are perpendicular to each other. The moment of inertia of the system about an axis passing through the centre and perpendicular to the plane of one of the rings is (mass the ring =m, radius =r)
A12mr2Bmr2C32mr2D2mr2 - Question 2 - Select One
Two rings of same radius and mass are placed such that their centres are at a common point and their planes are perpendicular to each other. The moment of inertia of the system about an axis passing through the centre and perpendicular to the plane of one of the rings is (mass the ring =m, radius =r)
A12mr2Bmr2C32mr2D2mr2 - Question 3 - Select One
Two rings of the same radius and mass are placed such that their centres are at a common point and their planes are perpendicular to each other. The moment of inertia of the system about an axis passing through the centre and perpendicular to the plane of one of the rings is (mass of the ring = m, radius = r)
A12mr2Bmr2C32mr2D2mr2
Two rings have the same mass (m) and radius (r). They are placed in such away that their centres are at a common point and their planes are perpendicular to each other. What is the moment of inertia of the system about an axis passing through their centre and perpendicular to the plane of one of the ring ?
View SolutionTwo thin uniform circular rings each of radius 10 cm and mass 0.1 kg are arranged such that they have a common centre and their planes are perpendicular to each other. The moment of inertia of this system about an axis passing through their common centre and perpendicular to the plane of one of the rings in kg−m2 is
View SolutionTwo rings of the same mass and radius are placed such that their centers are at a common point and their planes are perpendicular to each other . The moment of inertia of the system about an axis passing through the diameter of one of the rings is I . Then moment of inertia of one of the ring of the system about the central axis and perpendicular to its plane would be
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionMoment of inertia of a circular ring about an axis through its centre and perpendicular to its plane is
View Solution