The total energy of rolling of mass 'm' and radius 'R' is
3/2mv2
1/2mv2
mv2
5/2mv2
The correct Answer is:C
E=12mv2[1+K2/R2]
=12mv2[1+1]=mv2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The total energy of rolling ring of mass m and radius R is
A3/2mv2B1/2mv2Cmv2D5/2mv2 - Question 2 - Select One
A body is mass m is rotating in a vertical circle of radius 'r' with critical speed. The difference in its K.E at the top and at the bottom is
A92 mgrB6 mgrC4 mgrD2 mgr - Question 3 - Select One
A half section of thin circular pipe of mass m and radius 'r' is released from rest in the position shown. Pipe rolls without sliding. The change in potential energy of pipe after it has rolled through 90∘ :
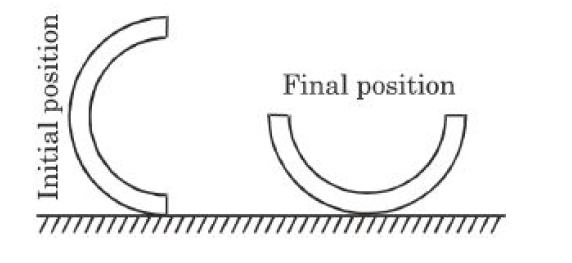 A4mgrπB2mgrπCmgrD√2mgr
A4mgrπB2mgrπCmgrD√2mgr
A satellite of mass m is Launched from the earth's surface in a circular orbit. If M and R denote the mas and radius of the earth respectively, then the total energy of the satellite at an altitude 2R is
View SolutionThe potential energy of a satellite of mass m revolving at height R above the surface of the earth where R= radius of earth is
View SolutionWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 3R ?
View SolutionWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
View SolutionWhat is the minimum energy required to launch a satellite of mass m from the surface of a planet of mass M and radius R in a circular orbit at an altitude of 2R?
View Solution

