The force constant of an ideal spring is 200 newton per meter. It is loaded with a mass of 200/π2 kg at the lower end the time period of its vibration is
2π2s
2s
1s
π2s
The correct Answer is:B
T=2π√mk=2π ⎷(200π2)200=2s
Topper's Solved these Questions
Similar Questions
The period of oscillation of a mass m suspended by an ideal spring is 2s. If an additional mass of 2 kg be suspended, the time period is increased by 1s. Find the value of m.
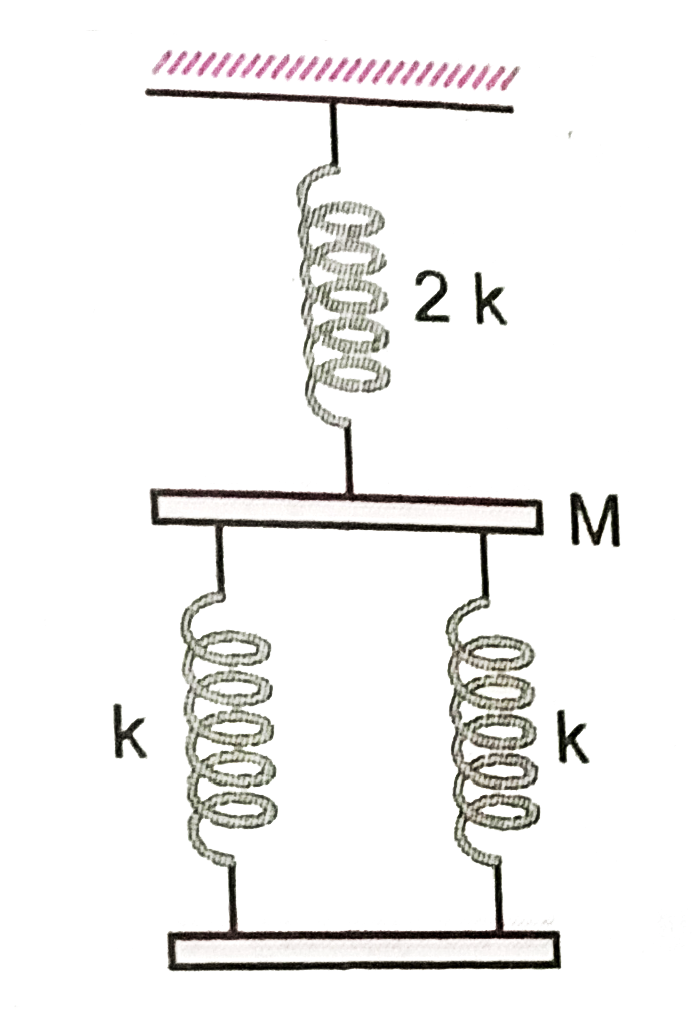
View SolutionThree spring are connected to a mass m(=100g) as shown in figure. Given k=2.5Nm−1. (a) What is the effecitve spring constant of the combination of spring constant of the combination of springs? (b) When mass m oscillates, find time period of its vibration.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A flat spiral spring of force constant K is loaded with mass M and oscillates vertically with a time period T. Then the mass suspended to the free end is
A4π2KT2BKT24π2CKT4π2DKT4π - Question 2 - Select One
A flat spiral spring of force constant k is loaded with mass M and oscillate about vertical with a time period T. Then the mass suspended to the free end is
A4π2kT2BkT24π2CkT4π2DkT4π
A spring of force constant 'k' is cut into four equal parts one part is attached with a mass m. The time period of oscillation will be
View SolutionA mass of 0.1 kg hangs at the end of a spring. When a mass of 0.01 kg more is added at the end of the spring, it stretches by 5 cm more. When the extra 0.01 kg mass is removed, determine the time period of vibration of the system.
View SolutionA light pulley is suspended at the lower end of a spring of constant k1, as shown in figure. An inextensible string passes over the pulley. At one end of string a mass m is suspended, the other end of the string is attached to another spring of constant k2. The other ends of both the springs are attached to rigid supports, as shown. Neglecting masses of springs and any friction, find the time period of small oscillations of mass m about equilibrium position.
 View Solution
View SolutionTwo blocks of masses 3kg and 6kg respectivley are placed on a smooth horizontal surface. They are connected by a light spring of force constant k=200N/m. Initially the spring is unstretched. The indicated velocities are imparted to the blocks. Find the maximum extension of the spring.
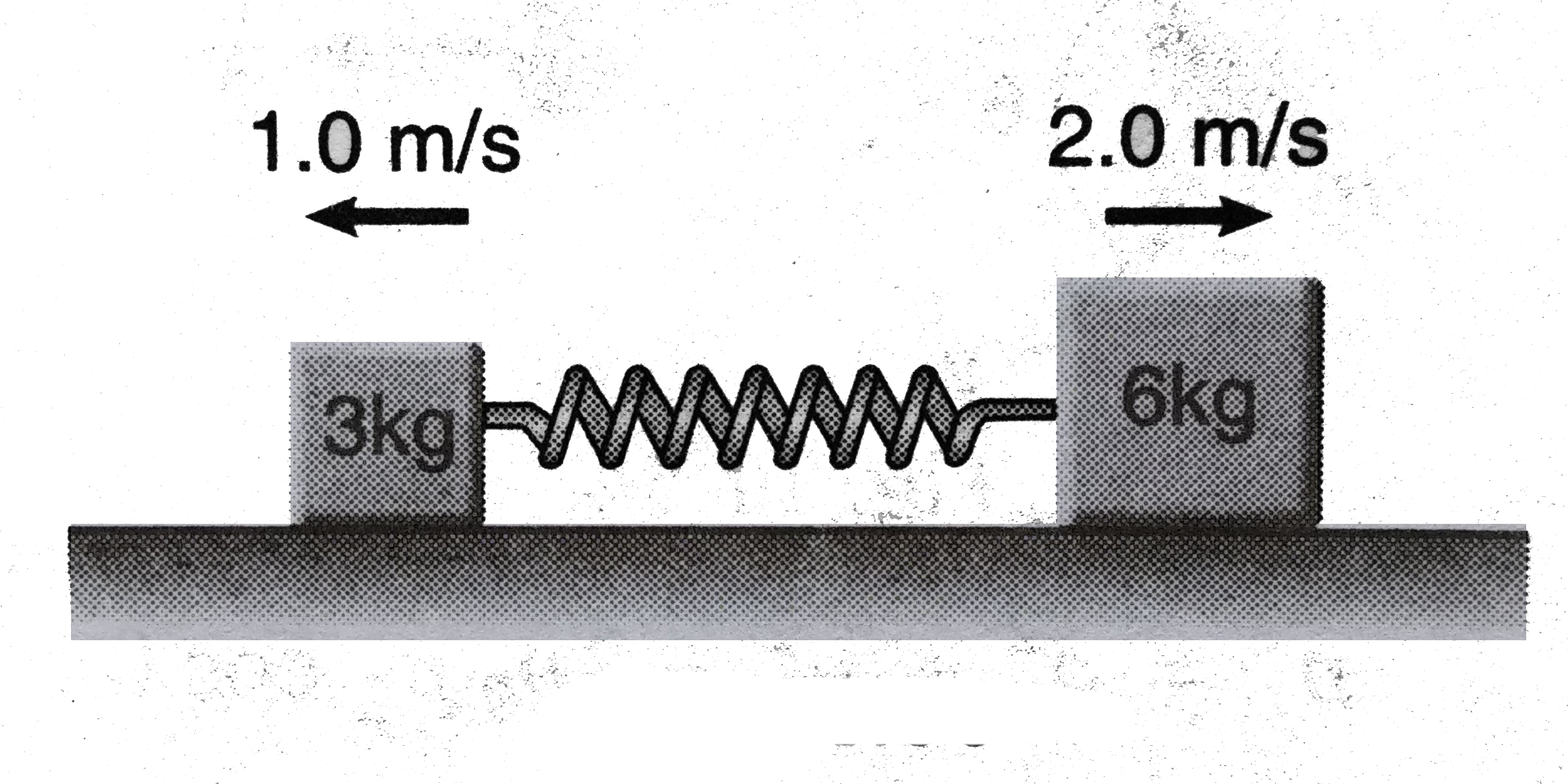 View Solution
View SolutionTwo blocks are connected by a spring of natural length 2 m. The force constant of spring is 200 N/m.
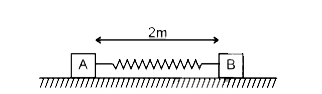
Find spring force in following situations :
A is kept at rest and B is displaced by 1 m in right direction.View SolutionTwo blocks are connected by a spring of natural length 2 m. The force constant of spring is 200 N/m.

Find spring force in following situations :
B is kept at rest and A is displaced by 1m in left direction.View Solution

