When two capacitors are connected in series, the equivalent capacitance is 1.2μF. When they are connected in parallel, the equivalent capacitance is 5μF. The capacitances of the capacitors are
3μFand2μF
4μFand1μF
2.5μFand2.5μF
3.5μFand1.5μF
The correct Answer is:A
To find the capacitances of the two capacitors C1 and C2 based on the given conditions, we can follow these steps:
Step 1: Understand the formulas for capacitors in series and parallel
When capacitors are connected in series, the equivalent capacitance Cs is given by:
1Cs=1C1+1C2
When capacitors are connected in parallel, the equivalent capacitance Cp is given by:
Cp=C1+C2
Step 2: Set up the equations based on the problem statement
From the problem, we know:
- Cs=1.2μF
- Cp=5μF
Using the series formula, we can rearrange it to:
C1C2=Cs(C1+C2)
Substituting Cs=1.2μF:
C1C2=1.2(C1+C2)
Using the parallel formula:
C1+C2=5μF
Step 3: Substitute C1+C2 into the series equation
Let C1+C2=5μF. We can substitute this into our earlier equation:
C1C2=1.2×5
C1C2=6μF2
Step 4: Set up a system of equations
Now we have two equations:
1. C1+C2=5
2. C1C2=6
Step 5: Solve the equations
Let's denote C1 and C2 as the roots of the quadratic equation:
x2−(C1+C2)x+C1C2=0
Substituting the known values:
x2−5x+6=0
Step 6: Factor the quadratic equation
Factoring the equation:
(x−2)(x−3)=0
Thus, the solutions are:
x=2orx=3
Step 7: Identify the capacitances
So, we have:
- C1=2μF
- C2=3μF
Conclusion
The capacitances of the two capacitors are C1=2μF and C2=3μF.
When capacitors are connected in series, the equivalent capacitance Cs is given by:
1Cs=1C1+1C2
When capacitors are connected in parallel, the equivalent capacitance Cp is given by:
Cp=C1+C2
From the problem, we know:
- Cs=1.2μF
- Cp=5μF
C1C2=Cs(C1+C2)
Substituting Cs=1.2μF:
C1C2=1.2(C1+C2)
C1+C2=5μF
Let C1+C2=5μF. We can substitute this into our earlier equation:
C1C2=1.2×5
C1C2=6μF2
Now we have two equations:
1. C1+C2=5
2. C1C2=6
Let's denote C1 and C2 as the roots of the quadratic equation:
x2−(C1+C2)x+C1C2=0
Substituting the known values:
x2−5x+6=0
Factoring the equation:
(x−2)(x−3)=0
Thus, the solutions are:
x=2orx=3
So, we have:
- C1=2μF
- C2=3μF
The capacitances of the two capacitors are C1=2μF and C2=3μF.
Topper's Solved these Questions
Similar Questions
When n, identical capacitors are connected in series then equivalent capacitance becomes x, and when the same are connected in parallel then equivalent capacitance becomes y. Calculate y/x.
View SolutionDerive an expression for the effective capacitance of three capacitors connected in series.
View Solution
Knowledge Check
- Question 1 - Select One
When two capacitors are connected in series, the equivalent capacitance is 154μF. When they are connected in parallel, the equivalent capacitance is 16μF. The individual capacitance are
A5μF,11μFB6μF,10μFC4μF,12μFD8μF,8μF - Question 2 - Select One
When two capacitances are connected in series, the equivalent capacitance is 2.4μF and when they connected in parallel, it is 10μF. The individual capacitances are
A6μFand4μFB5μFand5μFC7μFand3μFD8μFand2μF - Question 3 - Select One
Two identical capacitors when joined in series have an effective capacitance of 3μF. When connected in parallel, the effective capacitance becomes 12μF. What is the capacitance of each capacitor ?
A6μFB3μFC12μFD9μF
When three identical capacitors are connected in series, their equivalent capacitance is 2μF . Now they are connected in parallel across a source of e.m.f. 200 V. The total energy stored is
View SolutionCondensers of capacity 4μF, 5μF and 6μF are connected first in series . The effective capacitance is C1. When they are connected in parallel, the effective capacitance is C2. Then the ratio C2/C1 will be
View SolutionSeven capacitor are connected as shown in the figure.
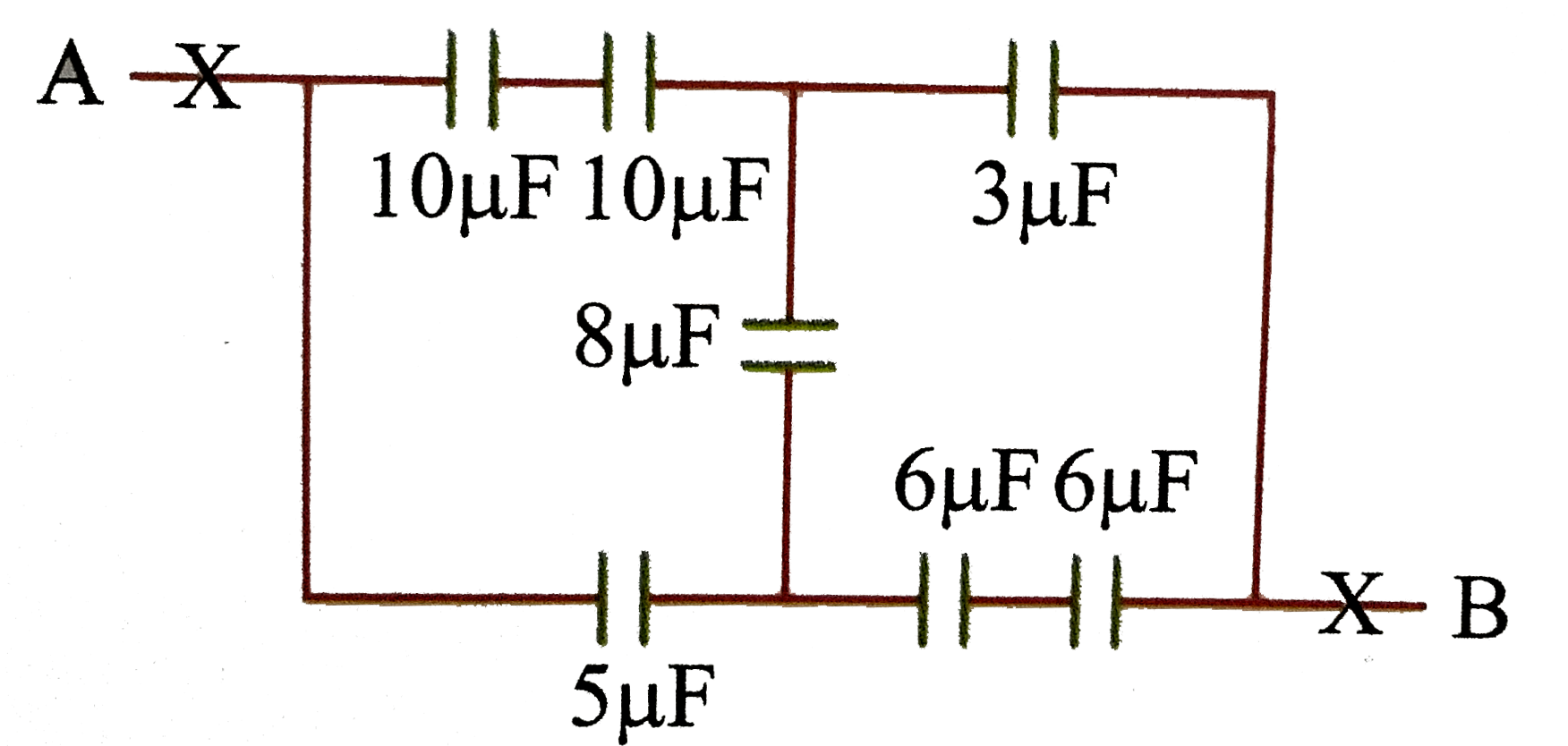 .
.
The equivalent capacitance in μF between A and B is.View Solutionn idential capacitors each of capacitance C when connected in series give equivalent capacitance 1.2μF and when connect in parallel give 120μF. Then n and C are
View SolutionWhen a number of capacitor are connected in parallel between two points, the equivalent capacitance
View Solution

