Show that the equation of the straight line joining the points (a,b) and (c,d) can be written as (x−a)(y−d)=(x−c)(y−b).Solution in Bengali
Step by step video & image solution for Show that the equation of the straight line joining the points (a,b) and (c,d) can be written as (x-a)(y-d)=(x-c)(y-b). by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- STRAIGHT LINEBOOK - CHHAYA PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - EXERCISE 2A (Short Answer Type Questions )
26 Videos
- STRAIGHT LINEBOOK - CHHAYA PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - EXERCISE 2A (Long Answer Type Questions )
19 Videos
- STRAIGHT LINEBOOK - CHHAYA PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - EXERCISE 2A (Multiple Choice Type Questions)
20 Videos
- MATHEMATICAL INDUCTION BOOK - CHHAYA PUBLICATIONCHAPTER - MATHEMATICAL INDUCTION EXERCISE - Sample Questions for Competitive Exams
20 Videos
Similar Questions
Find the equation of the straight line passing through the points (3,-4) and (1,2) and hence ,show that the three points (3,-4) , (1,2) and (2,-1) are collinear.
View SolutionA straigh line passes through the point (2,3) and is such that the sum of its intercepts on the coordinate axes is 10. Find the equation of the straight line.
View SolutionThe slope of the x- axis or of a line parallel to x-axis is
View SolutionIf for all n∈N and n≥1, then (32n−1) is always divisible by
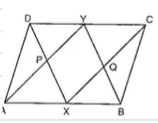
View SolutionIn Figure, X,Y are the mid-points of opposite sides AB and DC of a parallelogram ABCD.AY and DX are joined intersecting inP;CX and BY are joined intersecting in Q. Show that DXBY is a parallelogram, PXQY is parallelogram.
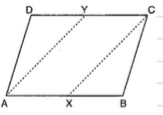
View SolutionIn Figure, ABCD is a parallelogram and X,Y are the mid=points of sides AB and DC respectively. Show that AXCY is a parallelogram.
View SolutionABCD is a parallelogram and line segments AX,CY bisect the angles AandC, respectively. Show that AX∣∣CY
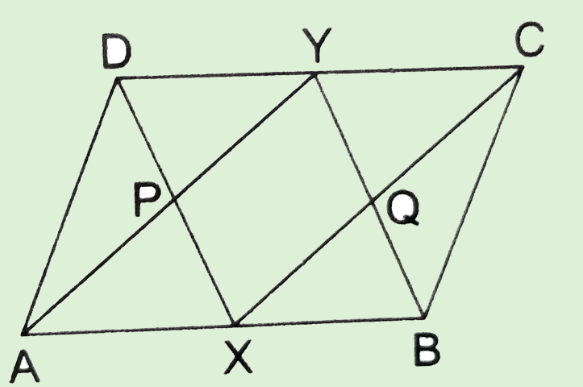
View SolutionIn Figure, X, Y are the mid-points of opposite sides AB and DC of a parallelogram ABCD.AY and DX are joined intersecting in P;CX and BY are joined intersecting in Q . Show that AXCY is a parallelogram (ii) DXBY is a parallelogram quadrilateral PXQY is a parallelogram
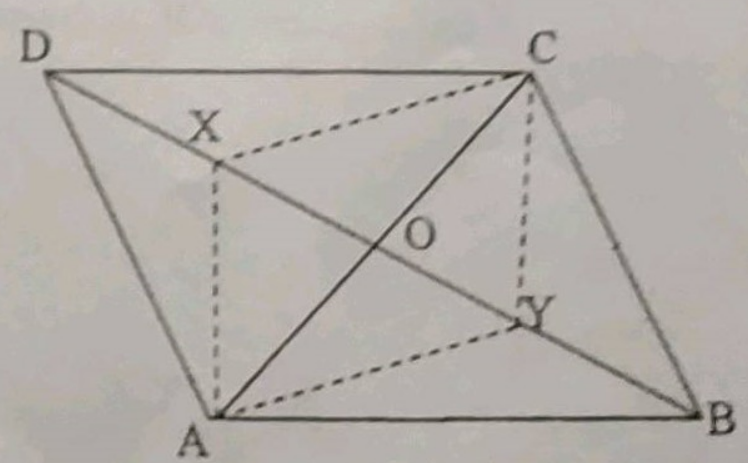
View SolutionIn Figure, ABCD is a parallelogram and X and Y are points on the diagonal BD such that DX=BY . Prove that AXCY is a parallelogram (ii) AX=CY, AY=CX AYB ≅CXD (iv) AXD≅ CYB
View Solutionদেখাও যে, (a,b) ও (c,d) বিন্দু দুটির সংযোজক সরলরেখার সমীকরণকে (x−a)(y−d)=(x−c)(y−b) আকারে প্রকাশ করা যায়।
View Solutionx=az+b,y=cz+d এবং x=a'z+b',y=c'z+d' সরলরেখা দুটি সামতলিক হলে করো যে, (a−a')(d−d')=(b−b')(c−c')
View SolutionIf (xa)+(yb)=1 and (xc)+(yd)=1 intersect the axes at four concylic points and a2+c2=b2+d2, then these lines can intersect at, (a,b,c,d>0)
View SolutionIn Figure, X,Y are the mid-points of opposite sides AB and DC of a parallelogram ABCD.AY and DX are joined intersecting inP;CX and BY are joined intersecting in Q. Show that DXBY is a parallelogram, PXQY is parallelogram.
View SolutionIn Figure, ABCD is a parallelogram and X,Y are the mid=points of sides AB and DC respectively. Show that AXCY is a parallelogram. Figure
View SolutionABCD is a parallelogram and line segments AX, CY bisect the angles A and C, respectively. Show that AX∣∣CY
View Solution
