The line segments AB and PQ intersect each other at the point C. AP and BQ are perpendiculars on AB respectively. If CA=20cm, CB=8cm and AP=10cm, then find the value of BQ.Solution in Bengali
Step by step video & image solution for The line segments AB and PQ intersect each other at the point C. AP and BQ are perpendiculars on AB respectively. If CA=20cm, CB=8cm and AP=10cm, then find the value of BQ. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMILARITYBOOK - CALCUTTA BOOK HOUSECHAPTER - SIMILARITYEXERCISE - Exercise 5.2
27 Videos
- SIMILARITYBOOK - CALCUTTA BOOK HOUSECHAPTER - SIMILARITYEXERCISE - Exercise 5.2
27 Videos
- SAMPLE QUESTIONS PAPERSBOOK - CALCUTTA BOOK HOUSECHAPTER - SAMPLE QUESTIONS PAPERSEXERCISE - QUESTION
157 Videos
- SIMPLE INTERESTBOOK - CALCUTTA BOOK HOUSECHAPTER - SIMPLE INTERESTEXERCISE - Long-answer type questions
37 Videos
Similar Questions
In ΔPQR and ΔDEF, PQDE=QREF=RPFD. Then which one of the following is correct?
View SolutionIn ΔPQR and ΔDEF, PQDE=QREF=RPFD. Then which one of the following is correct?
View SolutionA person deposite ₹ 100 in a bank and after 2 yars he got a total ₹121 as a return. Then the componund interest of the bank per annum is
View SolutionGoutam took a loan of some money from a cooperative bank for opening a poultry farm at the rate of simple interest of 12% per annum. Every month he has to repay 378Rs as interest. Determine the loan amount taken by him.
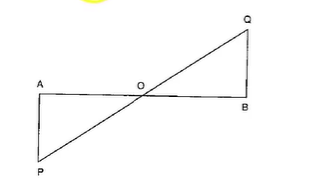
View SolutionIn Figure, AP and BQ are perpendiculars to the line segment AB and AP=BQ. Prove that O is the mid-point of line segment AB and PQ.
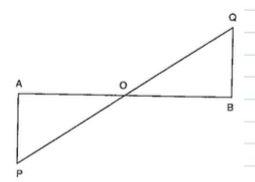
View SolutionIn Figure, AP and BQ are perpendiculars to the line segment AB and AP=BQ . Prove that O is the mid-point of line segment AB and PQ
View SolutionLet AP and BQ be two vertical poles at points A and B respectively. If AP=16m, BQ=22m and AB=20m , then find the distance of a point R on AB from the point A such that RP2+RQ2 is minimum.
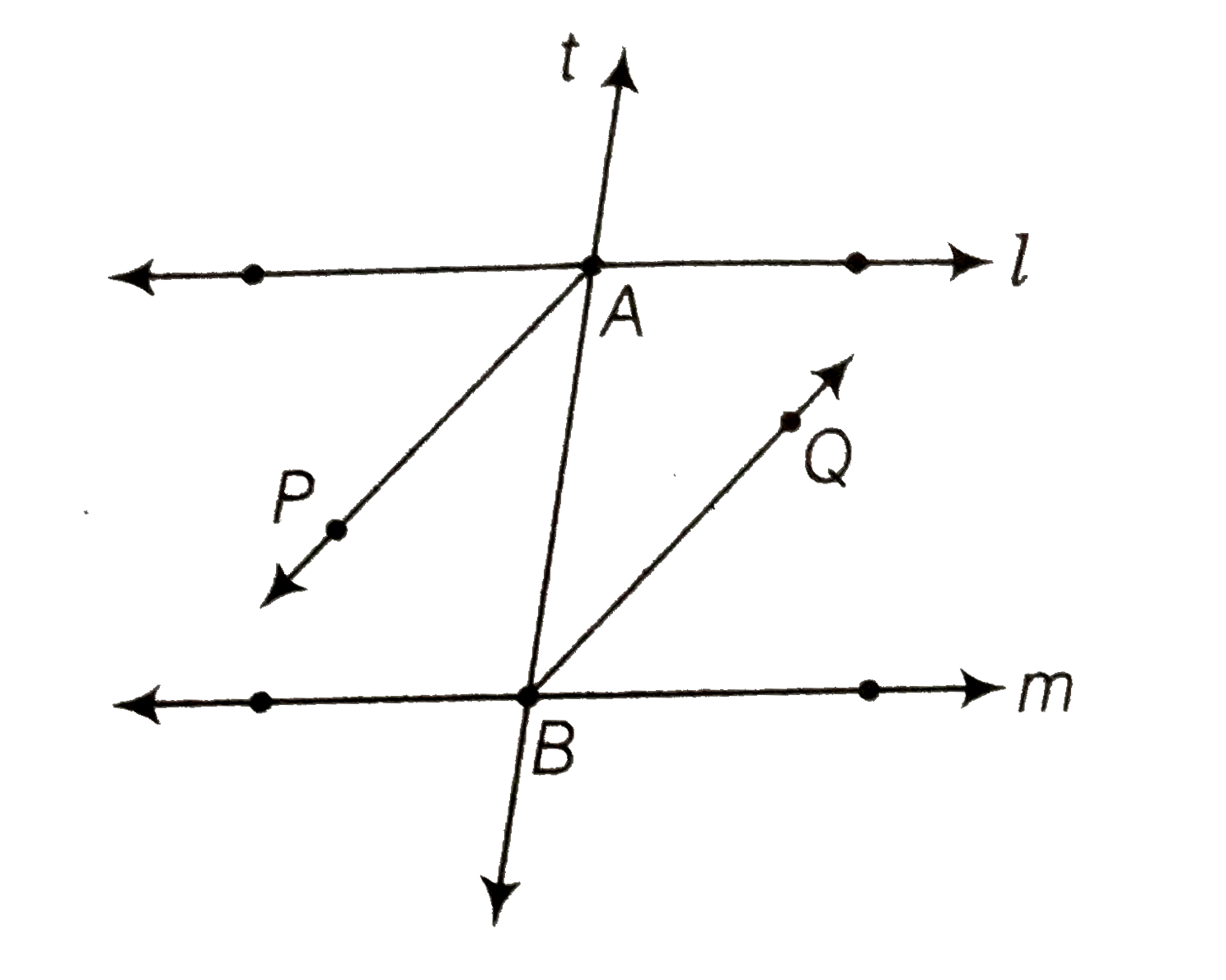
View SolutionAP and BQ are the bisectors of the two alternate interior angles formed by the intersection of a transversal t with parallel lines l and m (in the given figure). Show that AP||BQ.
View Solutionमान लीजिए बिंदु A और B पर क्रमशः AP तथा BQ दो उर्ध्वाधर स्तंभ है| यदि AP = 16 m, BQ = 22 m और AB = 20 m हों तो AB पर एक ऐसा बिंदु R ज्ञात कीजिए ताकि RP2+RQ2 निम्नतम है |
View Solutionमान लीजिए बिंदु A और B पर क्रमशः AP तथा BQ दो ऊध्वर्रधकर स्तम्भ है । यदि AP =16 मि, BQ =22 मि और AB = 20 मि हो तो AB पर एक ऐसा बिंदु R ज्ञात कीजिए ताकि RP2+RQ2 निम्नतम हो।
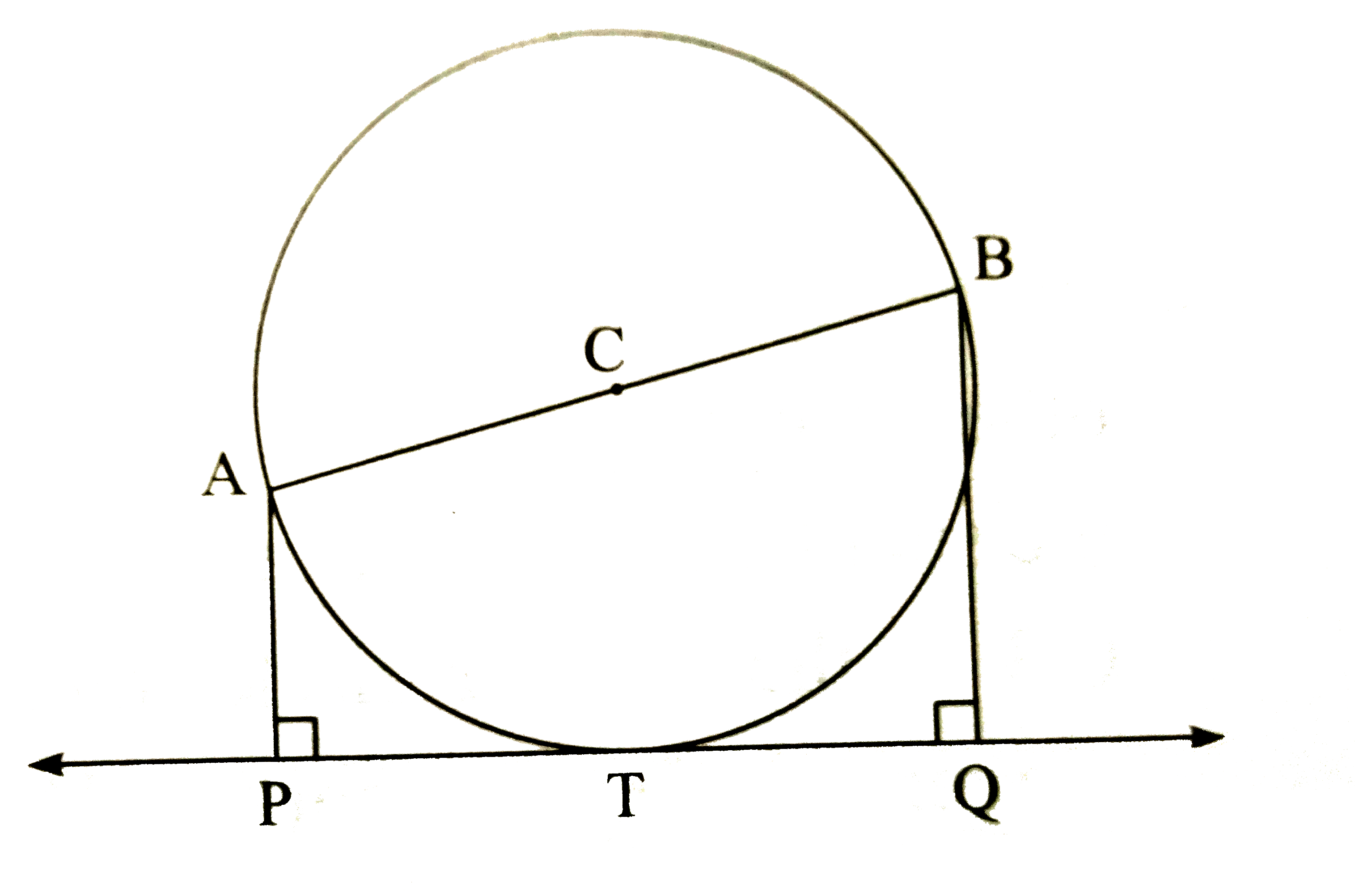
View SolutionIn the figure, seg AB is a diameter of a circle with centre C. Line PQ is a tangent , which touches the circle at point T. seg AP⊥ line PQ and seg BQ⊥ line PQ.
Prove that, seg CP≅ seg CQ.
View SolutionIn trapezium ABCD side AB∣∣ side PQ∣∣ side DC,AP=15,PD=12,QC=14, find BQ.
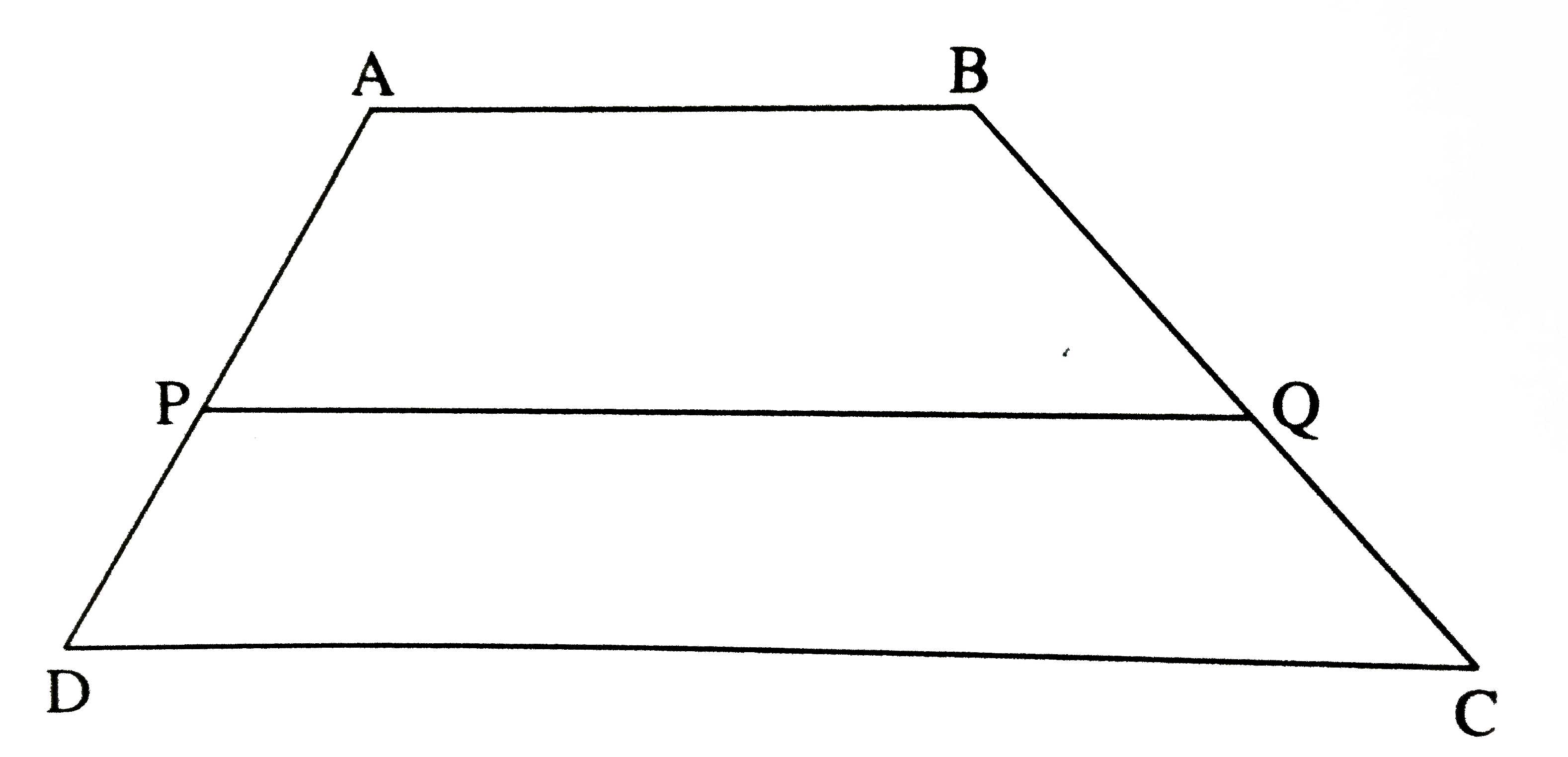
View SolutionIn the adjoining figure, seg AB is a diameter of a circle with centre C . Line PQ is a tangent, it touhces the circle at T. segs AP and BQ are perpendiculars to line PQ. Prove seg CP ≅ seg CQ .
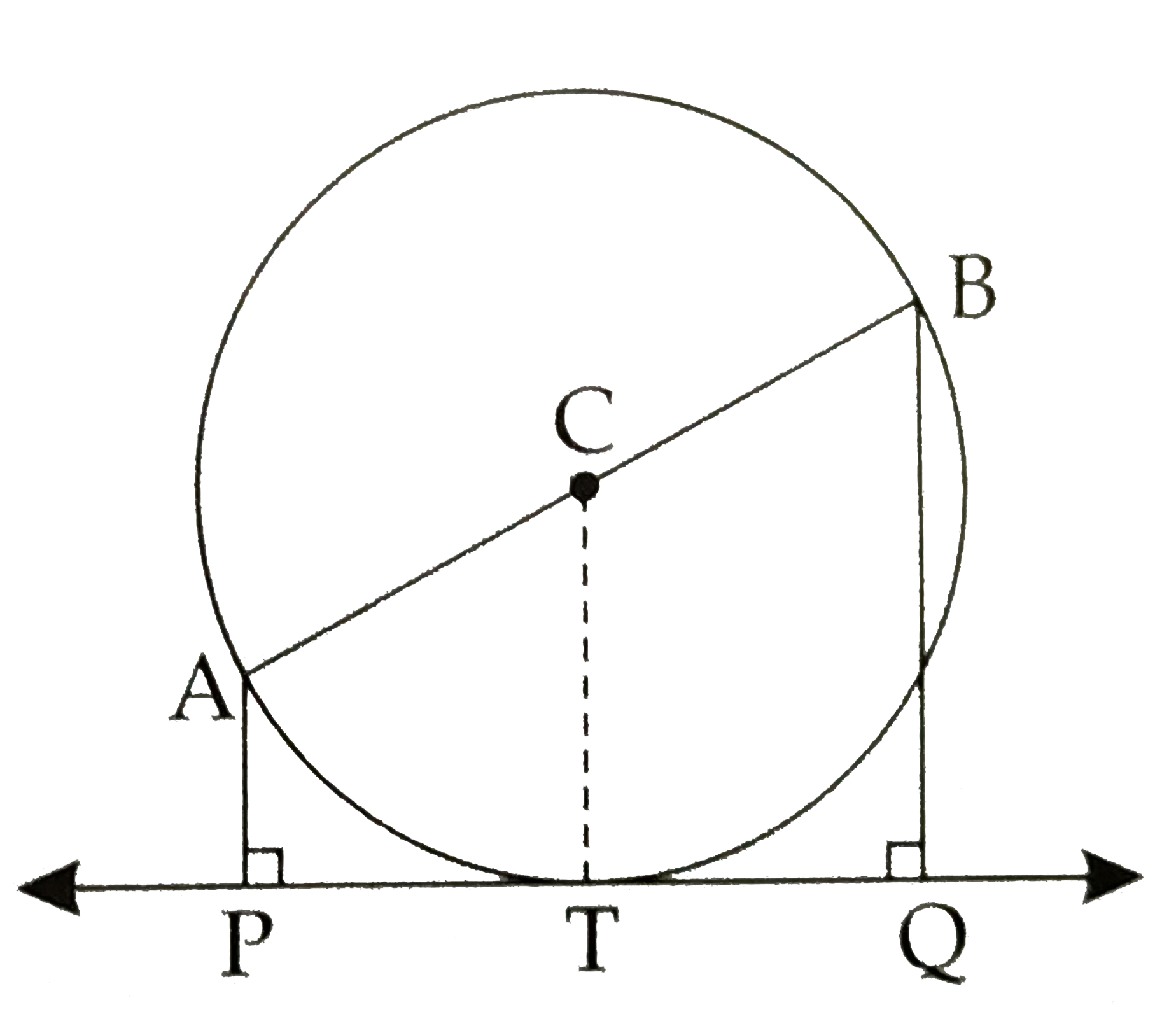
View SolutionIf the tangent at the point (a,b) on the curve x3+y3=a3+b3 meets the curve again at the point (p,q) then
1) ab+pq=0 2) bq+ab+ap=0 3) bp+aq+ab=0 4) bq+pq+ap=0
View SolutionAP and BQ are bisectors of two parallel intersections that are formed from the intersection of the parallel lines l and m by the oblique line t (figure). Show that AP || BQ is.
View Solution
