If ABCD is a square then show that the
points A, B, C and D are concyclic.
The correct Answer is:⇒ C lies on the circle passing through A, B, D.
∴ A, B, C, D are concyclic.
Topper's Solved these Questions
- CIRCLEBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - CIRCLEEXERCISE - EXERCISE - 1(b)
15 Videos
- CIRCLEBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - CIRCLEEXERCISE - EXERCISE - 1(c)
28 Videos
- CIRCLEBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - CIRCLEEXERCISE - EXERCISE - 1(e)
25 Videos
- BINOMIAL THEOREMBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - BINOMIAL THEOREMEXERCISE - TETUAL EXERCISES (EXERCISE - 6(c)) I.
15 Videos
- COMPLEX NUMBERSBOOK - VIKRAM PUBLICATION ( ANDHRA PUBLICATION)CHAPTER - COMPLEX NUMBERSEXERCISE - LAQ
1 Videos
Similar Questions
In the adjoining figure, ABC is a triangle in whcich AB=AC. If D and E are poitns on AB and AC respecrtively such that AD=AE, show that the points B,C,E and D are concyclic.
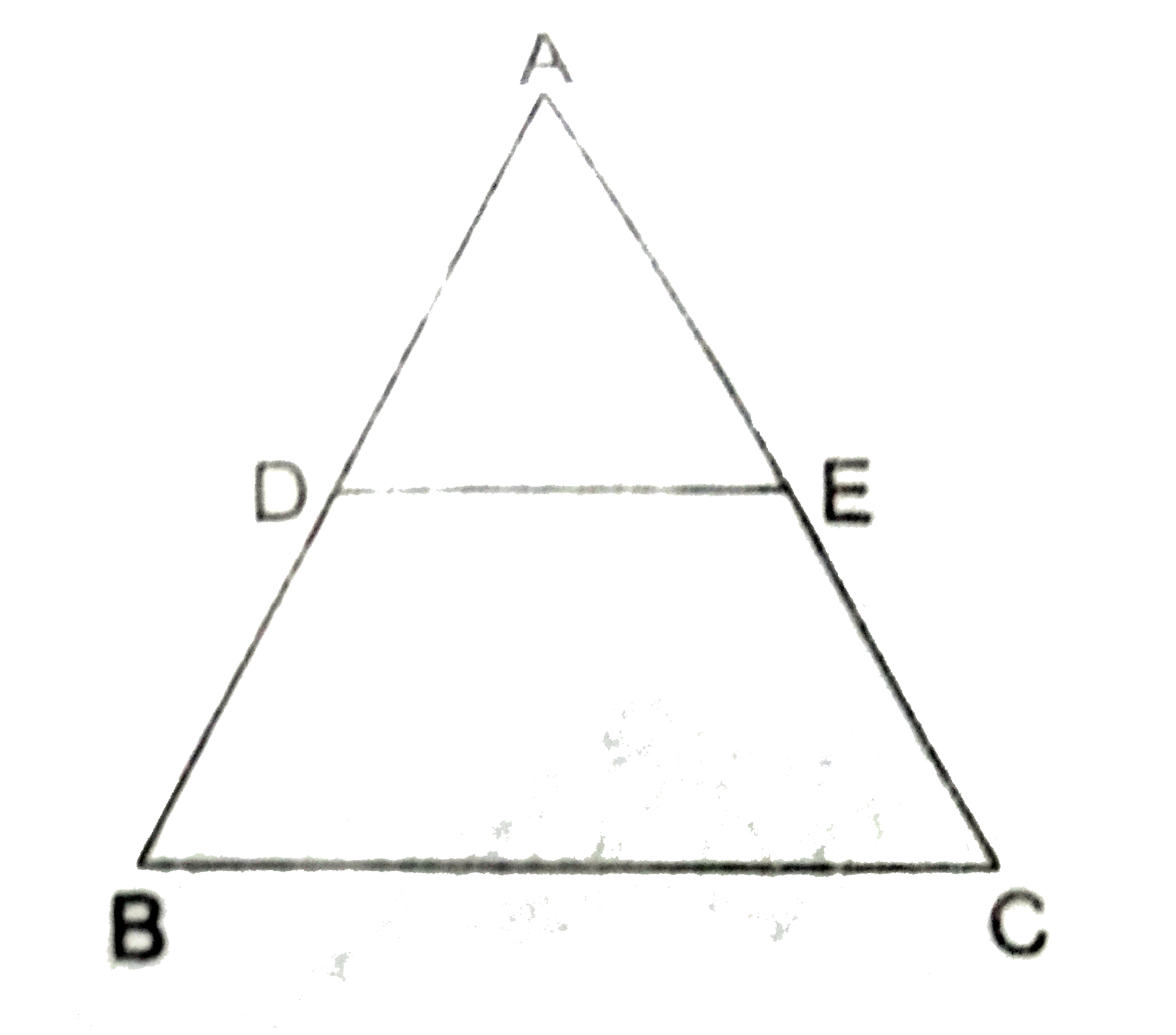
View SolutionIn Figure, ABC is a triangle in which AB=AC. Point DandE are points on the sides ABandAC respectively such that AD=AE. Show that the points B,C,EandD are concyclic.
View SolutionIn Figure, ABC is a triangle in which AB=AC. Point DandE are points onthe sides ABandAC respectively such that AD=AE. Show that the points B,C,EandD are concyclic.
View SolutionABC is an isosceles triangle in which AB=AC. If D and E are the mid-points of AB and AC respectively, prove that the points B, C, D and E are concyclic.
View SolutionIn the given figure, AE = DE and BC || AD. Prove that the points A,B,C and D are concyclic. Also, prove that the diagonals of the quadrilateral ABCD are equal.
View SolutionABC is an isosceles triangle in which AB=AC. If D and E are the mid-points of AB and AC respectively, Prove that the points B, C, D and E are concyclic.
View SolutionProve that the points A(4,0),B(6,1),C(4,3) and D(3, 2) are concyclic.
View SolutionStatement 1 :If the lines 2x+3y+19=0 and 9x+6y−17=0 cut the x-axis at A,B and the y-axis at C,D, then the points, A,B,C,D are concyclic. Statement 2 : Since OAxOB=OCxOD, where O is the origin, A,B,C,D are concyclic.
View SolutionABCD is a rectangle. The line through C perpendicular to AC meets AB produced at X and AD produced at Y. Prove that the points D, B, X, Y are concyclic.
View SolutionABCD is a parallelogram. A circle through A, B is so drawn that it intersects AD at P and BC at Q. Prove that P, Q, C and D are concyclic.
View Solution
