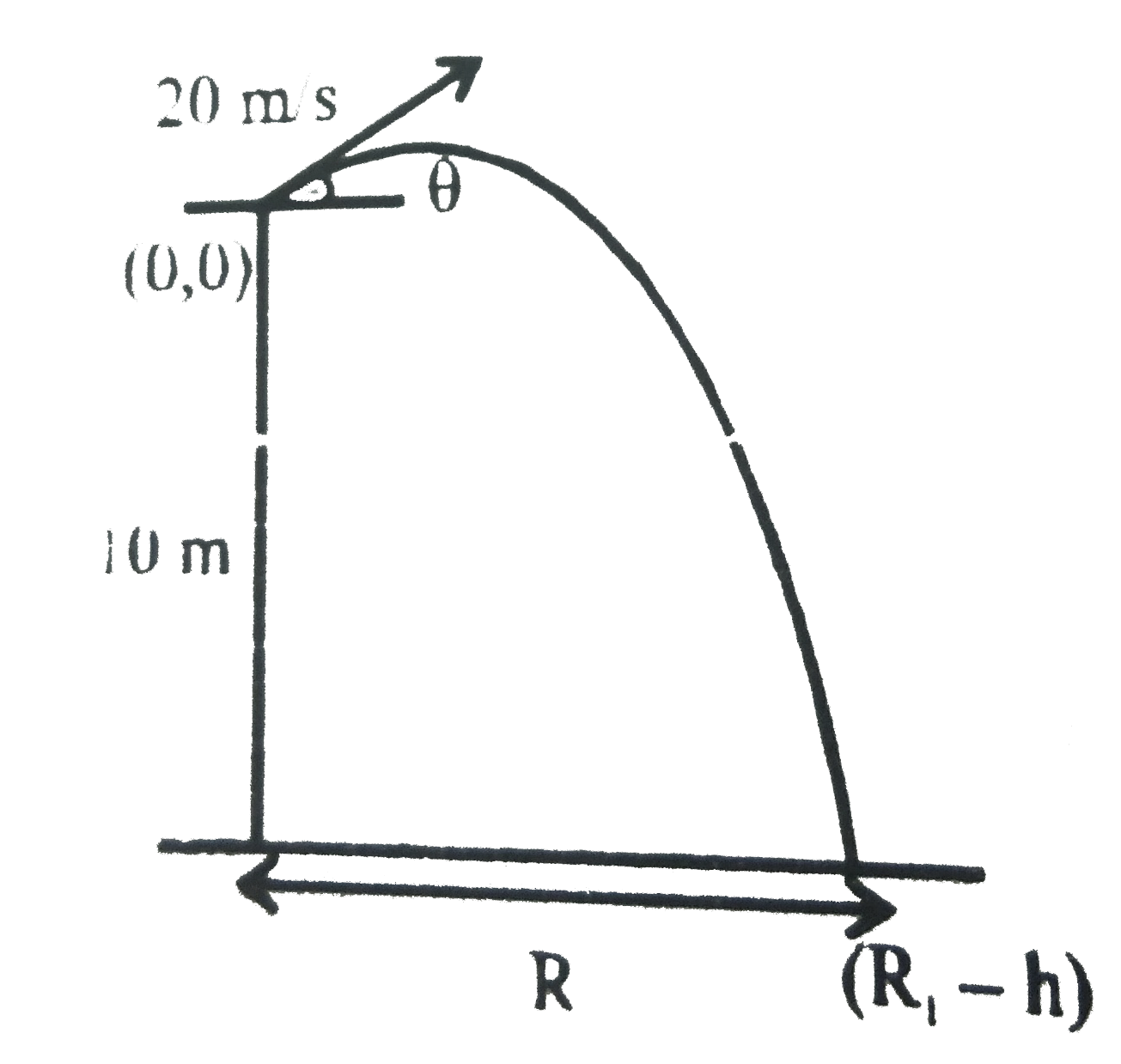
A parojectile thrown from platform at a height 10m above the gorund with velocity of 20m/sec. At what angle should the projectile be thrown to reach the frathest point form O, which is vertcally below the point from which it is thrown.
[g=10m/s2]
The correct Answer is:tan−1(√2/3)
equaiton. of trajectory is y=tanθ.x−12gx2u2cos2θ
y=tanθ.x−12gsec2θx2u2
y=tanθ.x−12g(1+tan2θ)x2u2
2u2y=2u2tanθ.x−g(1+tan2θ)x2
(R,−h) will stisfy the equaiton.of trajectroy.
−2u2h=2u2tanθR−gR2(1+tan2θ)
gR2tan2θ−2u2Rtanθ+gR2−2u2h=0..(1)
For real tanθ from quardatic equation b2−4ac>0
(2u2R)6(2)−4gR2(gR2−2u2h)≥0
u2−g2R2+2u2hg≥0
(u4g2+2u2hg)1/2≥R⇒Rmax=R1=(u4g2+2u2hg)1/2
R1=(u4g2+2u2hg)1/2
⇒R1=[400×400100+2×400×1010]1/2
R1=[1600+800]1/2⇒R1=(2400)1/2
R1=20√6m
substitute the value of R1 in (1) we get on solving
θ=tan−1(√23)
Similar Questions
A projectile thrown from a height of 10 m with velocity of √2m/s the projectile will fall, from the foot of projection, at distance- (g=10m/s2)
View SolutionA stone is thrown up from the top of a tower 20,m with a velocity of 24m/s a tan elevation of 30∘ above the horizontal. Find the horizontal distance from the foot of the tower to the point at which the stone hits the ground. Take g=10m/s2
View Solution
Knowledge Check
A projectile is thrown with an initial velocity of (x→i+y→j)m/s . If the range of the projectile is double the maximum height reached by it, then
Ax−2yBy=2xCx=yDy=4xIf an object is thrown upwards and rises to á height of 10 m. Calculate the velocity with which the object was thrown upwards and time taken by the object to reach the highest point.
A12m/s ,1 .43 sB14 m/s . 1.84 sC14 m/s , 1.43 sD12 m/s , 1.84 sA projectile is thrown with an initial velocity of →v=(pˆi+qˆj) m/s. If the range of the projectile is four times the maximum height reached by it, then
Ap = 2qBq = 4pCq - 2pDq = p
A ball is thrown from the top of a building with a velocity of 10 m/s and returns with a velocity of 30 m/s to the ground. Find the height of the building.
View SolutionA projectile is thrown at angle of 30∘ with a velocity of 10ms .The change in velocity during the time interval in which it reaches the highest point is
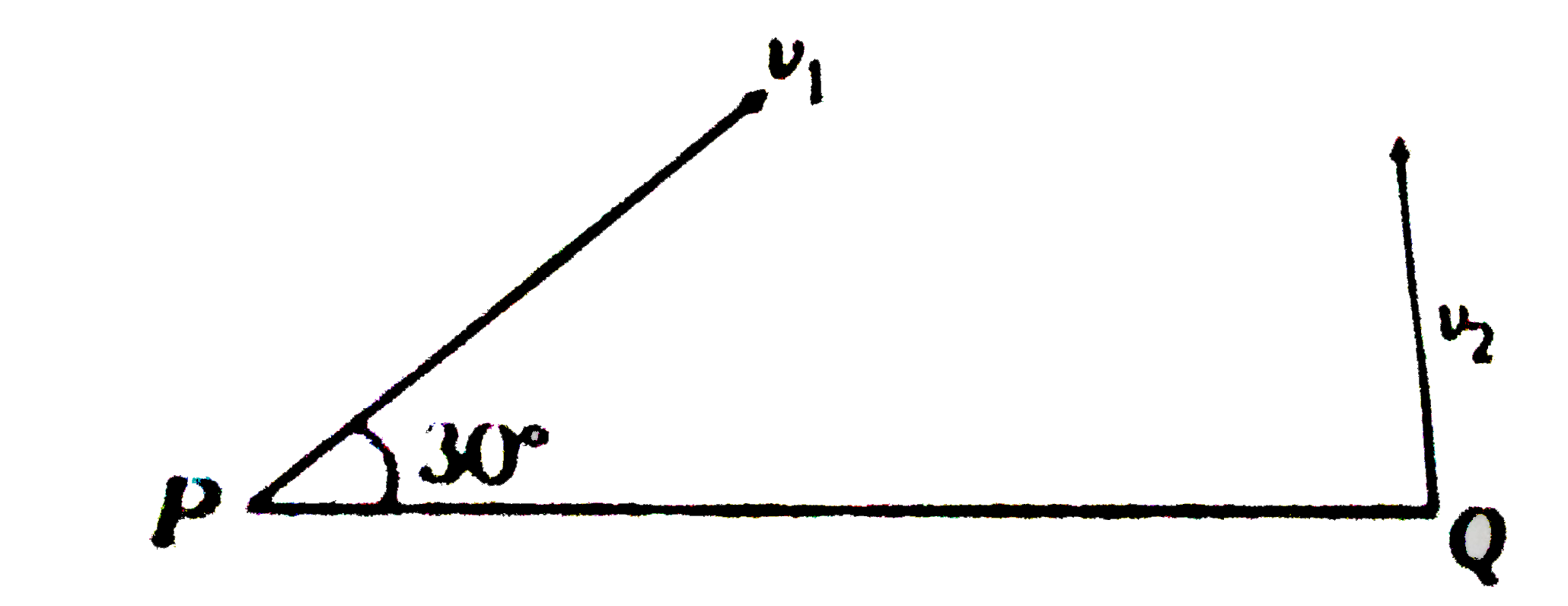
View SolutionA projectile A is thrown at an angle 30∘ to the horizontal from point P. At the same time, another projectile B is thrown with velocity v2 upwards from the point Q vertically below the highest point A would reach. For B to collide with A the ratio v2v1 should be
View SolutionA ball thrown by one player reaches the other in 2 seconds. The maximum height attained by the ball above the point of projection will be :-
(g=10m/s2)
View SolutionA projectile is thrown with a velocity of 20 m/s, at an angle of 60∘ with the horizontal. After how much time the velocity vector will make an angle of 45∘ with the horizontal (in upward direction) is (take g=10m/s2)−
View Solution
