A straight rod of length L is released on a frictionless horizontal floor in a vertical position. As it falls + slips, the distance of a point on the rod from the lower end, which follows a quarter circular locus is
L/2
L/4
L/8
None
The correct Answer is:B
Step by step video, text & image solution for A straight rod of length L is released on a frictionless horizontal floor in a vertical position. As it falls + slips, the distance of a point on the rod from the lower end, which follows a quarter circular locus is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
A rod of length l is released from the vertical position on a smooth horizontal plane. What is the nature of motion of the free end of the rod ?
View SolutionA rod of mass M and length L is kept on a frictionless horizontal floor. A particle of mass m moving perpendicular to the rod with a speed v strikes at the end of the rod and sticks there. Calculate angular velocity acquired by the combined system.
View Solution
Knowledge Check
- Question 1 - Select One
A straight rod of length L is released on a frictionless horizontal floor in a vertical position. As it falls + slips, the distance of a point on the rod from the lower end, which follows a quarter circular locus is
AL/2BL/4CL/8DNone - Question 2 - Select One
A thin rod of length L is vertically straight on horizontal floor. This rod falls freely to one side without slipping of its bottom. The linear velocity of centre of rod when its top end touches floor is
A√2gLB√3gL2C√3gLD√3gL4 - Question 3 - Select One
A rod of length l whose lower end is fixed on a horizontal plane, starts toppling from the vertical position. The velocity of the upper end when it hits the ground is.
A√g/lB√3glC3√g/lD√3g/(l)
A rod of length l is pivoted about a horizontal , frictionless pin through one end. The rod is released from ret in a vertical position. Find the velocity of the CM of the rod when the rod is inclined at an angle θ from the vertical.
 View Solution
View SolutionA uniform rod of length l is kept vertically on a rough horizontal surface at x = 0 . It is rotated slightly and released . When the rod finally falls on the horizontal surface , the lower end will remain at
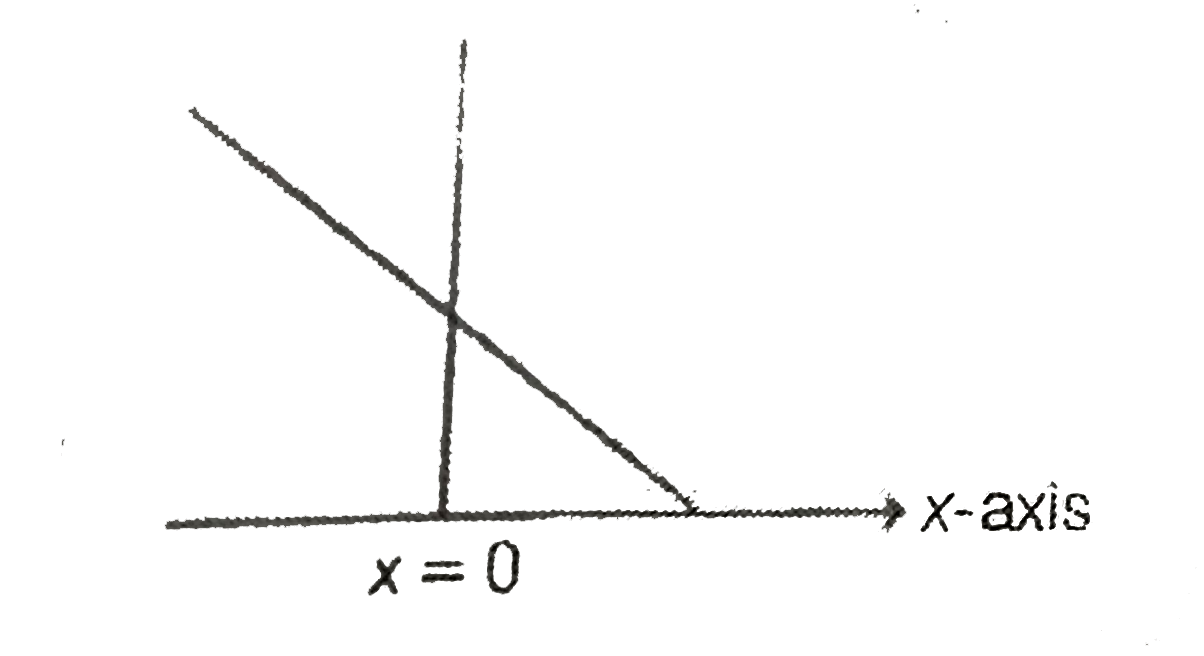 View Solution
View SolutionA rod of mass m and length l is released from rest from vertical position as shown in the figure. The normal force as a function of θ, which is exerted on the rod by the ground as it falls downwards, assuming that it does not slip is mg(3cosθ−1n)2 then n=
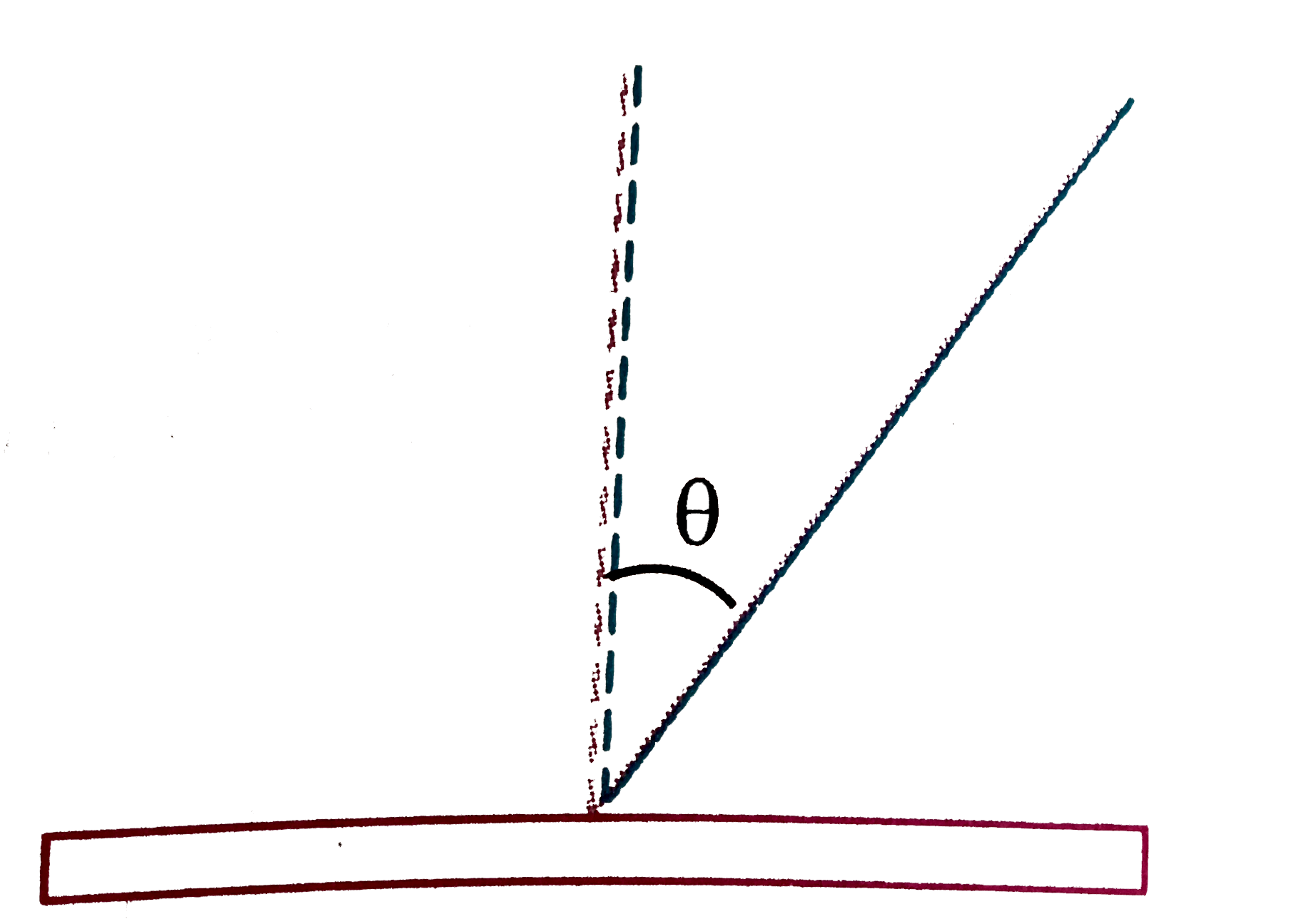 View Solution
View SolutionA rod of length L whose lower end is fixed along the horizontal plane starts to topple from the vertical position. The velocity of the upper end of the rod when it hits the ground is
View SolutionIn the above problem, if the rod is released from horizontal position, the angular velocity of the rod as it passes the vertical position is (l=length fo rod)
View Solution

