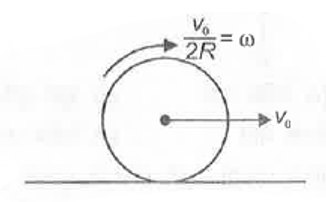
A uniform disk of mass $m$ and radius $R$ is projected horizontally with velocity $v_{0}$ on a rough horizontal floor so that it starts off with a purely sliding motion at $t = 0$ . After $t_{0}$ seconds it acquires a purely rolling motion as shown in figure.
(i) Calculate the velocity of the centre of mass of the disk at $t_{0}$ .

(ii) Assuming the coefficient of friction to be $μ$ calculate $t_{0}$ . Also calculate the work done by the frictional force as a function of time and the total work done by it over a time $t$ much longer than $t_{0}$ .

Text Solution

Verified by Experts

The correct Answer is:(i) $2 v_{0} / 3$ ,
(ii) $t = v / 3 μ g, W = \frac{1}{2} [3 μ^{2} m g^{2} t^{2} - 2 μ m g t v_{0}] (t < t_{0}), W = - \frac{1}{6} m v_{0}^{2} (t > t_{0})$ .

(i) For translational motion of centre of mass
(a) $t \leq t_{0} v = v_{0} - μ g t$ …(1)
For Rotational motion, torque is being provided by fricitonal force.
$μ m g R = I α \Rightarrow μ m g R = \frac{1}{2} m R^{2} α$
$α = \frac{2 μ g}{R}$
so $ω = a t \Rightarrow ω = \frac{2 μ g}{R} t$
at $t = t_{0}, v = R ω$
form (1) & (2)
$v_{0} - μ g t_{0} = 2 μ g t_{0} \Rightarrow t_{0} = \frac{v_{0}}{3 μ g}$
by elimination $t_{0}$ from equation (1) $v = \frac{2 v_{0}}{3}$
For $t < t_{0}$
Work done by friction $= Δ K = K_{f} - K_{i}$
= $(\frac{1}{2} m v^{2} + \frac{1}{2} I ω^{2}) - \frac{1}{2} m v_{0}^{2}$
= $\frac{1}{2} m {(v_{0} - μ g t)}_{0}^{2} + \frac{1}{2} (\frac{1}{2} m R^{2}) {(\frac{2 μ g}{R} t)}^{2} - \frac{1}{2} m v_{0}^{2}$
= $\frac{1}{2} [3 μ^{2} m g^{2} t^{2} - 2 μ m > v_{0}]$
For $t > t_{0}$
$W_{friction} = K_{f} - K_{i}$
$W_{friction} = \frac{1}{2} m v^{2} (1 + \frac{K^{2}}{R^{2}}) - \frac{1}{2} m v_{0}^{2}$
$W_{friction} = \frac{1}{2} m v^{2} \times \frac{3}{2} - \frac{1}{2} m v_{0}^{2}$ By putting $v = \frac{2 v_{0}}{3}$
$W_{friction} = - \frac{m v_{0}^{2}}{6}$ .

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Similar Questions

Knowledge Check

Question 1 - Select One
A uniform ring of mass 'm' and radius 'R' is projected horizontally with velcoty $v_{0}$ on a rough horizontal floor, so that it starts off with a purely sliding motion and it acquires a pure rolling motion after moving a distance d. If the coefficient of friction between the ground and ring is $μ$ , then work done by the friction in the process is
A $- μ m g d$
B $- \frac{1}{4} m v_{0}^{2}$
C $μ m g d$
D $- \frac{1}{8} m v_{0}^{2}$
Question 2 - Select One

A disc of mass $M$ and radius $R$ is rolling purely with centre's velcity $v_{0}$ on a flat horizontal floor when it hits a step in the floor of height $R / 4$ The corner of the step is sufficiently rough to prevent any slippoing of the disc against itself. What is the velocity of the centre of the disc just after impact?
A $4 v_{0} / 5$
B $4 v_{0} / 7$
C $5 v_{0} / 6$
Dnone of these

Recommended Questions

Home
Ask Doubt
Profile