Given a battery, how would you connect two capaitors, it series or in paralllel for them to store the greater (a) total charge (b) total energy ?
Total charge q=CV and total energy U=12CV2
Now, V is constant and Cp>Cs,
therefore, parallel combination is required for strong greater charge and greater energy.
Similar Questions
A battery of 10V is connected to a capacitor of capacity 0.lF. The battery is now removed and this capacitor is connected to a second uncharged capacitor. If the charges are distributed equally on these two capacitors, find the total energy stored in the two capacitors. Find the ratio of final energy to initial energy stored in capacitors.
View SolutionRahul connected the two wires in series and observe that total resistance increases. Justify.
View Solution
Knowledge Check
Two capcitors, 3 μF and 4μF, are individually charged across a 6 V battery. After being disconnected from the battery, they are connected together with the negative plate of one attached to the positive plate of the other. What is the final total energy stored ?
A1.26×10−4JB2.57×10−4JC1.25×10−6JD2.57×10−6JThe enrgy of a charged capacitor is U. Another identical capacitor is connected parallel to the first capacitor, after disconnecting the battery. The total energy of the system of these capacitors will be
AU4BU2C3U2D2U4
The figure below shows a battery of emf ε connected to an inductor L and resistance R in series. If the switch is closed at t=0, then the total charge that flows from the battery in time constant of the circuit is
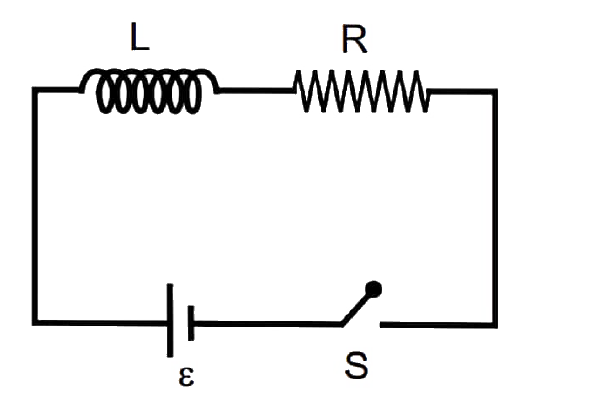
View SolutionYou have two identical capacitors and an extermal potential source.
a. Compare the total energy stored in capaciton when they are connected to the applied potential in series and in parallel.
b. Compare the maximum amount of charge stored in each case.
c. Energy storage in a capacitor can be limited by the maximum electric field between the plates. What is the ratio of the electric field for the series for parallel combinations?
View SolutionA capacitor of capacitance C1 is charged to a potential V1 while another capacitor of capacitance C2 is charged to a potential difference V2 . The capacitors are now disconnected from their respective charging batteries and connected in parallel to each other .
(a) Find the total energy stored in the two capacitors before they are connected.
(b) Find the total energy stored in the parallel combination of the two capacitors.
(c ) Explain the reason for the difference of energy in parallel combination in comparison to the total energy before they are connected.
View SolutionA capacitor of capacitance C1 is charged to a potential V1 , while another capacitor of capacitance C2 is charged to a potential difference V2 . The capacitors are now disconnected from their respective charging batteries and connected in parallel to each other
Find the total energy stored in the parallel combination of two capacitors.
View SolutionSuppose the initial charge on the capacitor in Exercise 7.7 is 6 mC. What is the total energy stored in the circuit initially ? What is the total energy at later time?
View SolutionA capacitor is charged using a battery and energy stored is U. After disconnecting the battery, another uncharged capacitor of same capacitance is connected in parallel to the first capacitor. Then loss of energy is equal to
View Solution
