Consider a sphere of radius R with charge density distributed as $ρ (R) = k r$ for $r \leq R and = 0$ for $r > R$ .
(a) Find the electric field at all points r.
(b) suppose the total charge on the sphere is 2e, where e is the electron charge. Where can two protons be embedded such that the force on each of them is zero. Assume that the introduction of the proton does not alter the negative charge distribution.

Video Solution

Text Solution

Verified by Experts

The given charge density distribution of the sphere fo radius R is
$ρ (r) = k r$ for $r \leq R$
$= 0$ for $r > R$
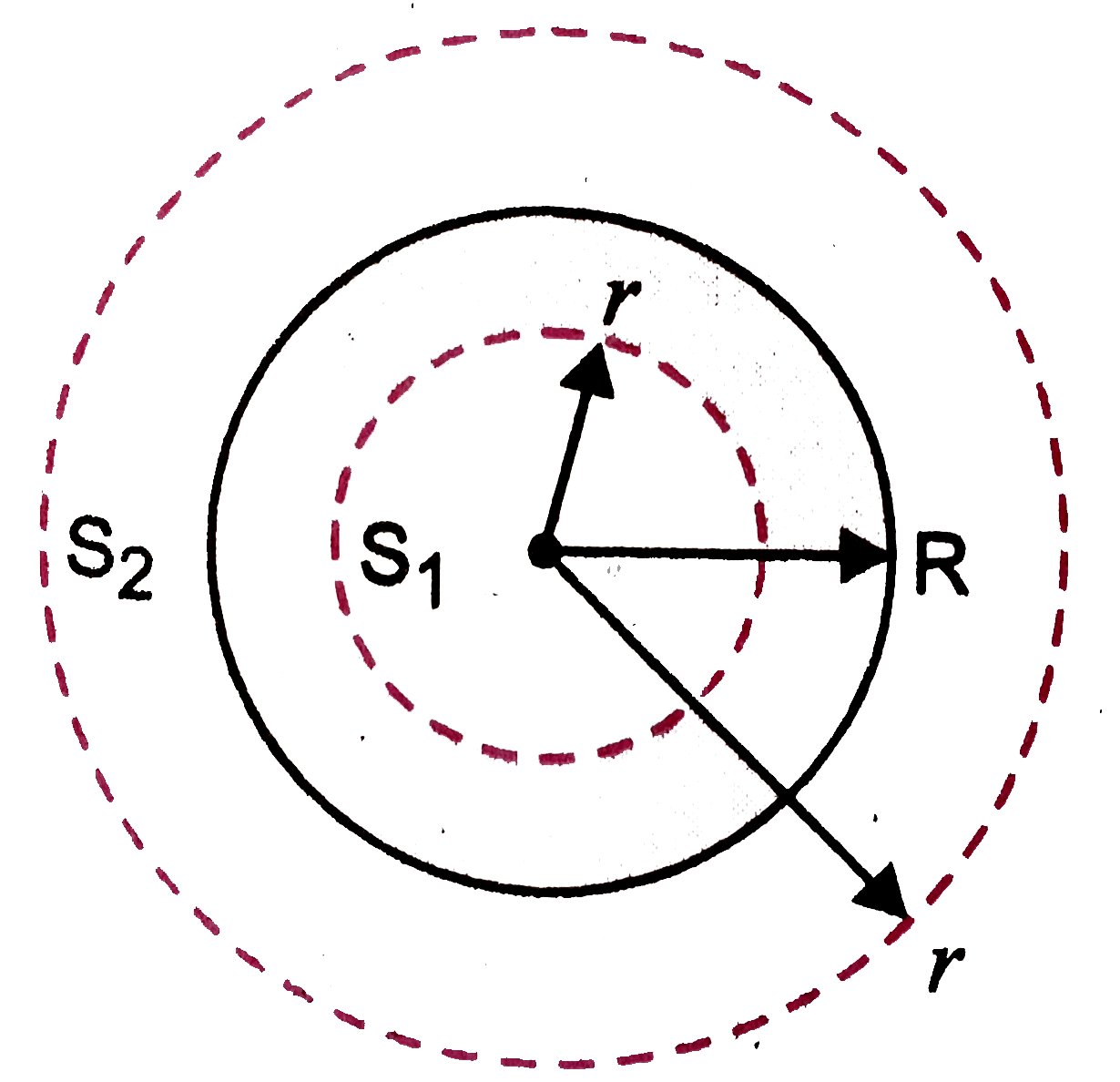
The electric field is obviously radial, as shown in Fig.
For points $r < R$
Let us consider a spherical Gaussian surface $S_{1}$ of radius r. Then on the surface,
$\oint \to E . \to d s = \frac{1}{\in_{0}} \int ρ d V$
As $V = \frac{4}{3} π r^{3}, d V = \frac{4}{3} π 3, r^{2} d r = 4 π r^{2} d r and ρ (r) = k r ∴ \oint \to E . \to d s = \frac{1}{\in_{0}} 4 π k \int_{0}^{r} r r^{2} d r$
$(E) 4 π r^{2} = \frac{4 π k}{\in_{0}} \frac{r^{4}}{4}$
or $E = \frac{1}{4 \in_{0}} k r^{2}$ ...(i)
The direction of $\to E$ is radially outwards (for positive charge density)
For points $r > R$ , let us consider a sphericall Gaussian surface $S_{2}$ of radius r. Then on the surface, when $r = R$
$\oint \to E . \to d s = \frac{1}{\in_{0}} \int ρ . d V$
$E (4 π r^{2}) = \frac{4 π k}{\in_{0}} \int_{0}^{R} r^{3} d r = \frac{4 π k}{\in_{0}} d r = \frac{4 π k}{\in_{0}} \frac{R^{4}}{4}$
or $E = \frac{k}{4 \in_{0}} \frac{R^{4}}{r^{2}}$
The direction of $\to E$ is radially outwards (for positive charge density).
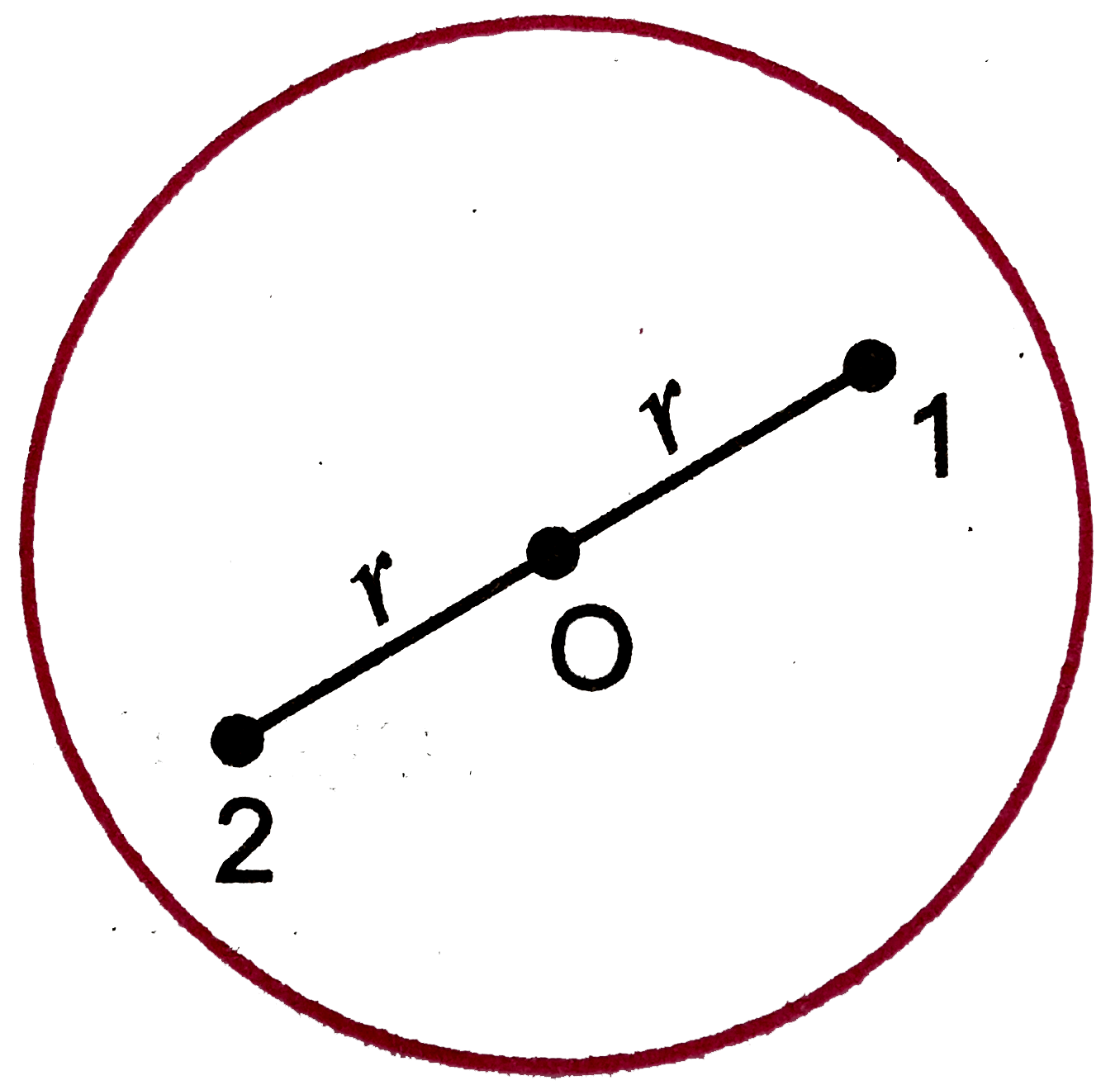
(b) From symmetry, we find that the two protons must be on the opposite sides of the center, along a diameter of the sphere as shown in Fig. Proceeding as above, charge on the sphere.
$q = \int_{0}^{R} ρ d V = \int_{0}^{R} (k r) 4 π r^{2} d r = 4 π k \frac{R^{4}}{4} = 2 e$
$∴ k = \frac{2 e}{π R^{4}}$ ....(iii)
If protons 1 and 2 are embedded at distance r from the center of the sphere as shown , then attractive force on proton 1 due to charge distribution is
$F_{1} = - e E = - e \frac{k r^{2}}{4 \in_{0}}$ ....using (i)
Repulsive force on proton 1 due to proton 1 due to proton 2 is $F_{2} = \frac{e^{2}}{4 π \in_{0} {(2 r)}^{2}}$
Net force on proton 1 $F = F_{1} + F_{2} = - e \frac{k r^{2}}{4 \in_{0}} + \frac{e^{2}}{16 π \in_{0} r^{2}}$
Using (iii), $F = [- \frac{e r^{2}}{4 \in_{0}} \frac{2 e}{π R^{4}} + \frac{e^{2}}{16 π \in_{0} r^{4}}]$
This net force on proton 1 will be zero, when $\frac{e r^{2} . 2 e}{4 \in_{0}} = \frac{e^{2}}{16 π \in_{0} r^{2}} or r^{4} = \frac{R^{4}}{8} or r = \frac{R}{{(8)}^{1 / 4}}$
This is the distance of each of the two protons from the center of the sphere.

Updated on:21/07/2023

Let $ρ (r) = \frac{Q r}{π R^{4}}$ be the charge density distribution for a solid sphere of radius $R$ and total charge $Q$ . For a point $P$ inside the sphere at a distance $r_{1}$ from the centre of the sphere, the magnitude of electric field is
A $\frac{Q r_{1}^{2}}{4 π ε_{0} R^{4}}$
B $\frac{Q r_{1}^{2}}{3 π ε_{0} R_{4}}$
C0
D $\frac{Q}{4 i ε_{0} r_{1}^{2}}$
Let $ρ (r) = \frac{Q r}{π R^{4}}$ be the charge density distribution for a solid sphere of radius R and total charge Q. For a point P inside the sphere at a distance $r_{1}$ from the centre of the sphere, the magnitude of electric field is
A $\frac{Q}{4 π ε_{0} r_{1}^{2}}$
B $\frac{Q r_{1}^{2}}{4 π ε_{0} R^{4}}$
C $\frac{Q r_{1}^{2}}{3 π ε_{0} R^{4}}$
Dzero
Let $ρ (r) = \frac{Q r}{π R^{4}}$ be the charge density distribution for a soild sphere of radius R and total charge Q. For a point P inside the sphere at a distance $r_{1}$ from the centre of the sphere, the magnitude of electric field is
A $\frac{Q}{4 π ε_{0} r_{1}^{2}}$
B $\frac{Q r_{1}^{2}}{4 π ε_{0} R^{4}}$
C $\frac{Q r_{1}^{2}}{3 π ε_{0} R^{4}}$
Dzero