Two charges +0.2μμCand−0.2μμC are placed 10−6cm apart. Calculate electric field at an axial point at a distance of 10 cm from their middle point.
The correct Answer is:3.6×10−8N/C
To calculate the electric field at an axial point at a distance of 10 cm from the midpoint of two charges +0.2μC and −0.2μC that are placed 10−6cm apart, we can follow these steps:
Step 1: Understand the Configuration
We have two charges:
- Charge q1=+0.2μC=0.2×10−6C
- Charge q2=−0.2μC=−0.2×10−6C
The distance between the charges d=10−6cm=10−8m.
The distance from the midpoint of the charges to the point where we want to calculate the electric field is r=10cm=0.1m.
Step 2: Calculate the Dipole Moment
The dipole moment p is given by the formula:
p=q⋅d
where q is the magnitude of one of the charges and d is the separation between the charges.
Substituting the values:
p=(0.2×10−6C)⋅(10−8m)=2×10−14C⋅m
Step 3: Use the Formula for Electric Field of a Dipole
The electric field E at a point on the axial line of a dipole is given by the formula:
E=2kpr3
where k is Coulomb's constant, k=9×109N⋅m2/C2.
Step 4: Substitute the Values into the Formula
Now substituting the values into the electric field formula:
E=2⋅(9×109)⋅(2×10−14)(0.1)3
Calculating (0.1)3:
(0.1)3=0.001=10−3
Now substituting:
E=2⋅(9×109)⋅(2×10−14)10−3
Step 5: Simplify the Expression
Calculating the numerator:
2⋅(9×109)⋅(2×10−14)=36×10−5=3.6×10−4
Now substituting back into the electric field equation:
E=3.6×10−410−3=3.6×101=36N/C
Final Result
Thus, the electric field at the axial point at a distance of 10 cm from the midpoint of the dipole is:
E=36N/C
We have two charges:
- Charge q1=+0.2μC=0.2×10−6C
- Charge q2=−0.2μC=−0.2×10−6C
The dipole moment p is given by the formula:
p=q⋅d
where q is the magnitude of one of the charges and d is the separation between the charges.
p=(0.2×10−6C)⋅(10−8m)=2×10−14C⋅m
The electric field E at a point on the axial line of a dipole is given by the formula:
E=2kpr3
where k is Coulomb's constant, k=9×109N⋅m2/C2.
Now substituting the values into the electric field formula:
E=2⋅(9×109)⋅(2×10−14)(0.1)3
(0.1)3=0.001=10−3
E=2⋅(9×109)⋅(2×10−14)10−3
Calculating the numerator:
2⋅(9×109)⋅(2×10−14)=36×10−5=3.6×10−4
E=3.6×10−410−3=3.6×101=36N/C
Thus, the electric field at the axial point at a distance of 10 cm from the midpoint of the dipole is:
E=36N/C
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - FILL IN THE BLANKS8videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - PROBLEMS FOR PRACTICE8videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTROSTATICSEXERCISE - VALUE BASED QUESTIONS7videos
ELECTROSTATICS
- BOOK - PRADEEPCHAPTER - ELECTRONIC DEVICESEXERCISE - Fill in the Blanks1videos
ELECTRONIC DEVICES
- BOOK - PRADEEPCHAPTER - MAGNETIC EFFECT OF CURRENT AND MAGNETISMEXERCISE - Competition Focus (Multiple Choice Questions)2videos
MAGNETIC EFFECT OF CURRENT AND MAGNETISM
Similar Questions
Two charges, each of 5μC but opposite in sign, are placed 4 cm apart. Calculate the electric field intensity of a point that is a distance 4 cm from the mid point on the axial line of the dipole.
View SolutionTwo charges, one +5μC and another −5μC are placed 1 mm apart. Calculate the dipole moment.
View Solution
Knowledge Check
- Question 1 - Select One
Two charges +5μC and +10μC are placed 20cm apart. The net electric field at the mid-point between the two charges is
A4.5×106N/C directed towards +5μCB4.5×106N/C directed towards +10μCC13.5×106N/C directed towards +5μCD13.5×106N/C directed towards +10μC - Question 2 - Select One
Two positive charges of magnitude 4C and 6C are placed 10cm apart . The electric potential at a distance of 10cm from the middle point on the right bisector of the line,joining the two charges is
A5×1011VB4×109VC8×1011VD5×109V - Question 3 - Select One
Two positive charges of 1mu Cand2 mu C are placed 1 metre apart. The value of electric field in N/C at the middle point of the line joining the charge will be :
A10.8×104B3.6×104C1.8×104D5.4×104
Two charges, one +5μC and another −5μC are placed 1 mm apart. Calculate the dipole moment.
View SolutionTwo charges ±10μC are placed 5⋅0mm apart. Determine the electric field at (a) point P on the axis of dipole 15cm away from its center on the side of the positive charge. As shown in Figure and at (b) a point Q. 15cm away form O on a line passing through O and a line passing through O and normal to the axis of the dipole as shown in Fig.
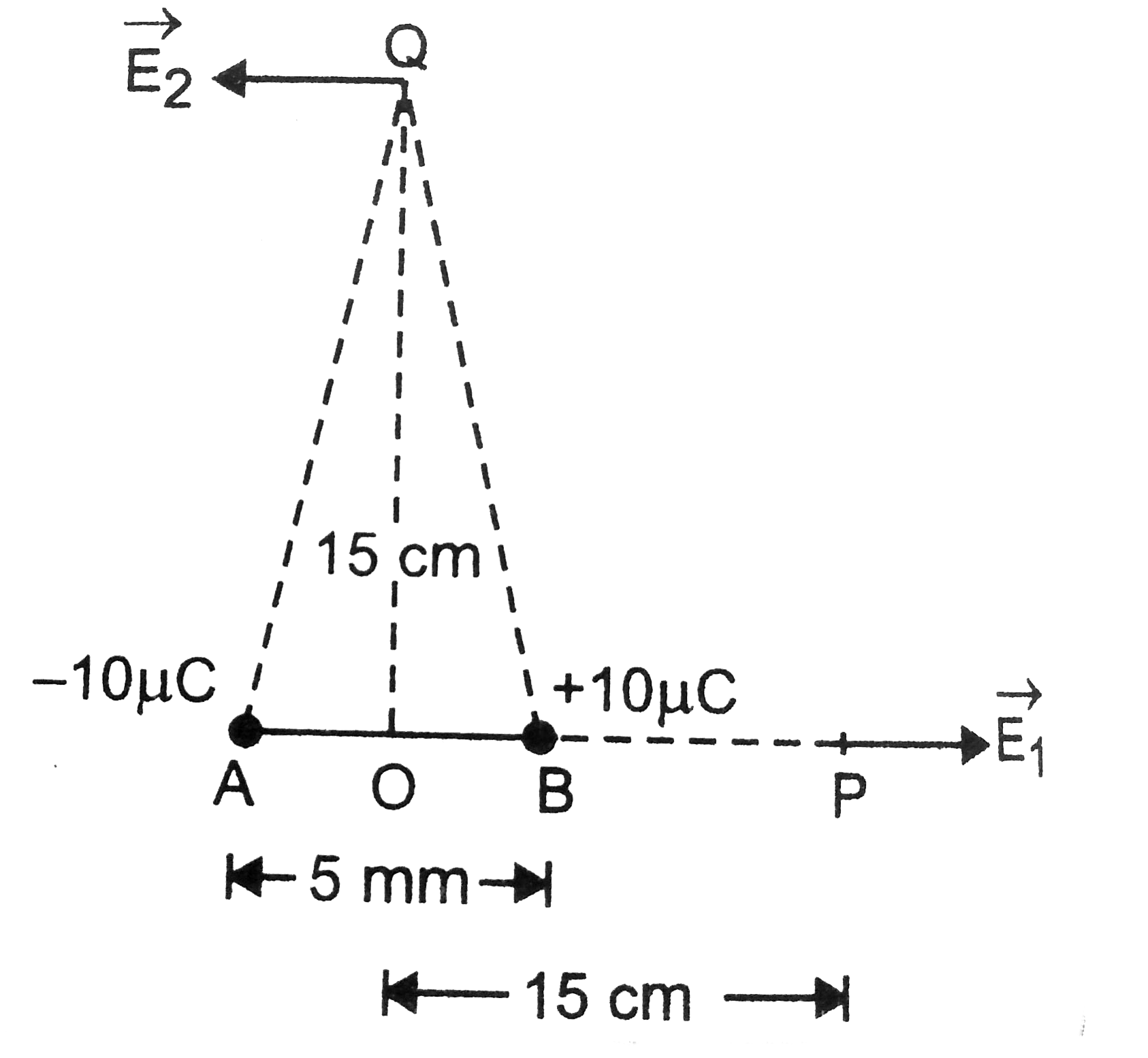 View Solution
View SolutionTwo charges +20μC and −20μC are 5 mm apart. Find the electric field on axial as well as on the equatorial line at a distance 20 cm from the mid -point of the dipole.
View SolutionA and B are two points separated by a distance 5cm.Two charges 10μC and 20μC are placed at A and B.The resultant electric intensity at a point P outside the charges at a distance "5cm from 10μC is
View SolutionA and "B" are two points separated by a distance "5cm" Two charges 10μC and 20μC are placed at "A" and "B" .The resultant electric intensity at a point "P" outside the charges at a distance "5cm" from "10μC" is
View Solution

