In a Wheatstone bridge circuit, P=5Ω,Q=6Ω,R=10Ω and S=5Ω. Find the additional resistance to be used in series with S, so that the bridge is balanced.
To solve the problem of finding the additional resistance X to be used in series with S in a Wheatstone bridge circuit, we can follow these steps:
1. Understand the Wheatstone Bridge Condition:
The Wheatstone bridge is balanced when the ratio of the resistances in one branch is equal to the ratio of the resistances in the other branch. The condition for balance can be expressed as:
S+XR=QP
where S is the resistance in series with X, R is the other resistance in that branch, Q is the resistance in the other branch, and P is the resistance in series with Q.
2. Substitute the Given Values:
We have the following values:
- P=5Ω
- Q=6Ω
- R=10Ω
- S=5Ω
Substitute these values into the balance condition:
5+X10=65
3. Cross-Multiply to Solve for X:
Cross-multiplying gives:
5+X=10⋅65
Simplifying the right side:
5+X=12
4. Isolate X:
Now, isolate X by subtracting 5 from both sides:
X=12−5
X=7Ω
Final Answer:
The additional resistance X to be used in series with S so that the bridge is balanced is 7Ω.
The Wheatstone bridge is balanced when the ratio of the resistances in one branch is equal to the ratio of the resistances in the other branch. The condition for balance can be expressed as:
S+XR=QP
where S is the resistance in series with X, R is the other resistance in that branch, Q is the resistance in the other branch, and P is the resistance in series with Q.
We have the following values:
- P=5Ω
- Q=6Ω
- R=10Ω
- S=5Ω
5+X10=65
Cross-multiplying gives:
5+X=10⋅65
Simplifying the right side:
5+X=12
Now, isolate X by subtracting 5 from both sides:
X=12−5
X=7Ω
The additional resistance X to be used in series with S so that the bridge is balanced is 7Ω.
Topper's Solved these Questions
- BOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Conceptual Problems3videos
CURRENT ELECTRICITY
- BOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Very short Q/A7videos
CURRENT ELECTRICITY
- BOOK - PRADEEPCHAPTER - COMMUNICATION SYSTEMSEXERCISE - MODEL TEST PAPER-29videos
COMMUNICATION SYSTEMS
- BOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise191videos
DUAL NATURE OF RADIATION AND MATTER
Similar Questions
In a Wheatstone's network, P=2Ω,Q=2Ω,R=2ΩandS=3Ω. The resistance with which S is to be shunted in order that the bridge may be balanced is
 View Solution
View SolutionThe resistance of the four arms of a Wheatstone bridge are P=10Ω , Q = 100Ω, R=40Ω and S =10Ω. What resistance in series or parallel with the last one will be required to obtain no deflection in the galvanometer?
View Solution
Knowledge Check
- Question 1 - Select One
In the Wheatstone's bridge shown, P=2Ω,Q=3Ω,R=6Ω and S=8Ω. In order to obtain balance, shunt resistance across S must be
 A2ΩB3ΩC6ΩD8Ω
A2ΩB3ΩC6ΩD8Ω - Question 2 - Select One
In a Wheatstone's network, P=2Ω,Q=2Ω,R=2ΩandS=3Ω. The resistance with which S is to be shunted in order that the bridge may be balanced is
 A1ΩB2ΩC4ΩD6Ω
A1ΩB2ΩC4ΩD6Ω - Question 3 - Select One
If P=Q=R=10ΩandS=20Ω, then what resistance should be joined with S to balance the wheatstone's network ?
AJoin a resistance of 10Ω in series with SBJoin a resistance of 10Ω in parallel with SCJoin a resistance of 20Ω in parallel with SDJoin a resistance of 20Ω in series with S
In the Wheatstone bridge network ABCD is balanced when P=500Ω,Q=250Ω and S=12Ω, what is the value of R ?
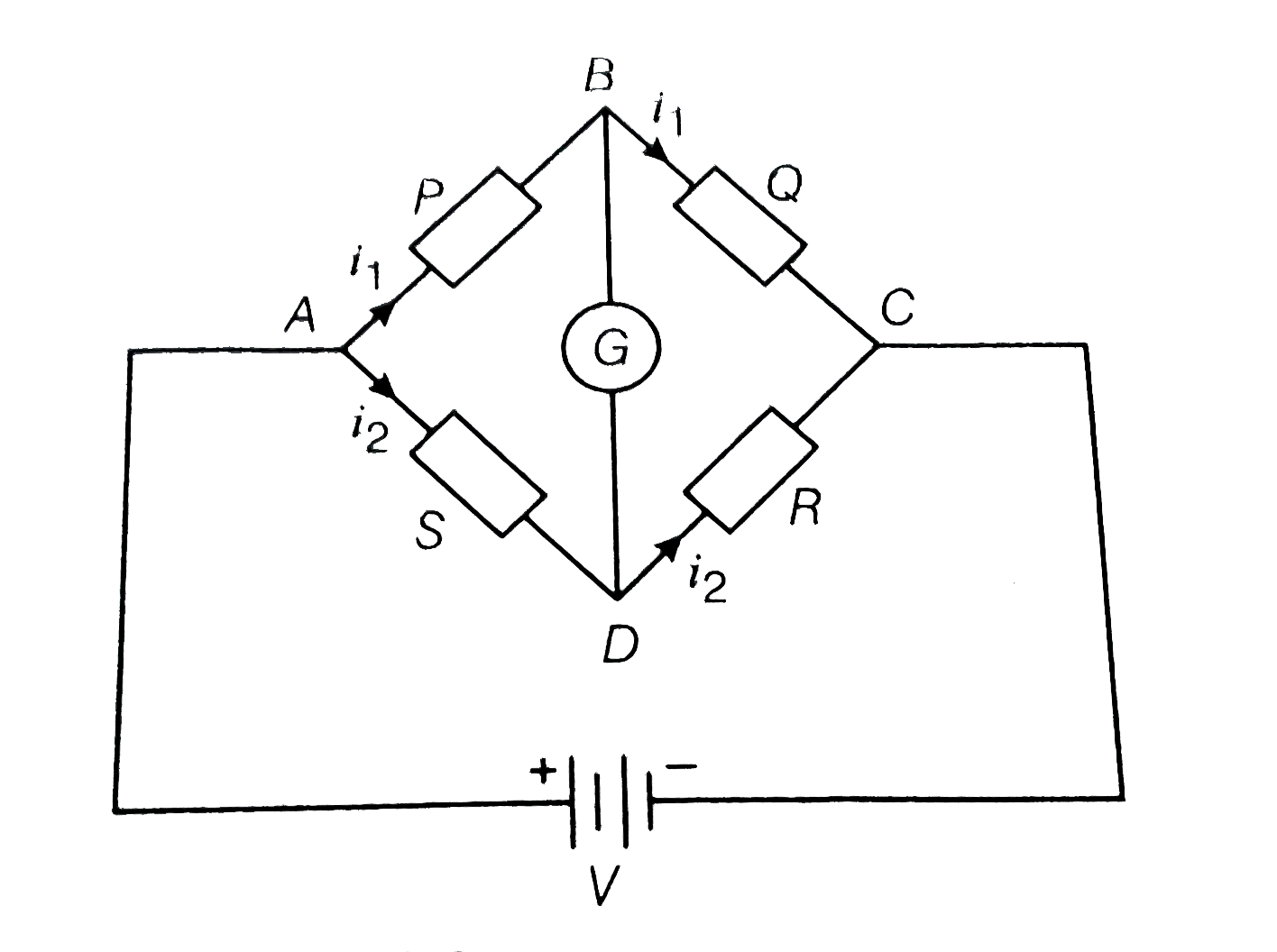 View Solution
View SolutionThe resistances of the four arms P, Q, R and S in a Wheatstone's bridge are 10Ω,30Ω,30Ω and 90Ω, respectively. The emfs and internal resistances of the cell are 7V and 5Ω respectively. If the galvanometer resistance is 50Ω, the current drawn from the cell will be
View SolutionIn Wheatstone's bridge P=9ohm, Q=11ohm, R=4ohm and S=6ohm. How much resistance must be put in parallel to the resistance S to balance the bridge
View SolutionA balanced wheat stone bridge has resistance P=10Ω,Q=20ΩR=5Ω,S=11Ω,ε=48VandC is grounded
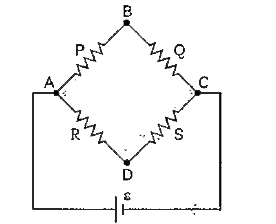
Potential of point B isView SolutionFour resistances arranged to form a Wheatstone's network are 8Ω,12Ω,6Ω, and 27Ω. The resistance that should be connected across 27Ω resistance to balance the bridge is
View Solution

