State Wheatstone bridge principle and deduce it using Kirchhoff's rules.

Video Solution

Text Solution

Generated By DoubtnutGPT

Step-by-Step Solution
Step 1: State the Wheatstone Bridge Principle
The Wheatstone Bridge principle states that a Wheatstone bridge is in equilibrium when the ratio of the resistances in one branch is equal to the ratio of the resistances in the other branch. Mathematically, this can be expressed as:
$\frac{R_{1}}{R_{2}} = \frac{R_{4}}{R_{3}}$
where $R_{1}$ and $R_{2}$ are the resistances in one branch, and $R_{3}$ and $R_{4}$ are the resistances in the other branch.
Step 2: Set Up the Circuit
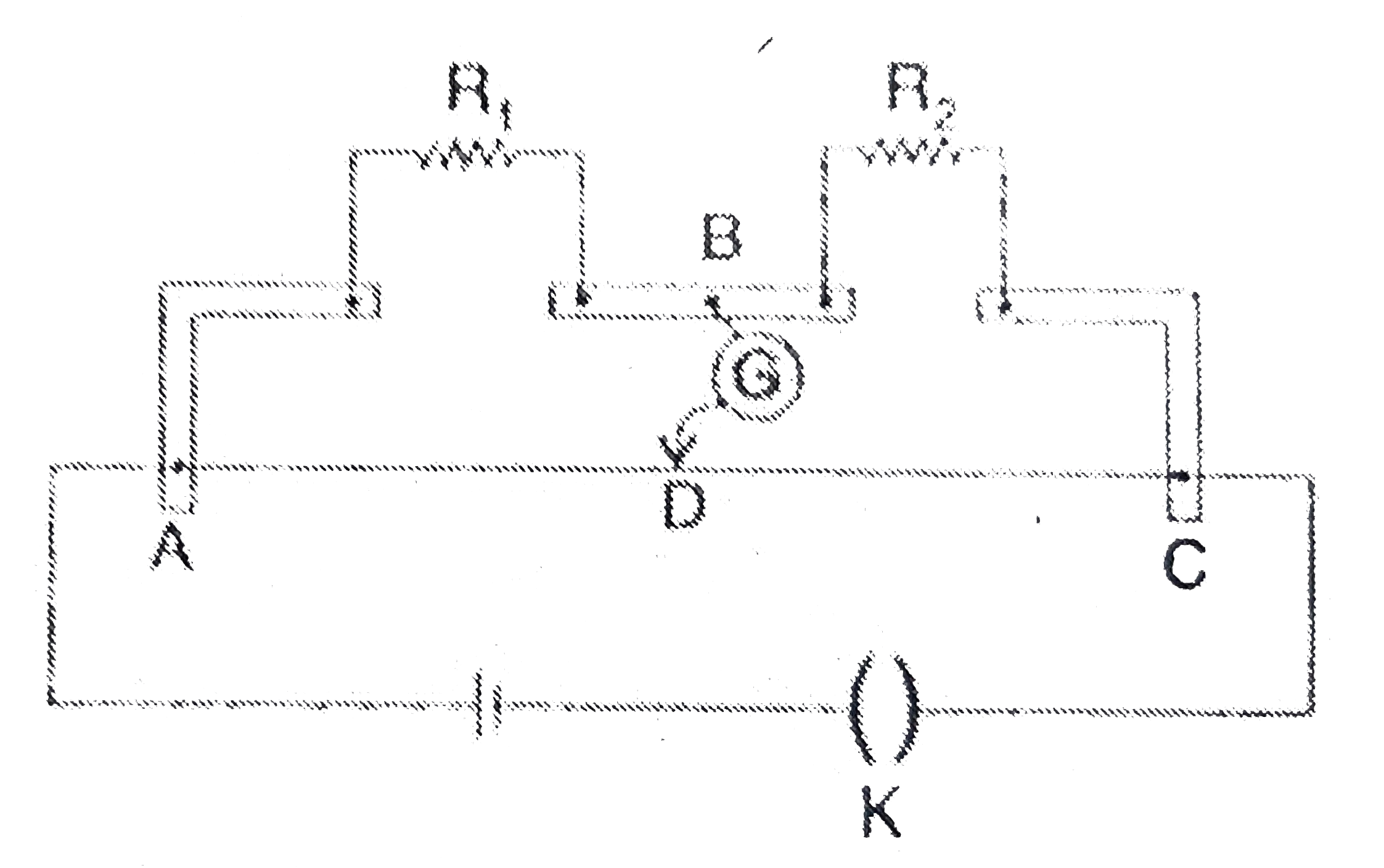
Consider a Wheatstone bridge circuit with resistances $R_{1}$ , $R_{2}$ , $R_{3}$ , and $R_{4}$ connected to a battery of emf $E$ . A galvanometer with resistance $r$ is connected between the junctions of $R_{1}$ and $R_{2}$ and the junctions of $R_{3}$ and $R_{4}$ .
Step 3: Apply Kirchhoff’s Laws
To analyze the circuit, we will apply Kirchhoff's Voltage Law (KVL). Assume the current entering the circuit is $I$ , which splits into two branches: $I_{1}$ through $R_{1}$ and $R_{2}$ and $I_{2}$ through $R_{3}$ and $R_{4}$ .
Step 4: Write KVL for the Loops
1. For the loop containing $R_{1}$ and $R_{2}$ :
$I_{1} R_{1} + I_{2} R_{2} - E = 0$
Rearranging gives:
$I_{1} R_{1} + I_{2} R_{2} = E (1)$
2. For the loop containing $R_{3}$ and $R_{4}$ :
$I_{2} R_{3} + I_{1} R_{4} = 0$
Rearranging gives:
$I_{2} R_{3} + I_{1} R_{4} = 0 (2)$
Step 5: Set the Condition for No Current in the Galvanometer
For the bridge to be balanced, the current through the galvanometer must be zero. This means:
$I_{1} R_{2} = I_{2} R_{3} (3)$
Step 6: Substitute and Solve
From equation (3), we can express $I_{2}$ in terms of $I_{1}$ :
$I_{2} = \frac{I_{1} R_{2}}{R_{3}}$
Substituting this into equation (1):
$I_{1} R_{1} + (\frac{I_{1} R_{2}}{R_{3}}) R_{2} = E$
Factoring out $I_{1}$ :
$I_{1} (R_{1} + \frac{R_{2}^{2}}{R_{3}}) = E$
Step 7: Derive the Ratio of Resistances
Now, using the condition for balance (when $I = 0$ ):
$\frac{R_{1}}{R_{2}} = \frac{R_{4}}{R_{3}}$
This confirms the Wheatstone bridge principle.

Updated on:7/8/2024

|

Class 12 PHYSICS CURRENT ELECTRICITY

Topper's Solved these Questions

Similar Questions

Knowledge Check

Wheatstone bridge can be used
Ato compare two unknown resistances.
Bto measure small strains produced in hardmetal
Cas the working principle of meterbridge
Dall the above

PRADEEP-CURRENT ELECTRICITY-Problems for Practice (B)

Home
Ask Doubt
Profile