A small disc A is placed on an inclined plane forming an angle α with the horizontal and is imparted an initial velocity v0. Find how the velocity of the disc depends on the angle θ, shown in figure-2.209, if the friction coefficient μ=tanα and at the initial moment θ=π/2
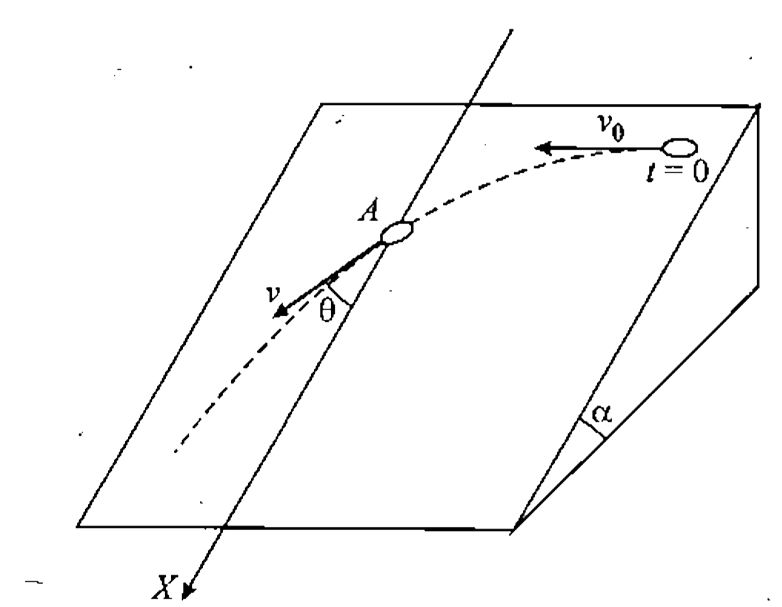
The acceleration of the disc along the plane is determined by the projection of the force of gravity on this plane Fx=mgsinα and the friction force fr=kmgcosα. In our case k=tanα and therefore
fr=Fx=mgsinα
Let us find the projection of the acceleration on the derection of the tangent to the trajectory and on the x-axis:
mwt=Fxcosφ−fr=mgsinα(cosφ−1)
mwx=Fx−frcosφ=mgsinα(1−cosφ)
It is seen from this that wt=−wx, which means that the velocity v and its projection vx differ only by a constant value C which does not change with time, i.e.
v=vx+C,
where vx=vcosφ. The constant C is found from the initial condition v=v0, where
C=v0 since φ=π2 initially. Finally we obtain
v=v0/(1+cosφ).
In the cource of time φ→0 and v→v0/2. (Motion then is unaccelerated.)

Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Laws Of Conservation Of Energy, Momentum And Angular Momentum
82 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation
34 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
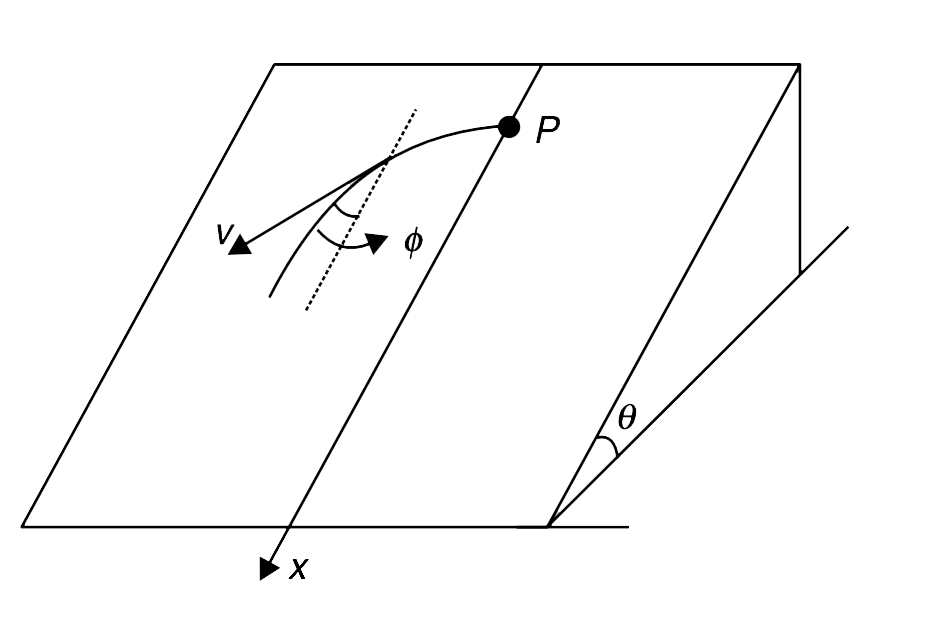
A small disc P is placed on an inclined plane forming an angle θ with the horizontal and imparted an initial velocity v0. Find how the velocity of disc depends on the angle ϕ which its velocity vector makes with the x axis (see figure). The coefficient of friction is μ=tanθ and initially ϕ0=π2
View SolutionA small body was launched up an inclined plane set at an angle α=15∘ against the horizontal. Find the coefficient of friction, if the time of the ascent of the body is η=2.0 times less than the time of its descent.
View Solution
Knowledge Check
A block is at rest on an inclined plane making an angle α with the horizontal. As the angle of the incline is increased the block start slipping when the angle of inclination becomes θ then coefficient of friction is equal to
AsinθBcosθCtanθDIndependent of θA particle is projected with a velocity u making an angle θ with the horizontal. At any instant its velocity becomes v which is perpendicular to the initial velocity u. Then v is
AucosθButanθCusecθDucotθA trolley containing a liquid slides down a smooth inclined plane of angle α with the horizontal. Find the angle of inclination θ of the free surface with the horizontal.
 Aθ=α2Bθ=2αCθ=zeroDθ=α
Aθ=α2Bθ=2αCθ=zeroDθ=α
Particle "A" is released from a point "P" on a smooth inclined plane inclined at an angle α with the horizontal.At the same instant another particle "B" is projected with initial velocity 'u' making an angle β 'with the horizontal.Both the particles meet again on the inclined plane.the relation between ' α and β is 1) α+β=π6 2) α+β=π4 3) α+β=π3 4) α+β=π2
View SolutionA small bar starts sliding down an inclined plane forming an angle α with the horizontal. The friction coefficient depends on the distance x covered as k=ax, where a is a constant. Find the distance covered by the bar till it stops, and its maximum velocity over this distance.
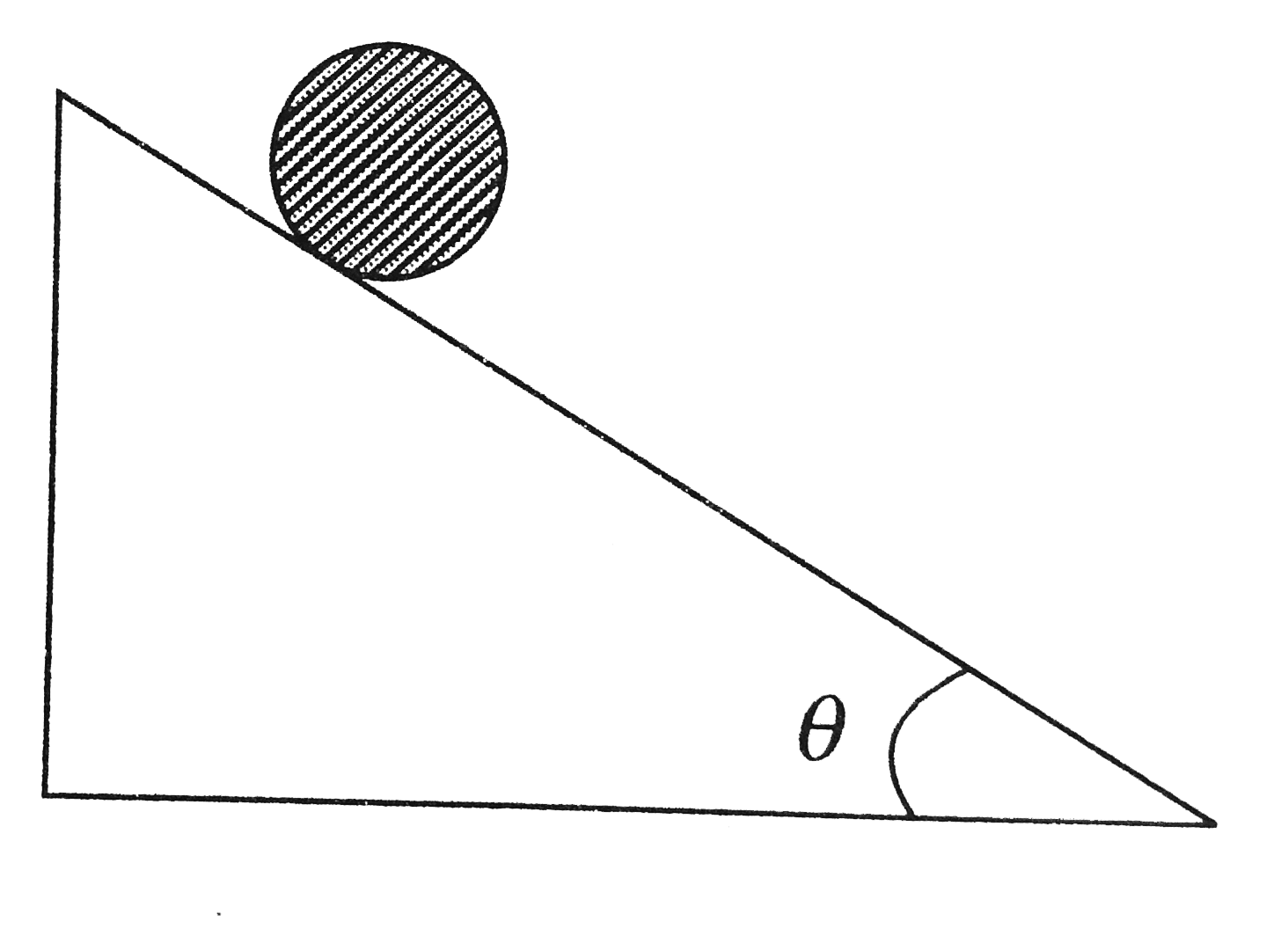
View SolutionA homogeneous ball is placed on a plane making an angle θ with the horizontal. At what values of the coefficient of friction μ the ball roll down the plane without slipping ?
 .
.
View SolutionA block is at rest on an inclined plane making an angle α with the horizontal . As the angle α of the incline is increased the block starts slipping when the angle of inclination becomes θ. The coefficient of static friction between the block and the surface of the inclined plane is or A body starts sliding down at an angle θ to the horizontal. Then the coefficient of friction is equal to
View SolutionA solid cylinder rolls up an inclined plane of inclination θ with an initial velocity v. How far does the cylinder go up the plane ?
View Solution
