A rifle was aimed at the vertical line on the target located precisely in the northern direction, and then fired. Assuming the air drag to be negligible, find how much off the line, and in what direction, will the bullet hit the target. The shot was fired in the horizontal direction at the latitude φ=60∘, the bullet velocity v=900m/s, and the distance from the target equals s=1.0km.
Define the axes as shown with z along the local vertical, x due east and y due north. (We assume we are in the northern hemisphere). Then the Coriolis force has the componets.
→Fcor=−2m(→ω×→v)
=2mω[vycosθ−vxsinθ)→i−vxcosθ→j+vxcosθ→k]=2mω(vycosθ−vxsinθ)→i
since vx is small when the direction in which the gun is fired is due north. Thus the equation of motion (neglecting centrifugal forces) are
..x=2mω(vysinφ−vxcosφ), ..y=0 and ..z=−g
Integrating we get .y=v (constant), .z=−gt
and .x=2ωvsinφt+ωgt2cosφ
Finally,
x=ωvt2sinφ+13ωgt3cosφ
Now v>>gt in the present case. so,
x≈ωvsinφ(sv)2=ωsinφs2v
≈7cm (to the east).

Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Laws Of Conservation Of Energy, Momentum And Angular Momentum
82 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Universal Gravitation
34 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
At the equator a stationary (relative to the Earth) body falls down from the height h=500m. Assuming the air drag to be negligible, find how much off the vertical, and in what direction, the body will deviate when it hits the ground.
View SolutionA bullet with muzzvelocity 100 m/s is to be shot at a target 30 m away in the horizontal line .How high above the target must the renaimed so that the bullet will hit the target?
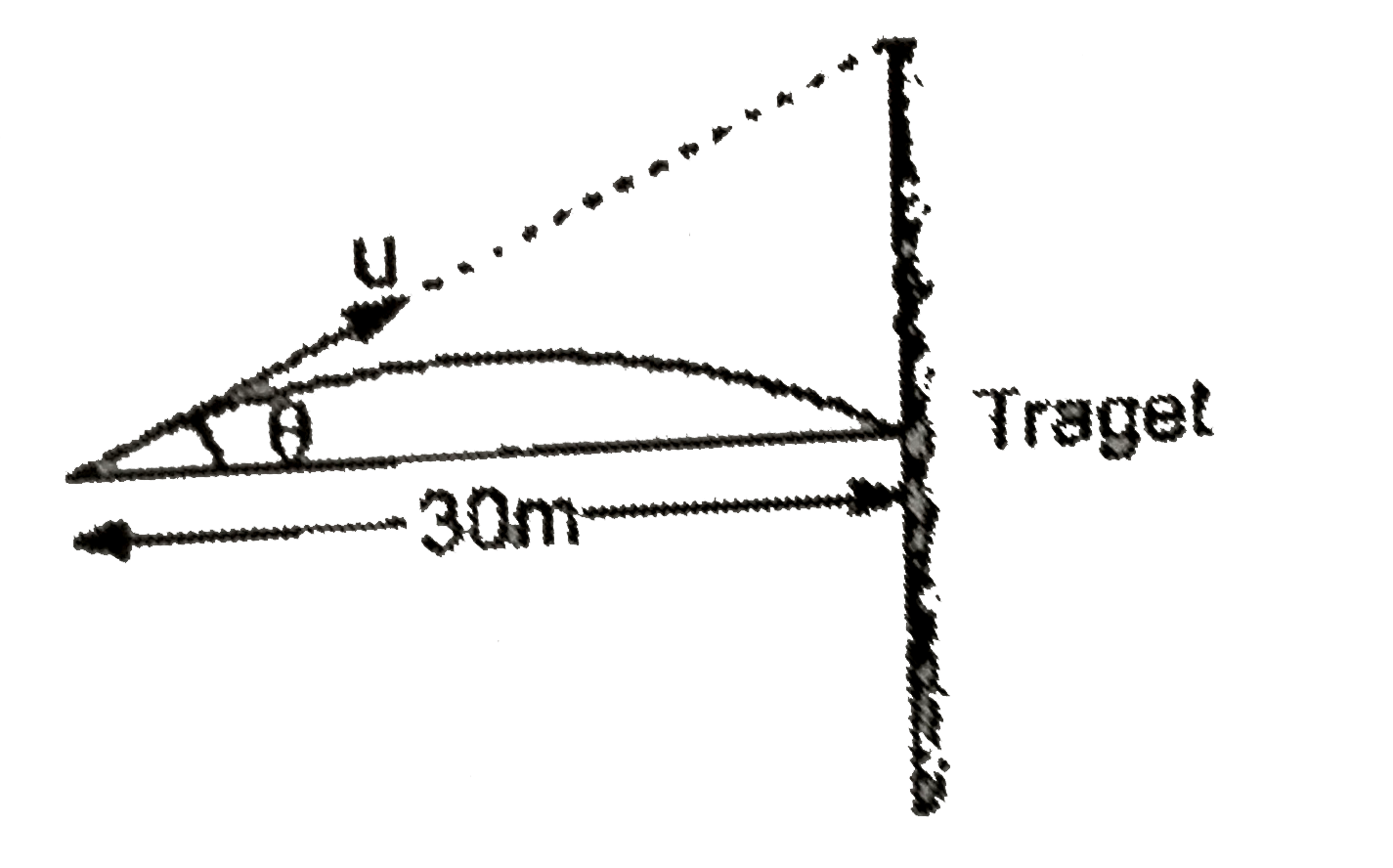
View Solution
Knowledge Check
A bullet fired from a rifle travels at an average speed of 2520 km/hr. It hits the target after 0.2 seconds. How far (in m) is the target from the rifle ?
A70B140C100D200A bullet is fired from a rifle that is held 1.6 m above the ground in a horizontal position. The initial speed of the bullet is 1100 m/s. Find the time it takes for the bullet to strike the ground and the horizontal distance traveled by the bullet.
A0.24 s, 693 mB0.57 s, 630 mC0.32 s, 440 mD0.63 s, 352 mA bullet with muzzle velocity 100ms−1 is to be shot at a target 30m away in the same horizontal line. How high above the target must the rifle be aimed so that the bullet will hit the target ?
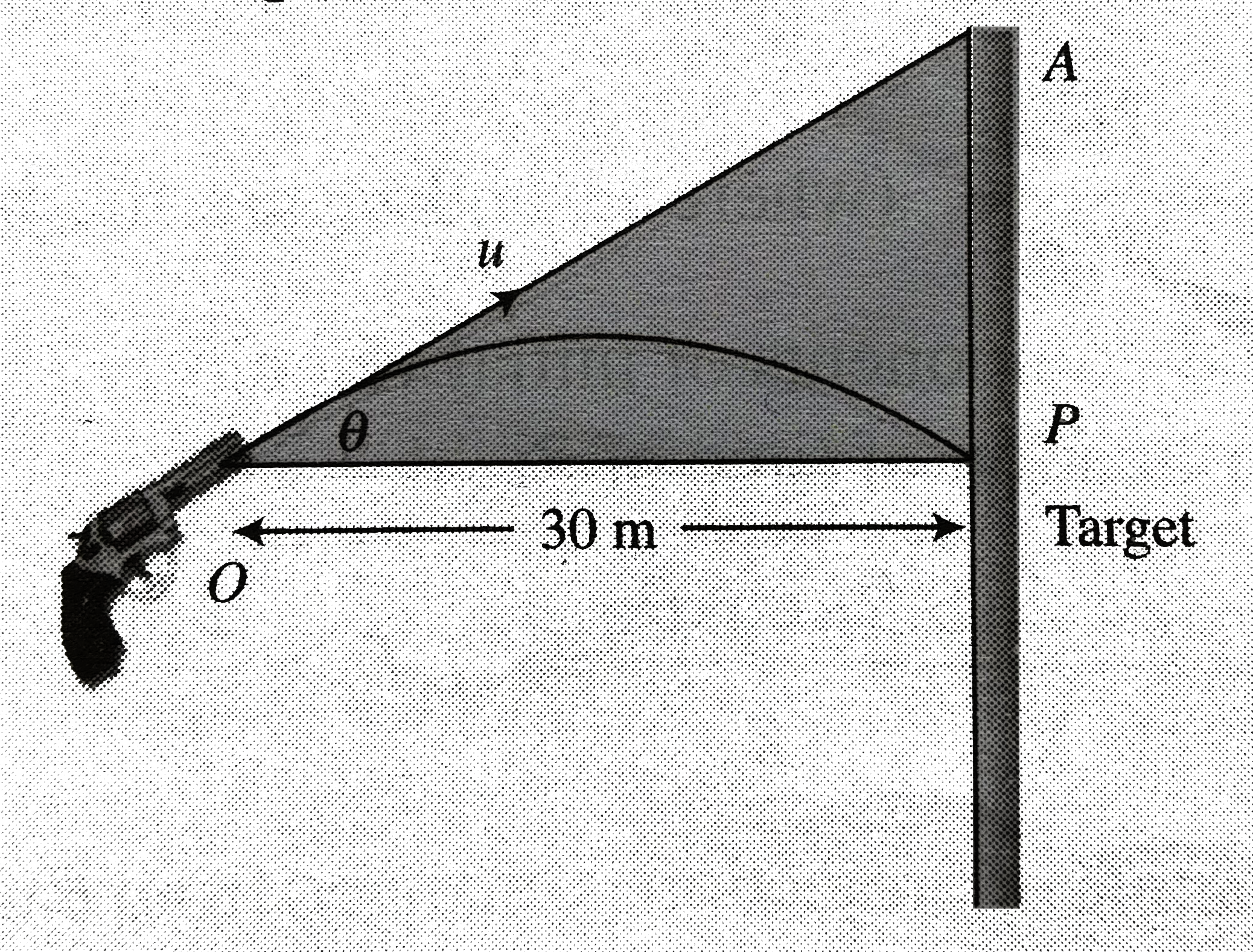 .A0B0.45cmC45cmD90cm
.A0B0.45cmC45cmD90cm
A bulley with muzzle velocity 100ms−1 is to be shot at a target 30m away in the same horizontal line. How high above the target must the rifle be aimed so that the bullet will hit the target ?
 .
.
View SolutionA projectile is fired with an initial velocity of 240 m/s at a target B located 600 m above the gun A and at a horizontal distance of 3600 m. Neglecting air resistance, determine the value of the firing angle θ .

View SolutionA rifle shoots a bullet with a muzzle velocity of 400ms−1 at a small target 400m away. The height above the target at which the bullet must be aimed to hit the target is (g=10ms−2).
View SolutionA rifle shoots a bullet with a muzzle velocity of 400ms−1 at a small target 400m away. The height above the target at which the bullet must be aimed to hit the target is (g=10ms−2).
View SolutionA bullet fired from a rifle travels at an average speed of 2520 km/hr. It hits the target after 0.2 seconds. How far (in metre) is the target from the rifle?
View Solution
