At two points of the reference frame K two events occurred separated by a time interval Δt. Demonstrate that if these events obey the cause-and-effect relationship in the frame K (e.g. a shot fired and a bullet hitting a target), they obey that relationship in any other inertial reference frame K′.
We can take the coordinates of the two events to be
A:(0,0,0,0)B:(Δt,a,0,0)
For B to be the effect and A to be cause we must have Δt>|a|c.
In the moving frame the coordinates of A and B become
A:(0,0,0,0),B:[γ(Δt−aVc2),γ(a−VΔt),0,0] where γ=1√1−(V2c2)
Since
(Δt′)2−a′2c2=γ2[(Δt−aVc2)2−1c2(a−VΔt)2)=(Δt)2−a2c2>0
we must have Δt′>|a′|c
Topper's Solved these Questions
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Hydrodynamics
25 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- THERMODYNAMICS AND MOLECULAR PHYSICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - THERMODYNAMICS AND MOLECULAR PHYSICSEXERCISE - Transport Phenomena
38 Videos
Similar Questions
At two points of an inertial reference frame separated by a distance along the x-axis of l=x2−x1 two events take place slmultaneously. Find the time interval between the events in an !arbitrary inertial reference frame.
View SolutionThe space-time diagram, shows three events A, B, and C which occurred on the x axis of some inertial reference frame. Find:
(a) the time interval between the events A and B in the reference frame where the two events occurred at the same point,
(b) the distance between the points at which the events A and C occured in the reference frame where these two events are simultaneous.
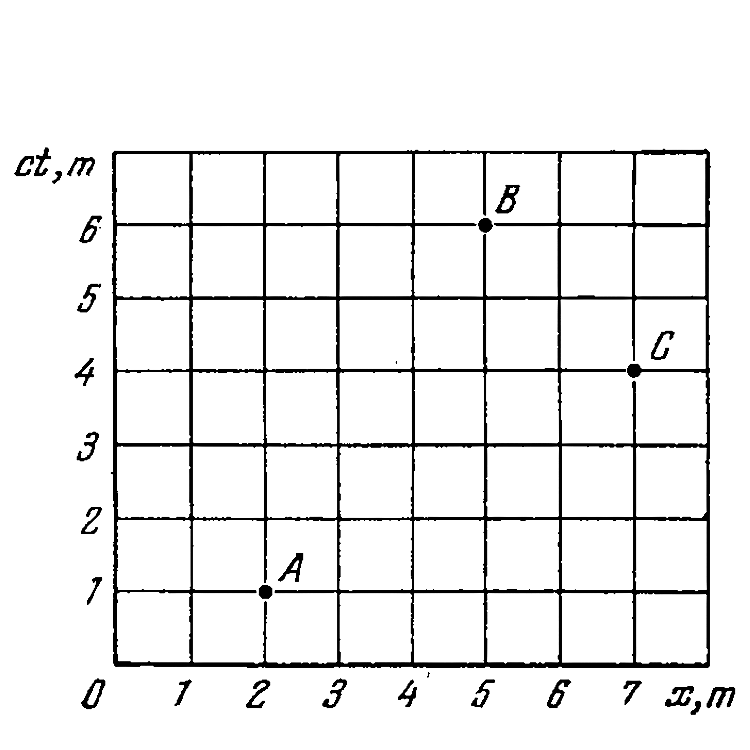
View Solution
Knowledge Check
Two events occur on the x axis separated in time by Δt and in space by Δx. A reference frame, traveling at less than the speed of light, in which the two events occur at the same time
AExists no matter what the values of Δx and ΔtBExists only if Δx/Δt<cCExists only if Δx/Δt>cDDoes not exist under any condition
The reference frame, in which the centre of inertia of a given system of particles is at rest, translates with a velocity V relative to an inertial reference frame K. The mass of the system of particles equals m, and the total energy of the system in the frame of the centre of inertia is equal to ˜E. Find the total energy E of this system of particles in the reference frame K.
View SolutionWith what velocity (relative to the reference frame K) did the clock move, if during the time interval t=5.0s, measured by the clock of the frame K, it becomes slow by Δt=0.10s?
View SolutionTwo events A and B occur at places separated by 106km, B occuring 5s after A . (a) Find the velocity of a frame in which these events occur at the same place . (b)What is the time interval between the events in this frame ?
View SolutionTwo events A and B occur at places separated by 106km, B occuring 5s after A . (a) Find the velocity of a frame in which these events occur at the same place . (b)What is the time interval between the events in this frame ?
View SolutionA point A oscillates according to a certain harmonic law in the reference frame K′ which in its turn performs harmonic oscillations relative to the reference fromeK. Both oscillations occur along the same direction. When the K′ frame oscillates at the frequency 20 or 24Hz, the beat frequency of the point A in the K frame turns out to be equal to v. At what frequency of oscillation of the frame K′ will the beat frequency of the point A become equal to 2v?
View SolutionDemonstrate that the angular momentum M of the sytem of particles relative to a point O of the reference frame K can be represented as
M=˜M+[rCp],
where ˜M is its proper angular momentum (in the reference frame moving translationally and fixed to the centre of inertia), rC is the radius vector of the centre of inertia relative to the point O, p is the total momentum of the system of particles in the reference frame K.
View SolutionA train of length l=350m starts moving rectilinearly with constant acceleration w=3.0⋅10−2m/s2, t=30s after the start the locomotive headlight is switched on (event 1), and τ=60s after that event the tail signal light is switched on (event 2). Find the distance between these events in the reference frames fixed to be train and to the Earth. How and at what constant velocity V relative to the Earth must a certain reference frame K move for the two events to occur in it at the same point?
View Solution
