The volume of one mode of an ideal gas with adiabatic exponent $γ$ is varied according to the law $V = a / T$ , where a is constant . Find the amount of heat obtained by the gas in this process, if the temperature is increased by $Δ T$ .

Video Solution

Text Solution

Verified by Experts

The differential work done by the gas
$d A = p d V = \frac{v R T^{2}}{a} (- \frac{a}{T^{2}}) d T = - v R d T$
$(a s p V = v R T and V = \frac{a}{T})$
So, $A = - \int_{T}^{T + Δ T} v R d T = - γ R Δ T$
From the first law of thermodymics
$Q = Δ U + A \frac{v R}{γ - 1} Δ T - v R Δ T$
= $v R Δ T . \frac{2 - γ}{γ - 1} = R Δ T . \frac{2 - γ}{γ - 1} (f or v = 1 mole)$ .

|

Updated on:21/07/2023

Class 12 PHYSICS THERMODYNAMICS AND MOLECULAR PHYSICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The volume of one mode of an ideal gas with adiabatic exponent $γ$ is varied according to the law $V = a / T$ , where a is constant . Find the amount of heat obtained by the gas in this process, if the temperature is increased by $Δ T$ .
A $(\frac{1 - γ}{γ + 1}) R △ T$
B $\frac{R}{γ - 1} △ T$
C $(\frac{2 - γ}{γ - 1}) R △ T$
D $\frac{R △ T}{γ - 1}$
Question 2 - Select One
The volume of one mole of ideal gas with adiabatic exponent is varied according to law $V = \frac{1}{T}$ . Find amount of heat obtained by gas in this process if gas temperature is increased by 100 K.
A $100 R$
B $200 R$
C $300 R$
D $400 R$
Question 3 - Select One
The efficiency of an ideal gas with adiabatic exponent $' γ'$ for the shown cyclic process would be

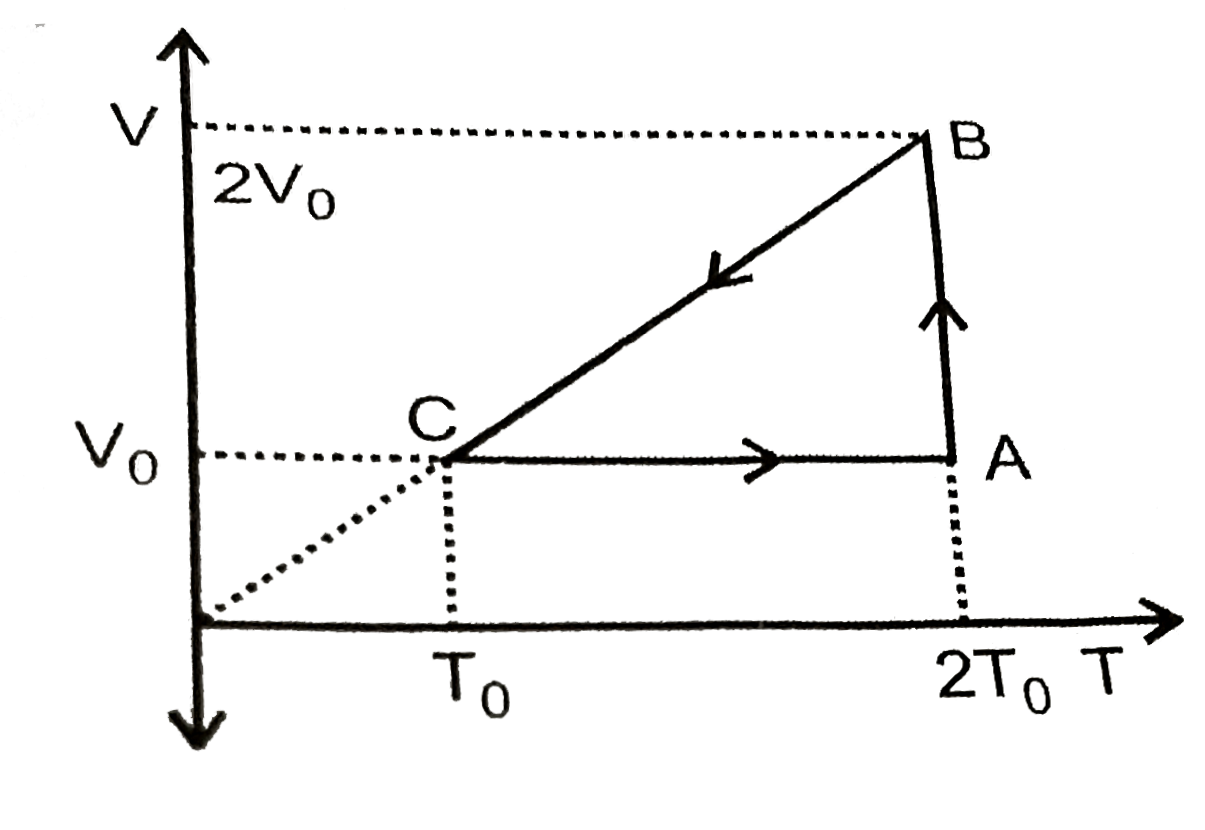A $\frac{(γ - 1) (2 ln 2 - 1)}{1 + (γ - 1) 2 ln 2}$
B $\frac{(γ - 1) (1 - 2 ln 2)}{(γ - 1) 2 ln 2 - 1}$
C $\frac{(2 ln 2 + 1) (γ - 1)}{(γ - 1) 2 ln 2 + 1}$
D $\frac{(2 ln 2 - 1)}{γ / (γ - 1)}$

IE IRODOV, LA SENA & SS KROTOV-THERMODYNAMICS AND MOLECULAR PHYSICS-The First Law Of Thermodynamics - Heat Capacity

Home
Ask Doubt
Profile