The system differs from the one examined in the foregoing problem (Fig ) by a capacitor of capacitance replacing the resistance . Find the accelearation of the connector.

From Lenz's law, the current the copper bar is directed from 1 to 2 or in other words, the induced current in the current is in clockwise sense.
Potential difference across the capacitor plates,
or,

Hence, the induced current in the loop,
But the variation of magnetic flux through the loop is caused by the movement of the bat.
So, the infuced
and,
Hence,
Now, the forces acting on the bars are the weight and the Ampere's force, where .
From Newton's second law, for the rod,
or,
Hence
Topper's Solved these Questions
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Motion Of Charged Particle In Magnetic Field36videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRODYNAMICSEXERCISE - Constant Magnetic Fiels - Magnetics69videos
ELECTRODYNAMICS
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTRICITY AND MAGNETISMEXERCISE - All Questions6videos
ELECTRICITY AND MAGNETISM
- BOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - ELECTROMAGNETISMEXERCISE - All Questions24videos
ELECTROMAGNETISM
Similar Questions
Twelve identical capacitors of capacitance C are connected across each edge of a cube. Find equivalent capacitance across one of the edges.
View SolutionTwelve identical capacitors of capacitance C are connected across each edge of a cube. Find equivalent capacitance across one of the face diagonals.
View Solution
Knowledge Check
- Question 1 - Select One
As in the figure, if a capacitor of capacitance 'C' is charged by connecting it with resistance R, then energy given by the battery will be
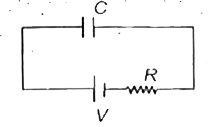 A12CV2Bless than 12CV2CCV2DMore than CV2
A12CV2Bless than 12CV2CCV2DMore than CV2 - Question 2 - Select One or More
A capacitor of capacitance C1 is charged to a potential difference V and then connected with an uncharged capacitor of capacitance C2 a resistance R. The switch is closed at t = 0. Choose the correct option(s):
AThe initial current through the resistance is VRBThe current through the resistance as a function of time is i(t)=VRe−tτ where τ=R(C1C2C1+C2)CThe charge on capacitor C1 as a function of time is q1(t)=(C1VC1+C2)(C1+C2e−tτ)DAfter a long time, the potential difference across the capacitor C1is(C1C1+C2)V - Question 3 - Select One
circuit consists of a source of EMF ξ and internal resistance r, two capacitors each of capacitance C and two resistors, each of value R. The voltage across either capacitor is:
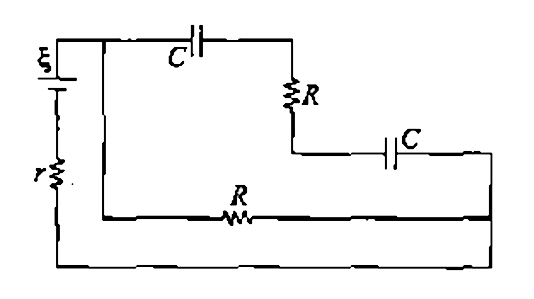 AξR2(R+r)BξR(R+r)Cξ(R+r)2RDZero
AξR2(R+r)BξR(R+r)Cξ(R+r)2RDZero
If a capacitor of capacitance C is also connected in the larger loop as shows in Fig . , find the charge on the capactior as a function of time.
 View Solution
View SolutionA capacitor of capacitance C charged by battery at V volt and then disconnected. At t = 0, it is connected to an uncharged capacitor of capacitance 2C through a resistance R. The charge on the second capacitor as a function of time is given by q=αCV3(1−e−3tβRC) then fing the value of αβ.
View SolutionA capacitor of capacitance C is given a charge go. At time t=0 it is connected to an uncharged capacitor of equal capacitance through a resistance R. Find the charge on the first capacitor and the second capacitor as a function of time t. Also plot the corresponding q-t graphs.
View SolutionA capacitor of capacitance C is charged to a potential differece V0. The terminals of the charged capacitor are then connected to those of an uncharged capacitor of capacitance C/2.
a. Compute the original charge of the system.
b. Find the final potential differce across each capacitor.
c. Find the final energy of the system.
d. Calculate the decrease in energy when the capacitors are connected.
e. Where did the ''lost'' energy go?View SolutionAn alternating current of frequency ω=314s−1 is fed to a circuit consisting of a capacitor of capacitance C=73μF and an active resistance R=100Ω connected in parallel. Find the impedance of the circuit.
View Solution

