The graph of the function y=g(x) is shown.The number of solutions of the equation ||g(x)|−1|=12, is

Video Solution
Doubtnut Promotions Banner
|
Answer

Step by step video & image solution for The graph of the function y = g (x) is shown.The number of solutions of the equation ||g(x)|-1|=1/2, is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023
Class 12MATHSDEFAULT

Similar Questions

Knowledge Check

  • The graph of the function y = g(x) is shown. The number of solutions of the equationm ||g(x)|−1|=12, is
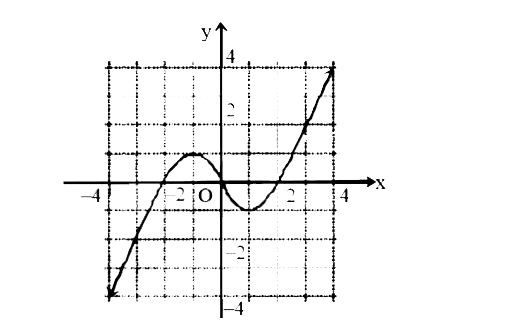    ocr_image

    A4
    B5
    C6
    D8

  • The absolute valued function f is defined as f(x)={xx≥0−xx<0} and fractional part function g(x) as g(x) = x-[x], graphically the number of real solution(s) of the equation f(x) = g(x) is obtained by finding the point(s) of interaction of the graph of y = f(x) and y = g(x).
    The number of solutions (s) of |x−1|={x},x∈[−1,1] is

    A1
    B2
    C3
    DInfinitely many

  • The absolute valued function f is defined as f(x)={xx≥0−xx<0} and fractional part function g(x) as g(x) = x-[x], graphically the number of real solution(s) of the equation f(x) = g(x) is obtained by finding the point(s) of interaction of the graph of y = f(x) and y = g(x).
    The number of solution (s) |x−1|−|x+2|=k, when −3<k<3

    A0
    B1
    C2
    D3