Two capacitors of capacitances C1andC2 are connected in parallel. If a charge q is given to the assembly, the charge gets shared. The ratio of the charge on the capacitor C1 to the charge on C2 is
C1C2
C2C1
C1C2
1C1C2
The correct Answer is:A
To solve the problem of finding the ratio of the charge on two capacitors connected in parallel, we can follow these steps:
1. Understand the Configuration:
- We have two capacitors, C1 and C2, connected in parallel. When capacitors are connected in parallel, they share the same voltage across their terminals.
2. Define the Charges:
- Let Q1 be the charge on capacitor C1 and Q2 be the charge on capacitor C2.
3. Use the Relationship of Voltage and Charge:
- The voltage across each capacitor can be expressed using the formula:
V=QC
- For capacitor C1:
V=Q1C1
- For capacitor C2:
V=Q2C2
4. Set the Voltages Equal:
- Since both capacitors are in parallel, the voltages across them are equal:
Q1C1=Q2C2
5. Rearrange the Equation:
- Cross-multiplying gives:
Q1⋅C2=Q2⋅C1
6. Find the Ratio of Charges:
- Dividing both sides by Q2⋅C1 gives:
Q1Q2=C1C2
7. Conclusion:
- Therefore, the ratio of the charge on capacitor C1 to the charge on capacitor C2 is:
Q1Q2=C1C2
Final Answer:
The ratio of the charge on capacitor C1 to the charge on capacitor C2 is C1C2.
---
- We have two capacitors, C1 and C2, connected in parallel. When capacitors are connected in parallel, they share the same voltage across their terminals.
- Let Q1 be the charge on capacitor C1 and Q2 be the charge on capacitor C2.
- The voltage across each capacitor can be expressed using the formula:
V=QC
- For capacitor C1:
V=Q1C1
- For capacitor C2:
V=Q2C2
- Since both capacitors are in parallel, the voltages across them are equal:
Q1C1=Q2C2
- Cross-multiplying gives:
Q1⋅C2=Q2⋅C1
- Dividing both sides by Q2⋅C1 gives:
Q1Q2=C1C2
- Therefore, the ratio of the charge on capacitor C1 to the charge on capacitor C2 is:
Q1Q2=C1C2
The ratio of the charge on capacitor C1 to the charge on capacitor C2 is C1C2.
Topper's Solved these Questions
- BOOK - MARVEL PUBLICATIONCHAPTER - ELECTROSTATICSEXERCISE - TEST YOUR GRASP20videos
ELECTROSTATICS
- BOOK - MARVEL PUBLICATIONCHAPTER - ELECTRONS AND PHOTONS EXERCISE - TEST YOUR GRASP - 1715videos
ELECTRONS AND PHOTONS
- BOOK - MARVEL PUBLICATIONCHAPTER - GRAVITATION EXERCISE - TEST YOUR GRASP -220videos
GRAVITATION
Similar Questions
Knowledge Check
- Question 1 - Select One
Two capacitors of capacitances C1 and C2 are connected in parallel. If a charge Q is given to the combination, the ratio of the charge on the capacitor C1 to the charge on C2 will be
AC1C2B√C1C2C√C2C1DC2C1 - Question 2 - Select One
Condsider
 .
.
The charge on capacitor C1 is.A225μCB135μCC270μCD360μC - Question 3 - Select One
Two capacitors each of 1μF capacitance are connected in parallel and are then charged by 200 V. The total energy of their charges is
A0.01 JB0.02 JC0.04 JD0.06 J
Two capacitors of capacitance C1 and C2 are connected in parallel and a charge Q is delivered to the combination. The two are then disconnected and reconnected in series. What are the new potential differences and charge on the capacitors?
View SolutionThree capacitors are connected as shwn in. Then, the charge on capacitor C1 is .
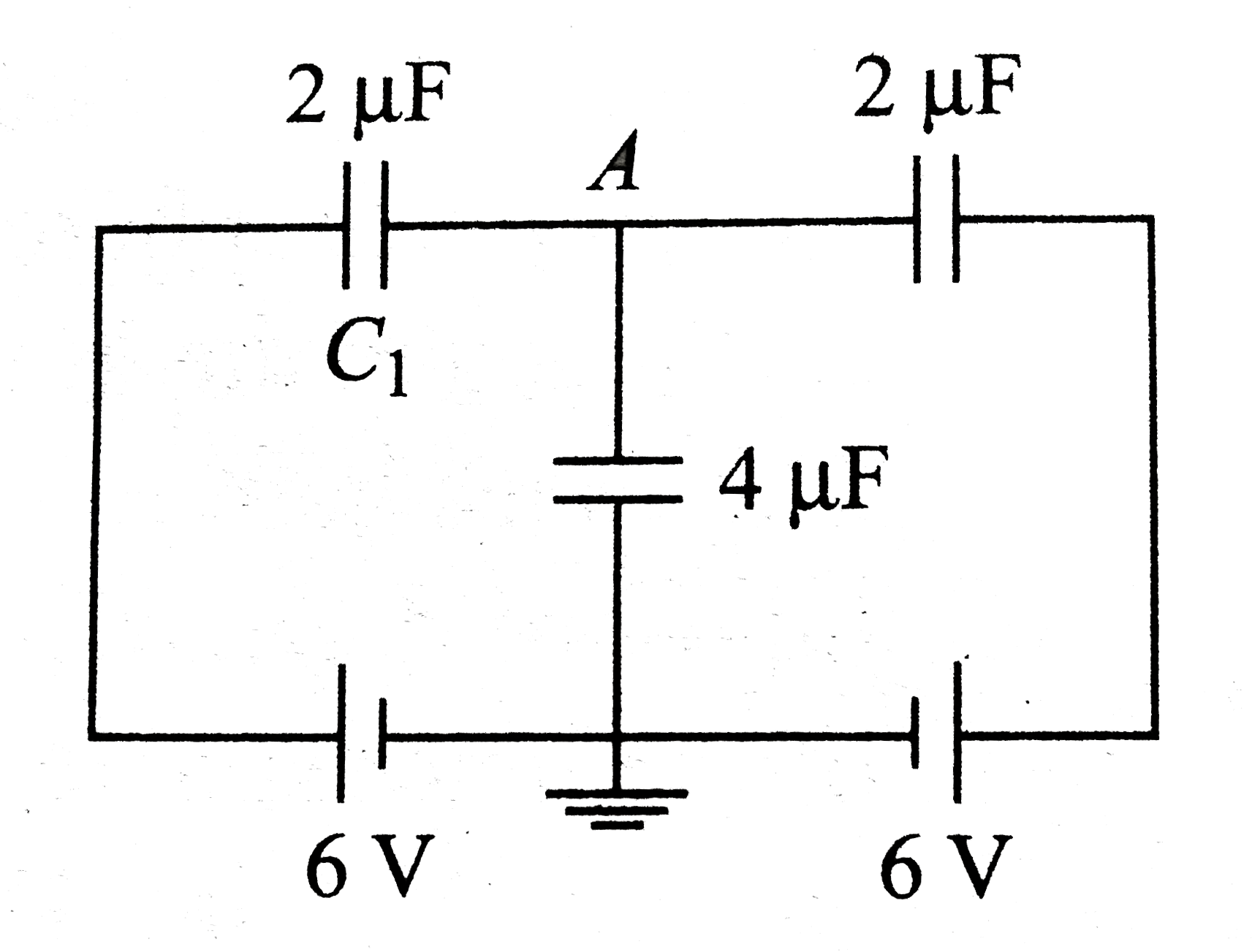 . View Solution
. View SolutionIn the question number 66, the charge on capacitors C1andC4 are
View SolutionA capacitor of capacitance C is given charge Q and then connected in parallel to a coil of inductance L. There is no resitance in the circuit. When the charge on the capacitor becomes zero, the current in the coil will be
View SolutionA capacitor of capacitance C carrying charge Q is connected to a source of emf E. Finally, the charge on capacitor would be
View Solution

