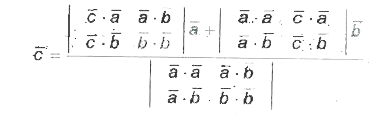
If a, b and c are three coplanar vectors. If a is not parallel to b, show that $c = \frac{∣ ∣ ∣ \begin{matrix} c \cdot a & a \cdot b c \cdot b & b \cdot b \end{matrix} ∣ ∣ ∣ a + ∣ ∣ ∣ \begin{matrix} a \cdot a & c \cdot a a \cdot b & c \cdot b \end{matrix} ∣ ∣ ∣ b}{∣ ∣ ∣ \begin{matrix} a \cdot a & a \cdot b a \cdot b & b \cdot b \end{matrix} ∣ ∣ ∣}$ .

Video Solution

Answer

Updated on:21/07/2023

If a, b and c are unit coplanar vectors, then $[2 a - b, 2 b - c, 2 c - a]$ is equal to
A1
B0
C $- \sqrt{3}$
D $\sqrt{3}$
If a, b and c are unit coplanar vectors, then $[\begin{matrix} 2 a - b & 2 b - c & 2 c - a \end{matrix}]$ is equal to
A1
B0
C $- \sqrt{3}$
D $\sqrt{3}$
If a, b and c three non-coplanar vectors, then $(a + b + c) . [a + b) \times (a + c)$ equals
A0
B $[a b c]$
C $2 [a b c]$
D $- [a b c]$

If a, b and c are three coplanar vectors. If a is not parallel to b, show that c=∣∣∣c⋅aa⋅bc⋅bb⋅b∣∣∣a+∣∣∣a⋅ac⋅aa⋅bc⋅b∣∣∣b∣∣∣a⋅aa⋅ba⋅bb⋅b∣∣∣.